Find the sum of number of faces, edges and vertices of a tetrahedron.
16
14
24
20
The correct Answer is:B
To find the sum of the number of faces, edges, and vertices of a tetrahedron, we can follow these steps:
Step 1: Identify the number of faces in a tetrahedron.
A tetrahedron is a type of pyramid with a triangular base. It has:
- 4 triangular faces (1 base triangle + 3 triangular sides).
Hint: Remember that each triangular side connects to the base triangle.
Step 2: Identify the number of edges in a tetrahedron.
Next, we count the edges. A tetrahedron has:
- 6 edges (3 edges from the base triangle + 3 edges connecting the apex to each vertex of the base).
Hint: Count the edges of the base triangle first, then add the edges connecting the apex.
Step 3: Identify the number of vertices in a tetrahedron.
Now, we count the vertices. A tetrahedron has:
- 4 vertices (3 vertices from the base triangle + 1 apex vertex).
Hint: Consider the corners of the base triangle and the top point.
Step 4: Calculate the sum of faces, edges, and vertices.
Now, we add the numbers we found:
- Number of faces = 4
- Number of edges = 6
- Number of vertices = 4
So, the sum is:
4 (faces)+6 (edges)+4 (vertices)=14
Final Answer:
The sum of the number of faces, edges, and vertices of a tetrahedron is 14.
---
A tetrahedron is a type of pyramid with a triangular base. It has:
- 4 triangular faces (1 base triangle + 3 triangular sides).
Next, we count the edges. A tetrahedron has:
- 6 edges (3 edges from the base triangle + 3 edges connecting the apex to each vertex of the base).
Now, we count the vertices. A tetrahedron has:
- 4 vertices (3 vertices from the base triangle + 1 apex vertex).
Now, we add the numbers we found:
- Number of faces = 4
- Number of edges = 6
- Number of vertices = 4
4 (faces)+6 (edges)+4 (vertices)=14
The sum of the number of faces, edges, and vertices of a tetrahedron is 14.
Topper's Solved these Questions
- BOOK - SCIENCE OLYMPIAD FOUNDATION CHAPTER - UNDERSTANDING ELEMENTARY SHAPESEXERCISE - ACHIEVERS SECTION (HOTS)5videos
UNDERSTANDING ELEMENTARY SHAPES
- BOOK - SCIENCE OLYMPIAD FOUNDATION CHAPTER - SYMMETRYEXERCISE - MATHEMATICAL REASONING15videos
SYMMETRY
- BOOK - SCIENCE OLYMPIAD FOUNDATION CHAPTER - WHOLE NUMBERSEXERCISE - Achievers section (HOTS)5videos
WHOLE NUMBERS
Similar Questions
Knowledge Check
- Question 1 - Select One
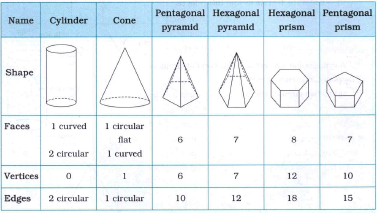
If a, b and c are respectively the number of faces, edges and vertices of a pentagonal pyramid, then the value of (a+b+c2)2 - 2 is
A−15B2C1.75D−1 - Question 2 - Select One
If a, b and c are respectively the number of faces, edges and vertices of a pentagonal pyramid, then the value of (a−b+c2)−2 is
A−15B2C1.75D−1 - Question 3 - Select One
If a, b and c are respectively the number of faces, edges and vertices of a pentagonal pyramid, then the value of (a+b+c2) - 12 is
A−15B2C175D−1
Make a conjuecture What do you think is true about the relationship between the number of vertices, edges and faces of a polyhedron ?
View SolutionIf x, y and z respectively represents the number of faces, edges and vertices of a hexagonal pyramid, then the value of (x−y+z2)2 is
View SolutionIf x,y and z respectively represents the number of faces, edges and vertices of a hexagonal pyramid, then the value of (x−y+z2)2 is
View SolutionFaces : The flat surface of any solid is called face.
Edges : Line segments where two faces meet is called an edge.
Vertices : Corners of the solid are its vertices.
Find the number of edges and vertices respectively of octahedron.View SolutionFaces : The flat surface of any solid is called face.
Edges : Line segments where two faces meet is called an edge.
Vertices : Corners of the solid are its vertices.
Find the number of faces, edges and vertices respectively of rectangular prism.View Solution