Find the value of AO) so that the function f(x) = (a+x)2sin(a+x)−a2sinax is continuous at x = 0.Solution in Telugu
a2cosa+asina
a2cosa+2asina
2a2+cosa+asina
none
The correct Answer is:B
Step by step video, text & image solution for Find the value of AO) so that the function f(x) = ((a+x)^(2)sin(a+x)-a^(2)sin a)/(x) is continuous at x = 0. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- LIMITS AND CONTINUITYBOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - LIMITS AND CONTINUITYEXERCISE - Exercise 2(Special Type Questions)
10 Videos
- LIMITS AND CONTINUITYBOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - LIMITS AND CONTINUITYEXERCISE - SET-3Match The Following
2 Videos
- LIMITS AND CONTINUITYBOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - LIMITS AND CONTINUITYEXERCISE - Exercise 1B( One Sided Limits)
71 Videos
- INVERSE TRIGONOMETRIC FUNCTIONSBOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - EXERCISE 2 (SPECIAL TYPE QUESTIONS) (SET - 4)
5 Videos
- LINEAR PROGRAMMING [APPENDIX-2]BOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - LINEAR PROGRAMMING [APPENDIX-2]EXERCISE - Exercise -1
33 Videos
Similar Questions
I. Ltx→0(1+x)2−(1−x)2−2(1+x)3−(1−x)3−3
II. If f(x)=4−7x3x+4andLtx→2f(x)=l,Ltx→0f(x)=m then the equation whose roots are 1/l, 1/m is x2−1=0
View SolutionMatch the following
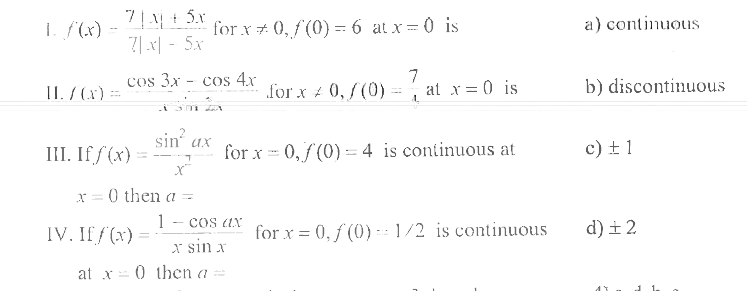
View SolutionLtx→0√x=
View SolutionA : The maximum value of f(x)=Sin−1x+Cos−1x−Tan−1x is 3π/4
R : Sin−1x+Cos−1x=π/2∀x∈R
View SolutionIf 2x+y≥10,x+2y≥10, then the lesat value of F=x+y is
View SolutionFind the value of a, so that f(x)={asin{π2(x+1)},ifx≤0,tanx−sinxx3,ifx>0is continuous at x =0
View SolutionLet the value of f(0)=k such that the function f(x)=(x sin(sin x)-sin^(2)x)/(x^(6)),x!=0 is continuous at point x=0. The value of 144k is
View SolutionIf f(x)=xsin(1)2,x≠0 then the value of the ltbRgt function at x = 0 so that the function is continuous at x= -0 , is :
View SolutionValue of f(0) so that f(x)=sin(x2+4x)−sin4xxtanxx≠0 , is continuous at x = 0 is
View SolutionIf f(x)=(a+x)2sin(a+x)−a2sinax,x≠0, then the value of f(0) so that f is continuous at x = 0 is
View SolutionThe value of f(0) so that the function f(x)=log(sec2x)xsinx,x≠0, is continuous at x = 0 is
View SolutionLet f(x)=sin(πcos2x)x2,x≠0 . The value of f(0) so that f is a continuous function is
View SolutionFind the value of f(0) so that the function f(x)=181−cos2x+sin2x√x2+1−1,x≠0 is continuous .
View SolutionFind the values of 'a' so that the function f(x) defined by f(x)={sin2axx2, x≠0 1, x=0 may be continuous at x=0 .
View SolutionIf f(x) = ((a+x)^(2)sin(a+x)-a^(2)sin a)/(x) , x !=0 , then the value of f(0) so that f is continuous at x = 0 is
View SolutionThe value of f(0) so that the function f(x) = (log(sec^2 x))/(x sin x), x != 0 , is continuous at x = 0 is
View Solution
