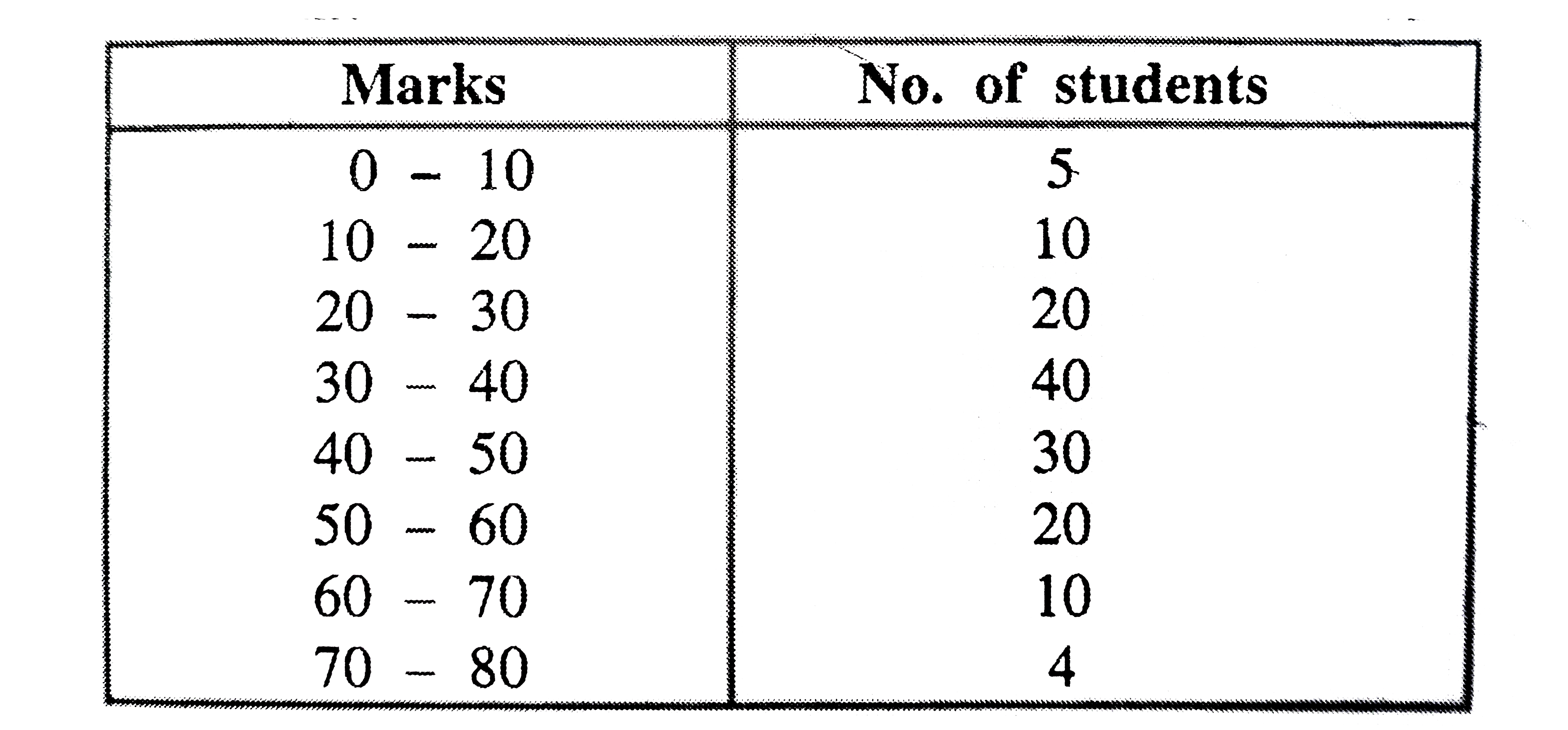
For a given distribution of marks mean is 35.16 and its standard deviation is 19.76. The coefficient of variation is :

A

$\frac{19.76}{35.16} \times 10$

B

$\frac{35.16}{19.76} \times 100$

C

$\frac{19.76}{35.16}$

D

None of these

Video Solution

Text Solution

Generated By DoubtnutGPT

The correct Answer is:D

To find the coefficient of variation (CV) for the given distribution of marks, we can follow these steps:
Step 1: Understand the formula for Coefficient of Variation
The coefficient of variation (CV) is calculated using the formula:
$C V = (\frac{Standard Deviation}{Mean}) \times 100$
Step 2: Identify the given values
From the problem, we have:
- Mean (μ) = 35.16
- Standard Deviation (σ) = 19.76
Step 3: Substitute the values into the formula
Now, we will substitute the given values into the CV formula:
$C V = (\frac{19.76}{35.16}) \times 100$
Step 4: Calculate the fraction
First, calculate the fraction:
$\frac{19.76}{35.16} \approx 0.561$
Step 5: Multiply by 100
Now, multiply the result by 100 to get the coefficient of variation:
$C V \approx 0.561 \times 100 \approx 56.1$
Final Answer
Thus, the coefficient of variation is approximately 56.1%.
---

|

Updated on:18/9/2024

Class 11 MATHS STATISTICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The standard deviation $(σ)$ of variate x is the square root of the A.M. of the squares of all deviations of x from the A.M. observations.
If $x_{i} / f_{i}$ , i=1,2,… n is a frequency distribution then $σ = \sqrt{\frac{1}{N} Σ_{i = 1}^{n} f_{i} {(x_{i} - ¯ x)}_{i}^{2}}, N = Σ_{i = 1}^{n} f_{i}$ and variance is the square of standard deviation. Coefficient of dispersion is $\frac{σ}{x}$ and coefficient of variation is $\frac{σ}{x} \times 100$
For a given distribution of marks mean is 35.16 and its standard deviation is 19.76 then coefficient of variation is
A1.778
B0.562
C177.8
D56.2
Question 2 - Select One
For a given distribution of marks, the mean is 35.16 and its standard deviation is 19.76. The coefficient of variation is
A $\frac{35.16}{19.76}$
B $\frac{19.76}{35.16}$
C $\frac{35.16}{19.76} \times 100$
D $\frac{19.76}{35.16} \times 100$
Question 3 - Select One
The Binomial distribution whose mean is 3 and whose standard deviation is 3/2 is
A ${(1 / 2 + 1 / 2)}^{12}$
B ${(1 / 4 + 3 / 4)}^{12}$
C ${(3 / 4 + 1 / 4)}^{12}$
Dnone

MODERN PUBLICATION-STATISTICS-Objective A(Multiple Choice Questions)

Home
Ask Doubt
Profile