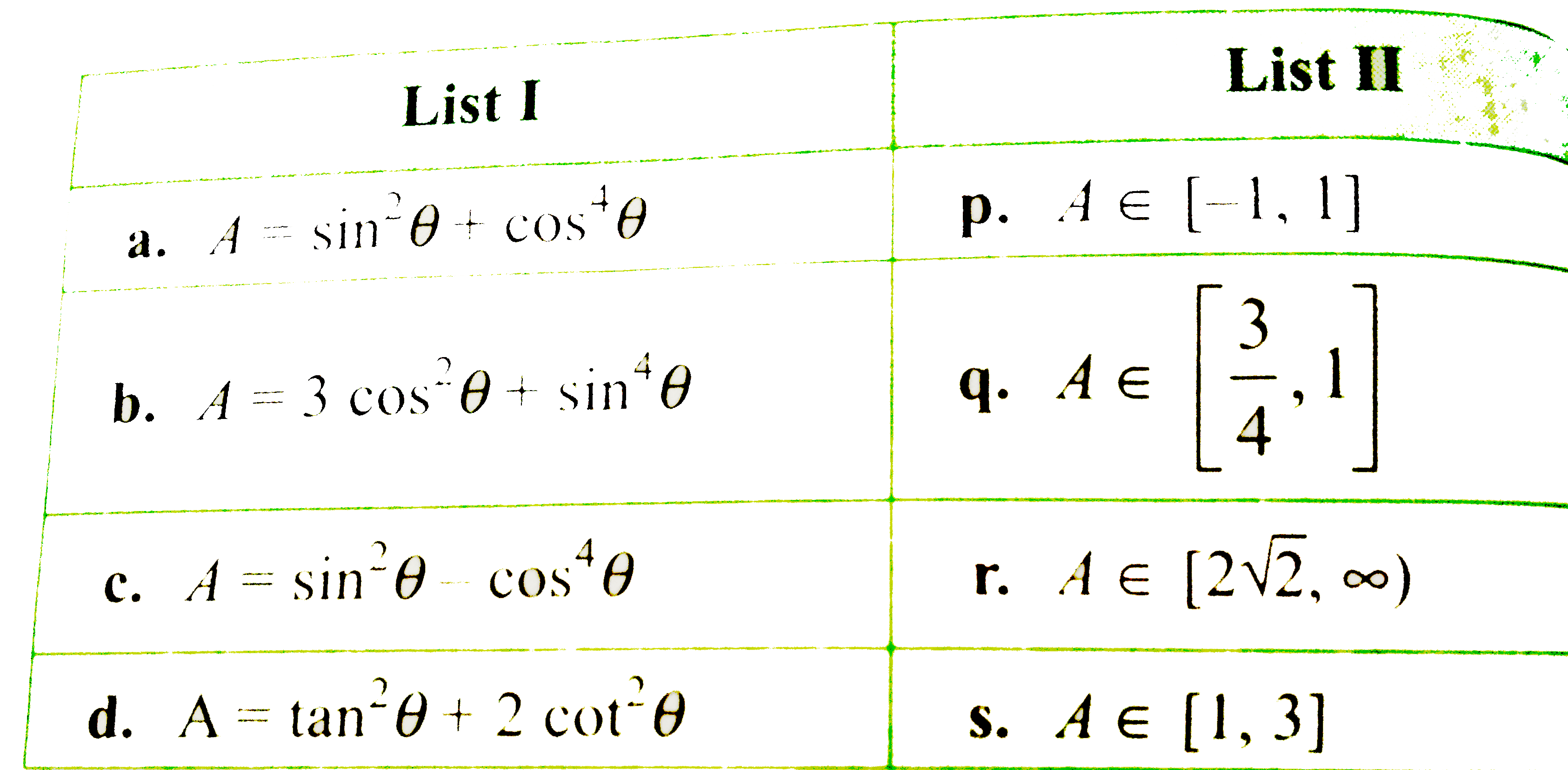
For all real values of θ, choose the correct options.
Video Solution
Text Solution
The correct Answer is:4
a. A=sin2θ+cos4θ
=1−cos2θ+cos4θ
=(cos2θ−1/2)2+3/4
0≤cos2θ≤1
⇒−1/2≤cos2θ−1/2≤1/2
⇒0≤(cos2θ−1/2)2≤1/4
⇒0≤(cos2θ−1/2)2≤1/4
⇒3/4≤(cos2θ−1/2)2+3/4≤1
b. 3cos2θ+sin4θ=3−3sin2θ+sin4θ
=(sin2θ−3/2)2+3/4
0≤sin2θ≤1
⇒−3/2≤sin2θ−3/2≤−1/2
⇒1/4≤(sin2θ−3/2)2+3/4≤3
⇒1≤(sin2θ−3/2)2+3/4≤3
c. A=sin2θ−cos4θ
=1−cos2θ−cos4θ
=5/4−(cos2θ+1/2)2
0≤cos2θ≤1
⇒1/2≤cos2θ+1/2≤3/2
⇒1/4≤(cos2+1/2)2≤9/4
⇒−9/4≤−(cos2θ+1/2)2≤−1/4
⇒−1≤5/4−(cos2θ+1/2)2≤1
d. A=tan2θ+2cot2θ
=(tanθ−√2cotθ)2+2√2≥2√2
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Numerical Value Type11videos
TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Archives JEE Main2videos
TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Linked Comprehension Type11videos
TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Archives (Matrix Match Type)1videos
TRIGONOMETRIC EQUATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASEXERCISE - Matrix Match Type1videos
TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULAS
Similar Questions
Knowledge Check
- Question 1 - Select One
Choose the correct option as directed.
ACsH>KH>NaH>LiH (Order of stability)BH2O<NH3<CH4 (Order of dipole moment)CPH3<AsH3<NH3<SbH3 (Order of boiling point)DX−−−H−X,X=O>F>N>S>Cl (Order of strength of H-bonding)
Choose the correct option w.r.t RNA.
View Solution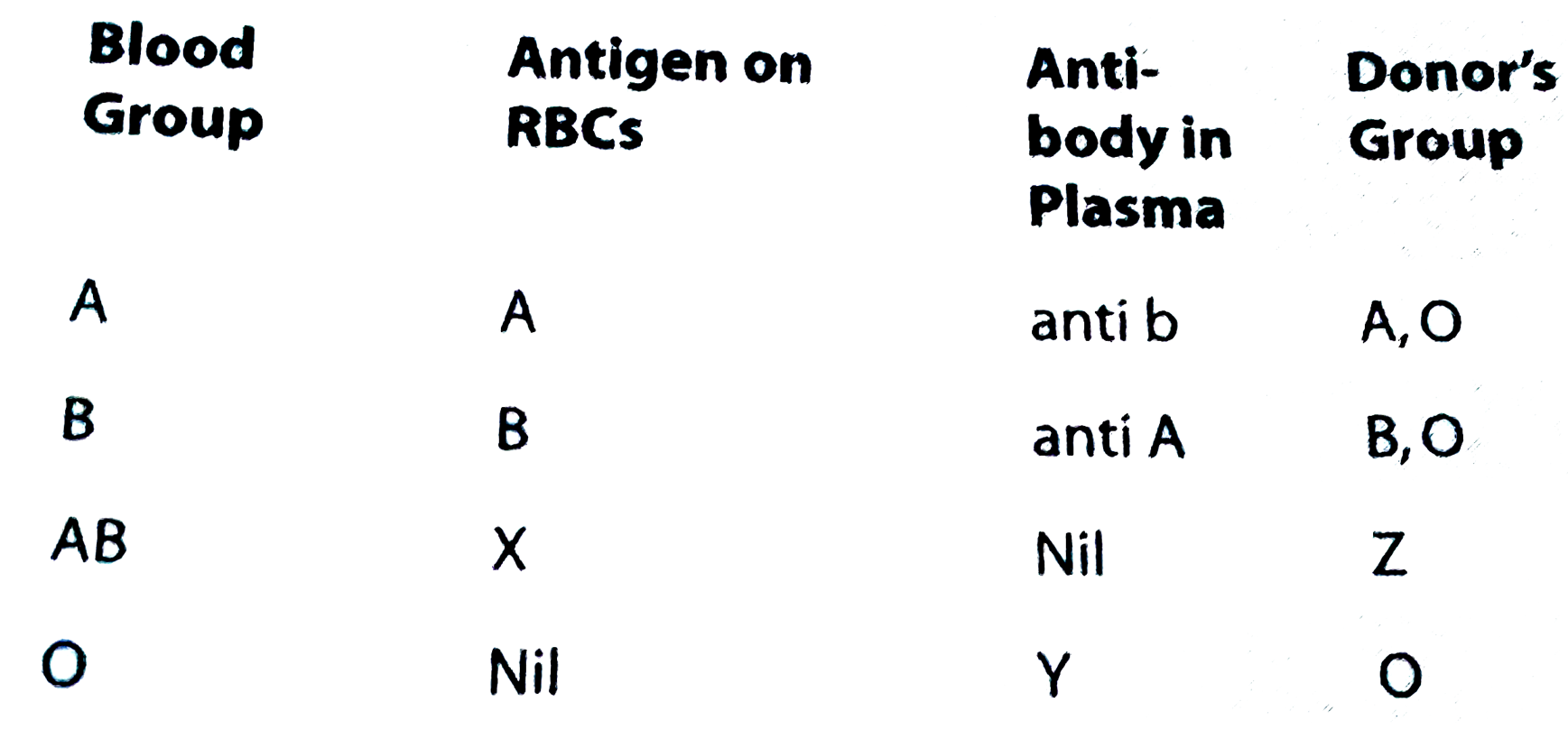
Choose the correct option for X, Y and Z.View SolutionChoose the correct option for major product
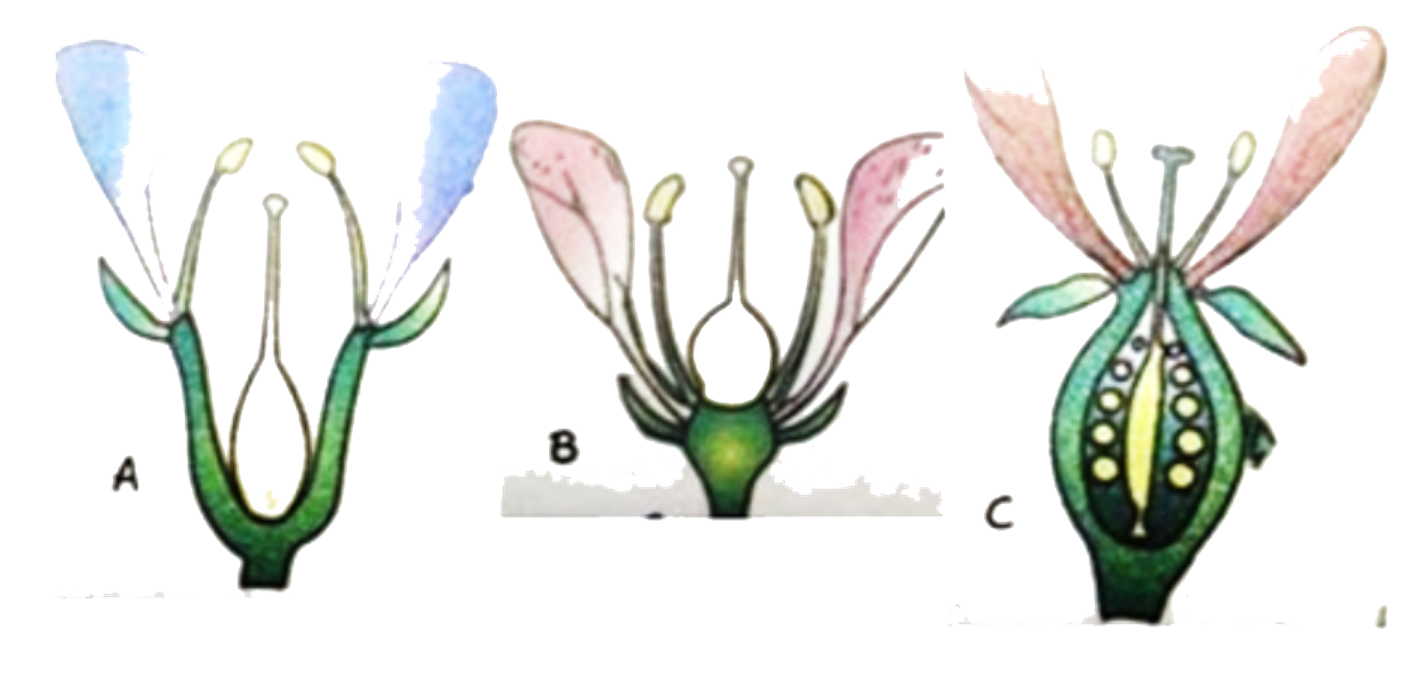
View SolutionObserve figures and choose the correct option.
 View Solution
View SolutionMatch the columns and choose the correct option
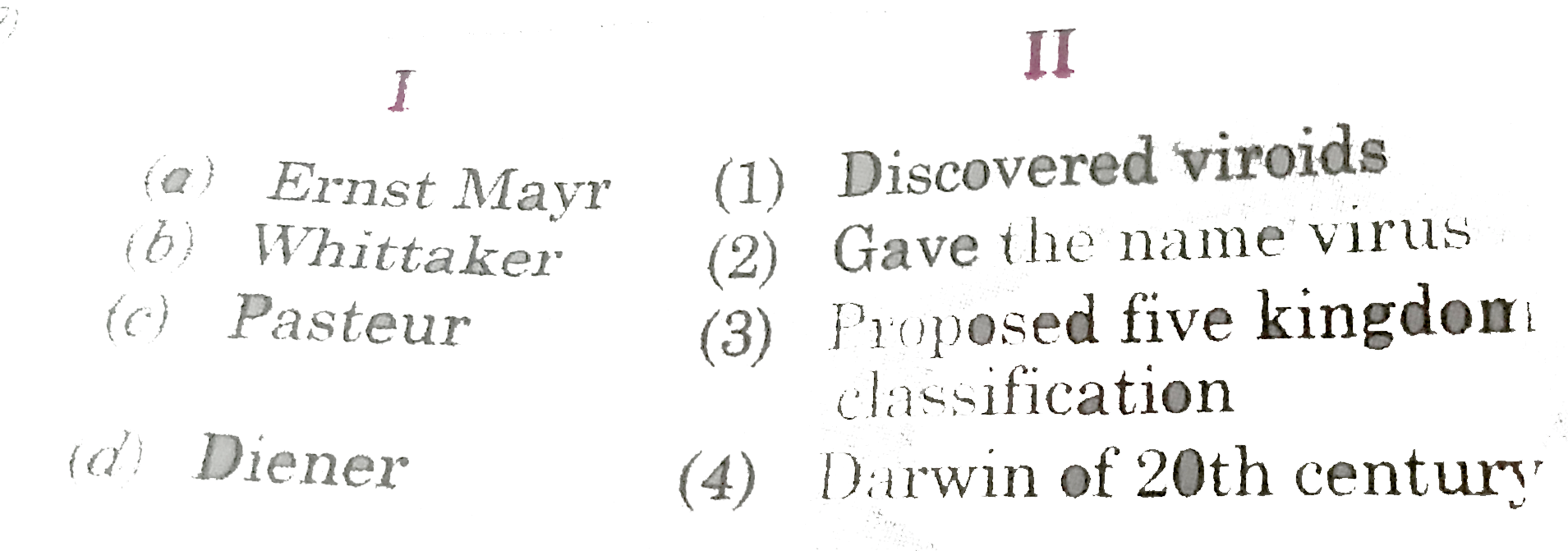 View Solution
View SolutionMatch the columns and choose the correct option
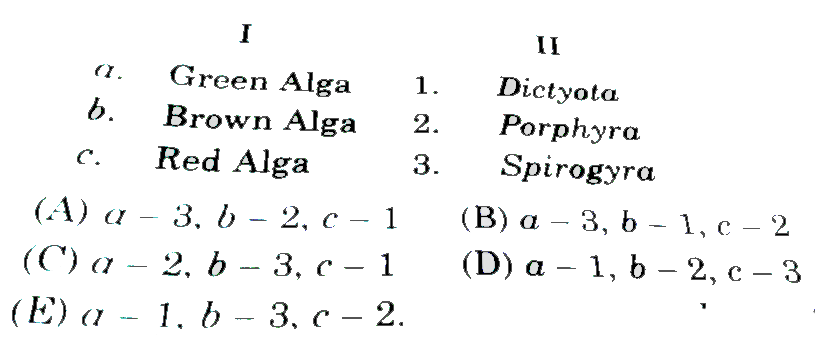 View Solution
View SolutionMatch the columns and choose the correct option
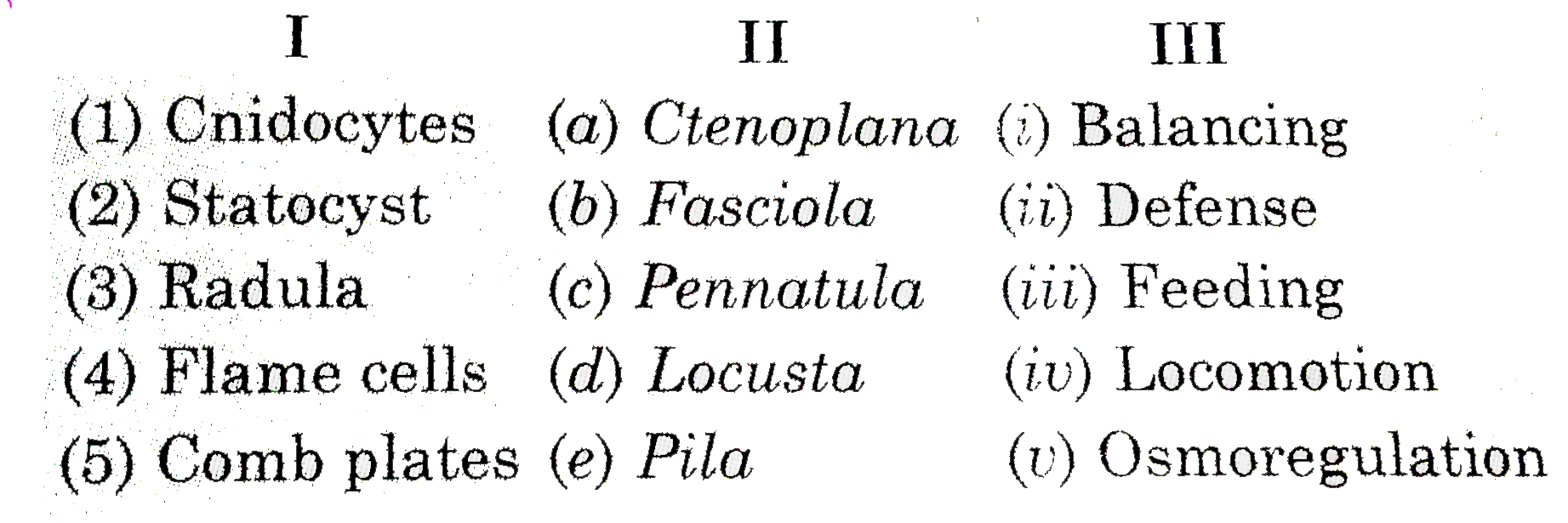 View Solution
View Solution

