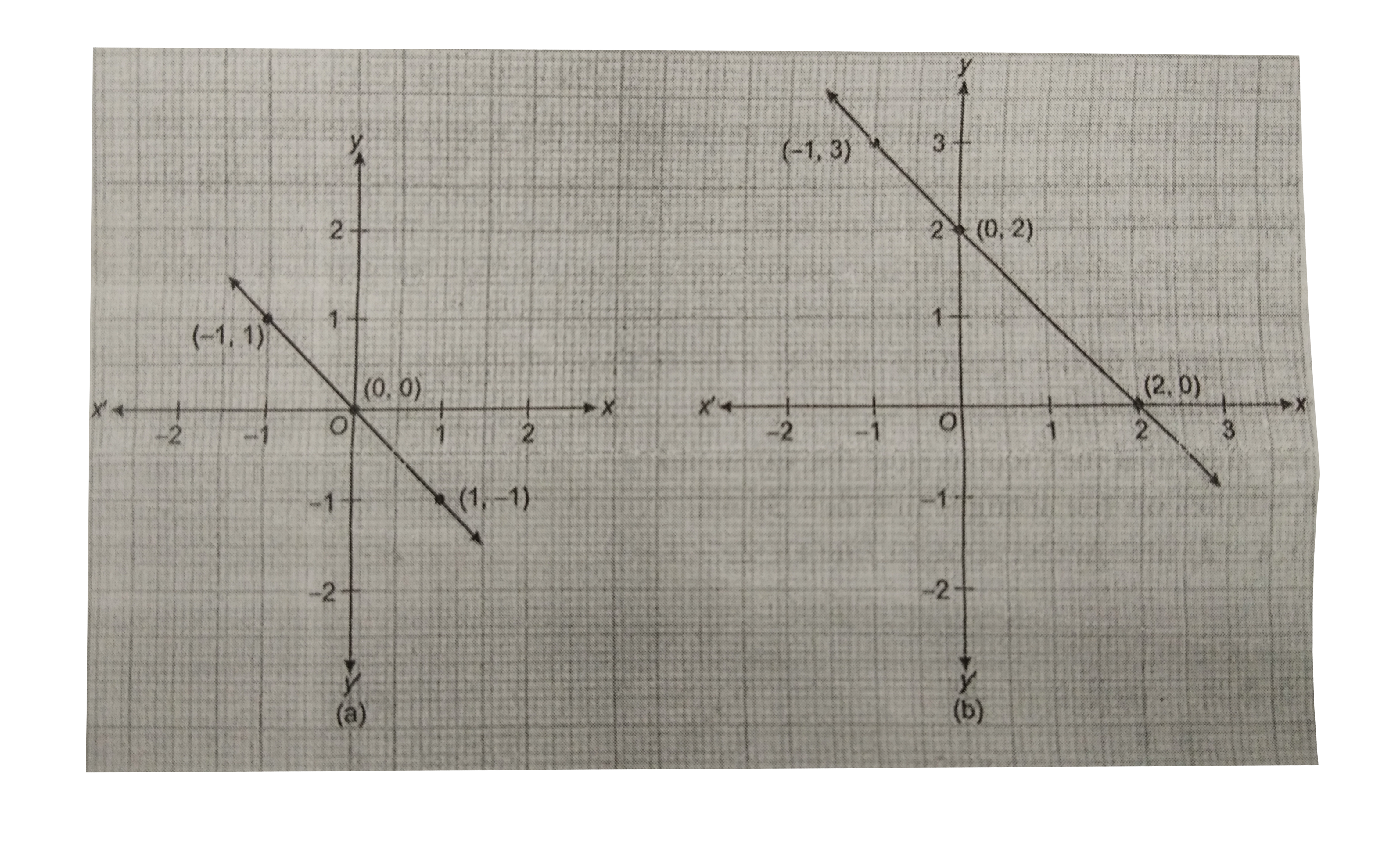
For each of the graphs given in Fig. 4.5 select the equation whose graph it is from the choices given below:(a) For Fig 4.5(i)(i) x+y=0 (ii) y=2x (iii) y=x (iv) y=2x+1(b) For fig 4,5(ii)(i) x+y=0 (ii) y = 2x (iii) y = 2x + 4 (iv) y = x - 4
To solve the problem of identifying the equations corresponding to the graphs in Fig. 4.5, we will analyze each graph and check which equation satisfies the points on the graph.
Part (a): For Fig 4.5(i)
Equations to consider:
1. x+y=0
2. y=2x
3. y=x
4. y=2x+1
Step 1: Check the equation x+y=0
- Test point (0, 0):
0+0=0(satisfied)
- Test point (1, 2):
1+2=3(not satisfied)
- Test point (-1, -2):
−1+(−2)=−3(not satisfied)
Conclusion: This equation does not satisfy all points.
Step 2: Check the equation y=2x
- Test point (0, 0):
0=2(0)(satisfied)
- Test point (1, 2):
2=2(1)(satisfied)
- Test point (-1, -2):
−2=2(−1)(satisfied)
Conclusion: This equation satisfies all points.
Step 3: Check the equation y=x
- Test point (0, 0):
0=0(satisfied)
- Test point (1, 2):
2=1(not satisfied)
- Test point (-1, -2):
−2=−1(not satisfied)
Conclusion: This equation does not satisfy all points.
Step 4: Check the equation y=2x+1
- Test point (0, 0):
0=2(0)+1(not satisfied)
- Test point (1, 2):
2=2(1)+1(not satisfied)
- Test point (-1, -2):
−2=2(−1)+1(not satisfied)
Conclusion: This equation does not satisfy all points.
Final Answer for Part (a):
The equation corresponding to Fig 4.5(i) is y=2x.
---
Part (b): For Fig 4.5(ii)
Equations to consider:
1. x+y=0
2. y=2x
3. y=2x+4
4. y=x−4
Step 1: Check the equation x+y=0
- Test point (0, 4):
0+4=4(not satisfied)
- Test point (1, 6):
1+6=7(not satisfied)
- Test point (-2, 0):
−2+0=−2(not satisfied)
Conclusion: This equation does not satisfy all points.
Step 2: Check the equation y=2x
- Test point (0, 4):
4=2(0)(not satisfied)
- Test point (1, 6):
6=2(1)(not satisfied)
- Test point (-2, 0):
0=2(−2)(not satisfied)
Conclusion: This equation does not satisfy all points.
Step 3: Check the equation y=2x+4
- Test point (0, 4):
4=2(0)+4(satisfied)
- Test point (1, 6):
6=2(1)+4(satisfied)
- Test point (-2, 0):
0=2(−2)+4(satisfied)
Conclusion: This equation satisfies all points.
Step 4: Check the equation y=x−4
- Test point (0, 4):
4=0−4(not satisfied)
- Test point (1, 6):
6=1−4(not satisfied)
- Test point (-2, 0):
0=−2−4(not satisfied)
Conclusion: This equation does not satisfy all points.
Final Answer for Part (b):
The equation corresponding to Fig 4.5(ii) is y=2x+4.
---
Summary of Answers:
- (a) y=2x
- (b) y=2x+4
1. x+y=0
2. y=2x
3. y=x
4. y=2x+1
- Test point (0, 0):
0+0=0(satisfied)
- Test point (1, 2):
1+2=3(not satisfied)
- Test point (-1, -2):
−1+(−2)=−3(not satisfied)
- Test point (0, 0):
0=2(0)(satisfied)
- Test point (1, 2):
2=2(1)(satisfied)
- Test point (-1, -2):
−2=2(−1)(satisfied)
- Test point (0, 0):
0=0(satisfied)
- Test point (1, 2):
2=1(not satisfied)
- Test point (-1, -2):
−2=−1(not satisfied)
- Test point (0, 0):
0=2(0)+1(not satisfied)
- Test point (1, 2):
2=2(1)+1(not satisfied)
- Test point (-1, -2):
−2=2(−1)+1(not satisfied)
The equation corresponding to Fig 4.5(i) is y=2x.
1. x+y=0
2. y=2x
3. y=2x+4
4. y=x−4
- Test point (0, 4):
0+4=4(not satisfied)
- Test point (1, 6):
1+6=7(not satisfied)
- Test point (-2, 0):
−2+0=−2(not satisfied)
- Test point (0, 4):
4=2(0)(not satisfied)
- Test point (1, 6):
6=2(1)(not satisfied)
- Test point (-2, 0):
0=2(−2)(not satisfied)
- Test point (0, 4):
4=2(0)+4(satisfied)
- Test point (1, 6):
6=2(1)+4(satisfied)
- Test point (-2, 0):
0=2(−2)+4(satisfied)
- Test point (0, 4):
4=0−4(not satisfied)
- Test point (1, 6):
6=1−4(not satisfied)
- Test point (-2, 0):
0=−2−4(not satisfied)
The equation corresponding to Fig 4.5(ii) is y=2x+4.
- (a) y=2x
- (b) y=2x+4
Topper's Solved these Questions
- LINEAR EQUATIONS IN TWO VARIABLESBOOK - NCERT ENGLISHCHAPTER - LINEAR EQUATIONS IN TWO VARIABLESEXERCISE - EXERCISE 4.4
2 Videos
- LINEAR EQUATIONS IN TWO VARIABLESBOOK - NCERT ENGLISHCHAPTER - LINEAR EQUATIONS IN TWO VARIABLESEXERCISE - Exercise 4.1
2 Videos
- LINEAR EQUATIONS IN TWO VARIABLESBOOK - NCERT ENGLISHCHAPTER - LINEAR EQUATIONS IN TWO VARIABLESEXERCISE - EXERCISE 4.2
4 Videos
- INTRODUCTION TO EUCLIDS GEOMETRYBOOK - NCERT ENGLISHCHAPTER - INTRODUCTION TO EUCLIDS GEOMETRYEXERCISE - Exercise 5.1
7 Videos
- LINES AND ANGLESBOOK - NCERT ENGLISHCHAPTER - LINES AND ANGLESEXERCISE - Exercise 6.1
6 Videos
Similar Questions
From the choices given below choose the equation whose graphs are given in fig (a) and fig(b)
For fig (a)
(i) y=x (ii) x+y=0
(iii) y=2x (iv) 2+3y=7x
For fig (b)
(i) y=x+2 (ii) y=x−2
(iii) y=−x+2 (iv) x+2
View SolutionDraw the graph of each of the following linear equations in two variables:(i) x+y=4 (ii) x−y=2 (iii) y=3x (iv) 3=2x+y
View Solution
Knowledge Check
The equation of the directrix of the parabola x2−4x−8y+12=0 is
Ay=0Bx−1=0Cy+1=0Dx+1=0
Draw the graph of each of the following linear equations in two variables:
(i) x+y=4 (ii) x−y=2
(iii) y=3x (iv) 3=2x+y
View SolutionDraw the graph for each equation given below:
(i) x=4,(ii) x-6=0,(iii) x+3=0,(iv)y=2 ,(v) 2x-5=0, (vi) y+3=0
View SolutionDraw the graph of each of the following given equations:
(i) y=3x, (ii) x=2y , (iii) x+3y=0 ,(iv)2x+3y=0
View SolutionFind the degree of each of the polynomials given below:(i) x5−x4+3 (ii) 2−y2−y3+2y8 (iii) 2
View SolutionDetermine graphically the vertices of the triangle, the equations of whose sides are given below: (i) 2y−x=8, 5y−x=14 and y−2x=1 (ii) y=x, y=0 and 3x+3y=10
View SolutionWrite each of the following as an equation in two variables:(i) x=−5 (ii) y=2 (iii) 2x=3 (iv) 5y=2
View SolutionFind the intercepts cuts on X-axis and Y -axis from the following lines :
(i)3x+4y=12 (ii)2x−5y=8
(iii)x+2y+3=0 (iv)2x−y+3=0
View Solution