For the given data, the calculation corresponding to all values of varsities (x, y) is following:
Σ(x−¯x)2=36,Σ(y−¯y)2=25,Σ(x−¯x),(y−¯y)=20
Karl Pearson's correlation coefficient is
0.66
−0.66
−0.5
1.66
The correct Answer is:a
To calculate Karl Pearson's correlation coefficient (R) using the provided data, we will follow these steps:
Step 1: Understand the given data
We have the following values:
- Σ(x−¯x)2=36
- Σ(y−¯y)2=25
- Σ(x−¯x)(y−¯y)=20
Step 2: Identify the formula for Karl Pearson's correlation coefficient
The formula for Karl Pearson's correlation coefficient (R) is given by:
R=Σ(x−¯x)(y−¯y)√Σ(x−¯x)2⋅Σ(y−¯y)2
Step 3: Substitute the known values into the formula
From the given data:
- Σ(x−¯x)(y−¯y)=20
- Σ(x−¯x)2=36
- Σ(y−¯y)2=25
Now substituting these values into the formula:
R=20√36⋅25
Step 4: Calculate the denominator
First, calculate 36⋅25:
36⋅25=900
Now, take the square root:
√900=30
Step 5: Complete the calculation for R
Now substitute back into the equation for R:
R=2030=23
Step 6: Convert to decimal form
To express 23 in decimal form:
R≈0.66
Final Answer
Thus, Karl Pearson's correlation coefficient is:
R≈0.66
---
We have the following values:
- Σ(x−¯x)2=36
- Σ(y−¯y)2=25
- Σ(x−¯x)(y−¯y)=20
The formula for Karl Pearson's correlation coefficient (R) is given by:
From the given data:
- Σ(x−¯x)(y−¯y)=20
- Σ(x−¯x)2=36
- Σ(y−¯y)2=25
First, calculate 36⋅25:
Now substitute back into the equation for R:
To express 23 in decimal form:
Thus, Karl Pearson's correlation coefficient is:
Topper's Solved these Questions
Similar Questions
For the given data the calculation corresponding to all values of series (x,y) is as following ∑(x−¯x)2=35,∑(y−¯y)2=25,∑(x−¯x)(y−¯y)=20
Find Karl Pearson's correlation coefficient.
View SolutionFrom the following data find regression equation of y on x, Σx=55,Σy=88,Σx2=385,Σy2=1114,Σxy=586,n=10
View Solution
Knowledge Check
If cov(X,Y)=16.5,Var(X)=8.25 and Var(Y)=33, then Karl Pearson's coefficient of correlation between X and Y is
A12B23C34D1
Find the Karl Pearson's co-efficient of correlation between x and y for the following data :
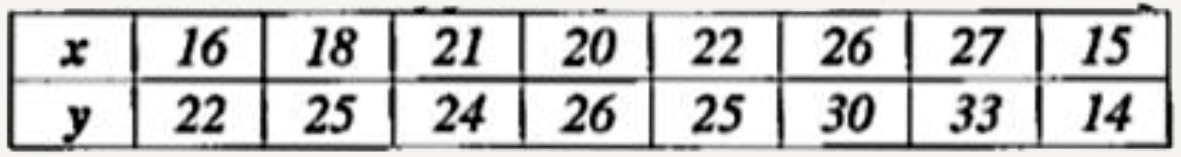
View SolutionGiven, n=5,Σxi=25,Σyi=20,Σxiyi=90 and Σx2i=135, find the regression coefficient of y on x.
View SolutionFor a bivariate data, you are given following information
Σ(xi−58)=46,Σ(xi−58)2=3086,Σ(yi−58)=9,Σ(yi−58)2=483,Σ(xi−58)(yi−58)=1095,n=7
Find (i) two lines of regression (ii) coefficient of correlation between x and y.
View SolutionFind correlation coefficient when, Σxi=40,Σyi=55,Σx2i=192,Σy2i=385,Σ(xi+yi)2=947 and n = 10.
View SolutionFind r(x,y) if cov (x,y) =-16.5, var(x)=2.25 and σy=12
View SolutionFind the Karl Pearson's coefficient of correlation between X and Y for the following data :
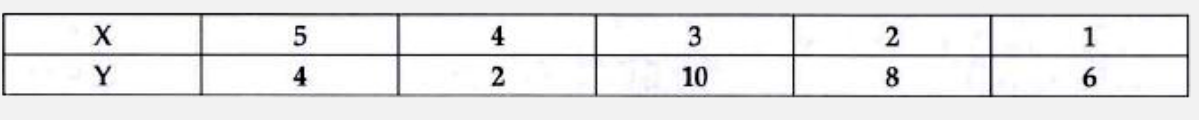
View SolutionIf Cov(x,y) = -2, Σy=30,Σx=25andΣxy=140 , find the number of observations .
View Solution
