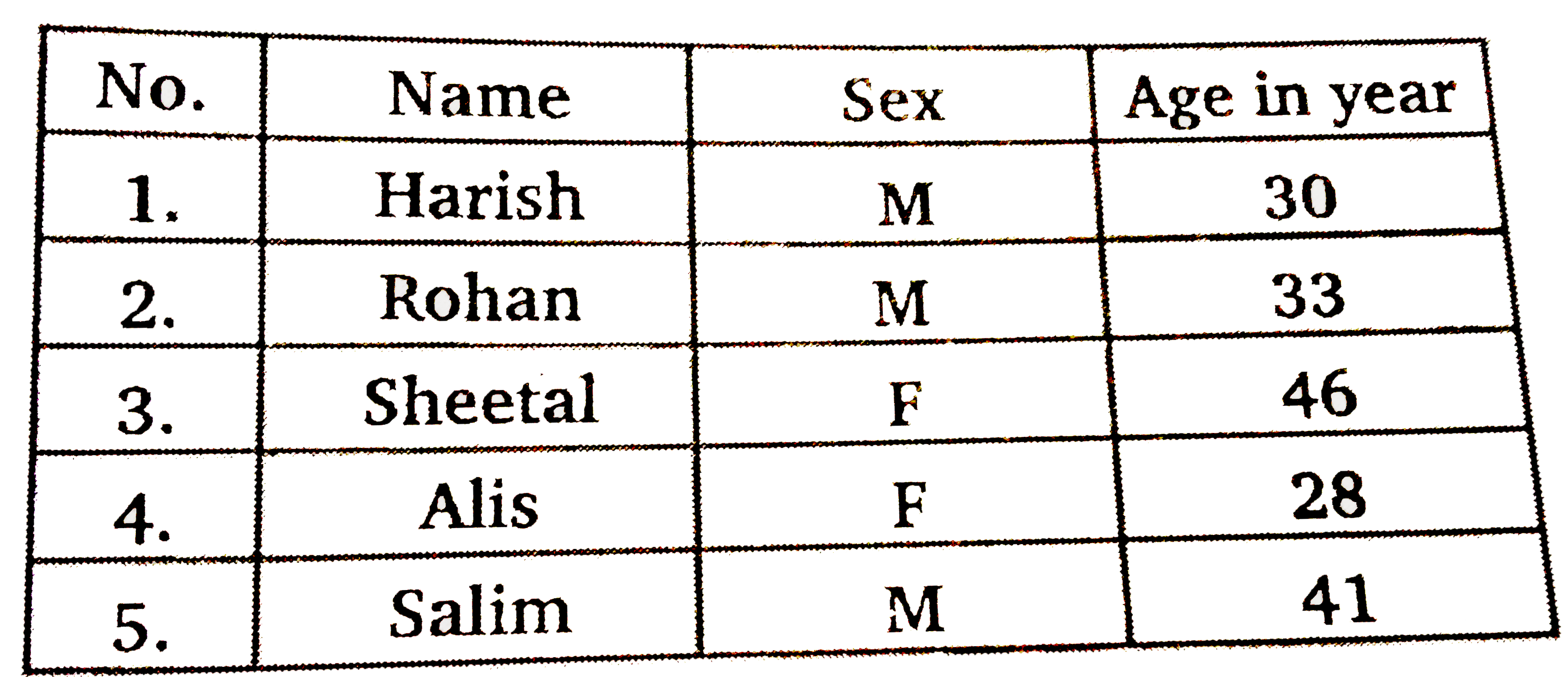
From the employees of a company, 5 persons are selected to represent them in the managing committee of the company. Particulars of five persons are as follows:S.No. Name Sex Age in years1. Harish M 302. Rohan M 333. Sheetal F 464. Alis F 285. Salim M 41A person is selected at random from this group to act as a spokesperson. What is the probability that the spokesperson will be either male or over 35 years?
To solve the problem, we need to find the probability that a randomly selected spokesperson from the group will be either male or over 35 years of age.
1. Identify the total number of employees:
- There are 5 employees in total.
- n(S)=5
2. Identify the number of males in the group:
- The males in the group are Harish (30), Rohan (33), and Salim (41).
- Total males = 3
- n(M)=3
3. Identify the number of employees over 35 years of age:
- The employees over 35 years are Sheetal (46) and Salim (41).
- Total employees over 35 = 2
- n(A)=2
4. Identify the number of males who are also over 35 years of age:
- The only male over 35 is Salim (41).
- Total males over 35 = 1
- n(M∩A)=1
5. Use the formula for the probability of the union of two events:
- The formula for the probability of either event M (male) or event A (over 35) is given by:
P(M∪A)=P(M)+P(A)−P(M∩A)
6. Calculate the probabilities:
- Probability of selecting a male:
P(M)=n(M)n(S)=35
- Probability of selecting someone over 35:
P(A)=n(A)n(S)=25
- Probability of selecting a male who is also over 35:
P(M∩A)=n(M∩A)n(S)=15
7. Substitute the values into the union formula:
P(M∪A)=P(M)+P(A)−P(M∩A)=35+25−15
P(M∪A)=3+2−15=45
Final Answer:
The probability that the spokesperson will be either male or over 35 years is 45.
---
- There are 5 employees in total.
- n(S)=5
- The males in the group are Harish (30), Rohan (33), and Salim (41).
- Total males = 3
- n(M)=3
- The employees over 35 years are Sheetal (46) and Salim (41).
- Total employees over 35 = 2
- n(A)=2
- The only male over 35 is Salim (41).
- Total males over 35 = 1
- n(M∩A)=1
- The formula for the probability of either event M (male) or event A (over 35) is given by:
P(M∪A)=P(M)+P(A)−P(M∩A)
- Probability of selecting a male:
P(M)=n(M)n(S)=35
- Probability of selecting someone over 35:
P(A)=n(A)n(S)=25
- Probability of selecting a male who is also over 35:
P(M∩A)=n(M∩A)n(S)=15
P(M∪A)=P(M)+P(A)−P(M∩A)=35+25−15
P(M∪A)=3+2−15=45
The probability that the spokesperson will be either male or over 35 years is 45.
Topper's Solved these Questions
Similar Questions
From the employees of a company, 5 persons are selected to represent them in the managing committee of the company. Particulars of five persons are as follows:
A person is selected at random from this group to act as a spokeperson. What is the probability that the spokesperson wil be either male or over 35 years?
View SolutionA committee of two persons is selected from two men and two women. What is the probability that the committee will have i. no man? Ii. one man? iii. two men?
View Solution
Knowledge Check
How many 3 - person committees can be selected from a fraternity with 25 members?
A15625B13800C2300D75If seven persons are to be seated in a row, then the probability that two particular persons are seated next to each other is
AA. 17BB. 27CC. 37DD. 47
A committee of two persons is selected from two men and two women. What is the probability that the committee will have i. no man? Ii. one man? iii. two men?
View SolutionIn how many ways 2 persons can be selected from 4 persons?
View SolutionThere are m persons sitting in a row. Two of the mare selected at random. The probability that the two selected persons are together
View Solution(n≥5) persons are sitting in a row. Three of these are selected at random. The probability that no two of the selected persons sit together is
View SolutionPersons are sitting in a row. Two of them are selected. Write the probability that they are together.
View SolutionIf 10 objects are distributed at random among 10 persons, then find the probability that at least one of them will not get anything.
View Solution
