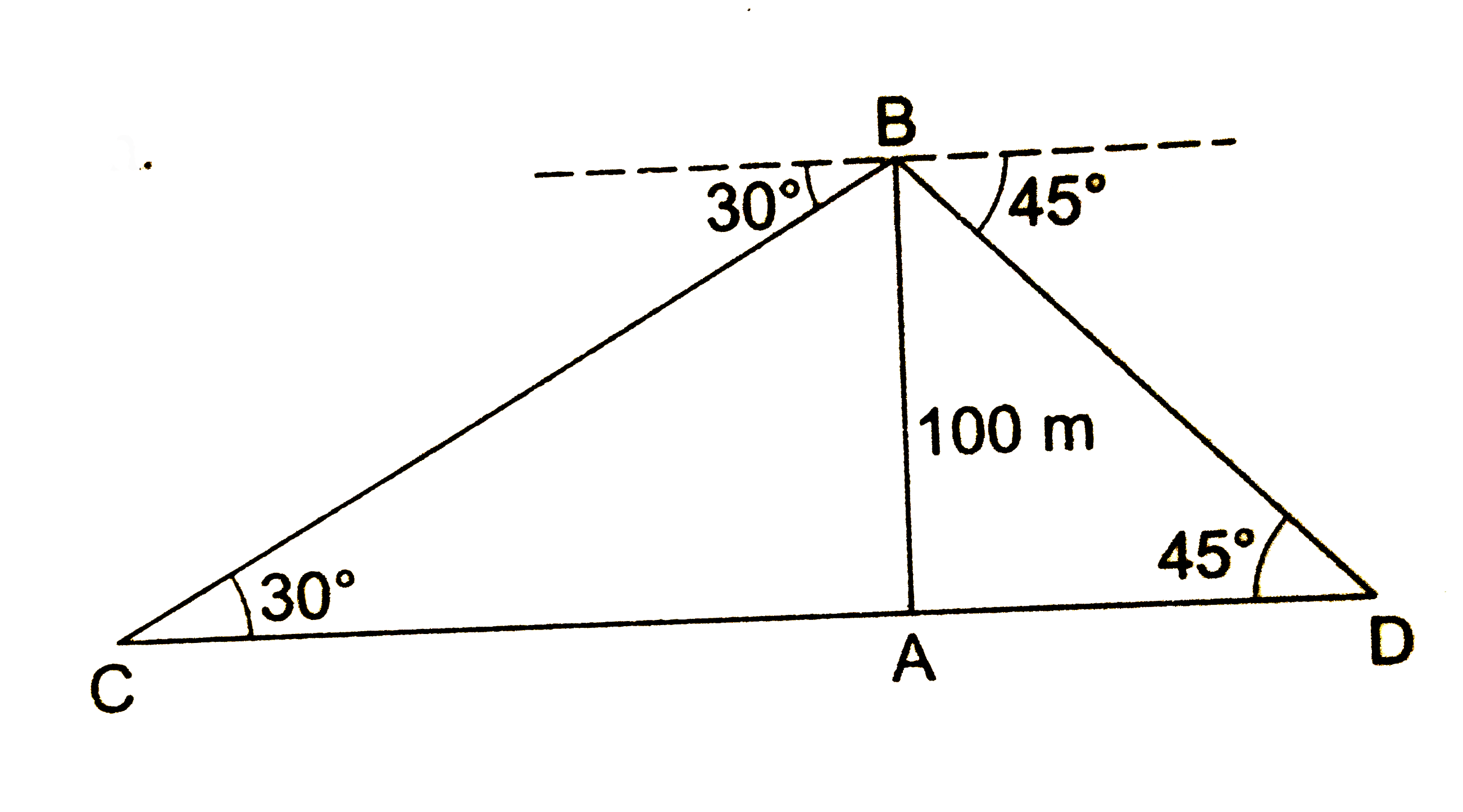
From the top of a tower 100m high, a man observes two cars on the opposite sidesof the tower and in same straight line with itsbase, with angles of depressoin 30∘ and 45∘ respectively. Find the distance between the cars . [Take √3=1.732]
The correct Answer is:273.2m
From right ΔBAD, we have
ADAB=cot45∘=1⇒AD100m⇒AD=100m.
From right ΔBAC, we have
ACAB=cot30∘=√3⇒AC100m=√3.
⇒Ac=(100×1.732)m=173.2m.
∴ distance between the cars.
=(173.2+100)m=273.2m.
Topper's Solved these Questions
- HEIGHTS AND DISTANCESBOOK - RS AGGARWALCHAPTER - HEIGHTS AND DISTANCESEXERCISE - Multiple Choice Questions (Mcq)
25 Videos
- HEIGHTS AND DISTANCESBOOK - RS AGGARWALCHAPTER - HEIGHTS AND DISTANCESEXERCISE - Multiple Choice Questions (Mcq)
25 Videos
- COORDINATE GEOMETRYBOOK - RS AGGARWALCHAPTER - COORDINATE GEOMETRYEXERCISE - Multiple Choice Questions (Mcq)
34 Videos
- LINEAR EQUATIONS IN TWO VARIABLES BOOK - RS AGGARWALCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - QUESTION
2 Videos
Similar Questions
From the top of a 120 m hight tower a man observes two cars on the opposite sides of the tower and in straight line with the base of tower with angles of depression as 60∘ and 45∘. Find the distance between the cars.
View SolutionThe angles of elevation of the top of a tower from two points at a distance of 4 m and 9 m from the base of the tower and in the same straight line with it are 60∘and30∘ respectively . Find the height of the tower .
View Solution
Knowledge Check
An observer standing on a 300 m high tower observes two boats in the same direction, their angle of depression are 60∘and30∘ respectively. The distance between the boats is
A173.2 mB346.4 mC25 mD72 mA straight highway leads to the foot of a tower of height 50 m. From the top of tower, the angles of depression of two cars standing on the highway are 30∘ and 60∘ respectively. What is the distance between the two cars and how far is each car from the tower?
A100√3mB100√3mC86.50 mDNone of theseTwo men are on opposite sides of a tower .They measure the angles of elevation of the top of the tower as 30∘and45∘ respectively .If the height of the tower is 50 metre , the distance between the two men is (Take √3=1.73)
A136.5 metreB50√3 metreC100√3 metreD135.5 metre
Two men are on opposite sides of a tower. They measure the angles of elevation of the top of the tower as 30∘ and45∘ respectively. If the height of the tower is 50metres, find the distance between the two men. [Take √3=1.732]
View SolutionFrom the top of a 60 m high building, the angles of depression of the top and the bottom of a tower are 45∘ and 60∘ respectively. Find the height of the tower. [Take √3=1.73]
View SolutionTwo points A and B are on the same side of a tower and in the same straight line with its base. The angles of depression of these points from the top of the tower are 60∘ and 45∘ respectively. If the height of the tower is 15 m, then find the distance between these points.
View SolutionA player sitting on the top of a tower of height 20 m observes the angle of depression of a ball lying on the ground as'60°. Find the distance between the foot of the tower and the balL Take √3=1.732
View SolutionTwo men are on the opposite sides of a tower. They measure the angles of elevation of the top of the tower as 45∘ and 30∘ respectively. If the height of the tower is 40 m, then the distance between the men is
View Solution
