Question

Give reasons to show that none of the following expressions is a polynomial.
(i) $f\left(x\right)=x+\frac{1}{x}$
(ii) $g\left(x\right)=\sqrt{x}-3$
(iii) $h\left(y\right)=\sqrt[3]{3y}-6$
(iv) $p\left(x\right)=\frac{(x-1)(x-3)}{x}$
(v) $q\left(x\right)=\frac{1}{x+2}$
(vi) $r\left(x\right)=\frac{x+3}{x+4}$

Explanation

(i) `f(x)=x+(1)/(x)` may be written as `f(x)=x+x^(-1)`.
Here , in one term , the exponent of x is - 1 , which is a negative integer .
`thereforef(x)` is not a polynomial .
(ii) `g(x)=sqrt(x)-3` may be written as g(x) `=^(1//2)-3`. Here , in one term , the exponent of x is `(1)/(2)` , which is not a non - negative integer .
`therefore` g (x) is not a polynomial .
(iii) `h(y)=root(3)(y)-6` may be written as `h(y)=y^(1//3)-6`. Here , in one term , the exponent of y is `(1)/(3)`, which is not a non - negative integer.
`therefore h(y)` is not a polynomial .
(iv) `p(x)=((x-1)(x-3))/(x)`
`rArr p(x)=(x^(2)-4x+3)/(x)=(x-4+(3)/(x))=(x-4+3x^(-1))`.
Here , in one term , the exponent of x is -1 , which is a negative integer.
`thereforep(x)` is not a polynomial.
(v) `q(x)=(1)/((x+2))=(x+2)^(-1)`, which is not a polynomial in `(x+2) `and therefore , it is not a polynomial in x .
(vi) On dividing `(x+3)` by `(x+4)` , we get 1 as quotient and -1 as remainder.

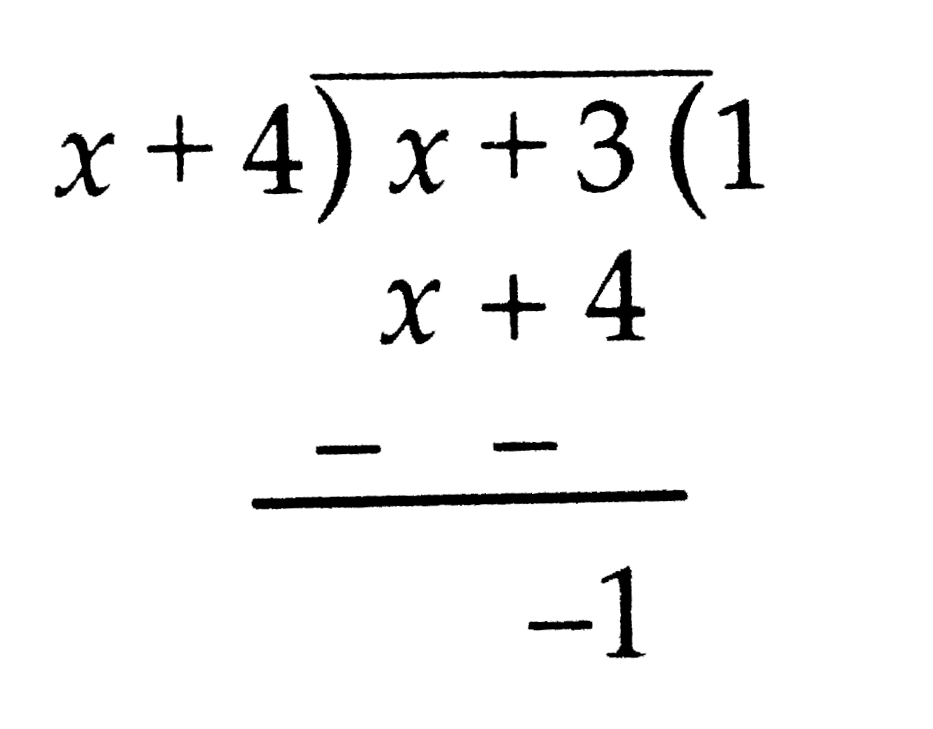
`therefore` we may write , `(x+3)/(x+4)=1-(1)/((x+4))=1-(x+4)^(-1)`, which is not a polynomial .
Hence , r `(x)=(x+3)/(x+4)` is not a polynomial.