Given below are pairs of statements. In each case, combine them using 'if and only if'.
(i) p: In ΔABC,∠B=anl≥C.
q: In ΔABC,AC=AB.
(ii) p: A and B are two sets such that A ⊆ B and B ⊆ A.
q: A=B.
(iii) p: ΔABC is equilateral.
q: ΔABC is equiangular.
(iv) p: {a∈R such that |a|<2}.
q:{a∈R such that (a>−2anda<2)}.
Video Solution
Text Solution
We can combine the given statements as given below.
(i) In ΔABC,∠B=∠C⇔AC=AB.
(ii) For any sets A and B, A=B⇔(A⊆BandB⊆A).
(iii) AΔABC is equilateral ⇔ its is equiangular.
(iv) For every real a,|a|<2⇔(a>−2anda<2).
|
Topper's Solved these Questions
- MATHEMATICAL REASONING BOOK - RS AGGARWALCHAPTER - MATHEMATICAL REASONING EXERCISE - (EXERCISE 29A)
4 Videos
- MATHEMATICAL REASONING BOOK - RS AGGARWALCHAPTER - MATHEMATICAL REASONING EXERCISE - (EXERCISE 29B)
4 Videos
- LogarithmBOOK - RS AGGARWALCHAPTER - LogarithmEXERCISE - Exercise 1
9 Videos
- MEASUREMENT OF ANGLESBOOK - RS AGGARWALCHAPTER - MEASUREMENT OF ANGLESEXERCISE - Exercise 14
16 Videos
Similar Questions
Knowledge Check
Let p: A triangle is equilateral, q: A triangle is equiangular, then inverse of q→p is
AIf a triangle is not equilateral then it is not equiangular.BIf a triangle is not equiangular then it is not equilateral.CIf a triangle is equiangular then it is not equilateralDIf a triangle is equiangular then it is equilateral.In ΔABC, P is a point on BC such that BP:PC=4:5 and Q is the mid - point of BP. Then area (ΔABQ) : area (ΔABC) is equal to :
A1:3B1:9C2:9D2:5If in ΔABC, sides a, b, c are in A.P. then
AB>60∘BB<60∘CB≤60∘DB=|A−C|
If in a ΔABC,a,b,c are in A.P. then it is necessary that
View SolutionThe angles A, B and C of a ΔABC are in A.P. If AB=6,BC=7,then AC=
View SolutionIn an equilateral ΔABC,D is the midpoint of AB and E is the midpoint of AC. Then, ar(ΔABC):ar(ΔADE)=?
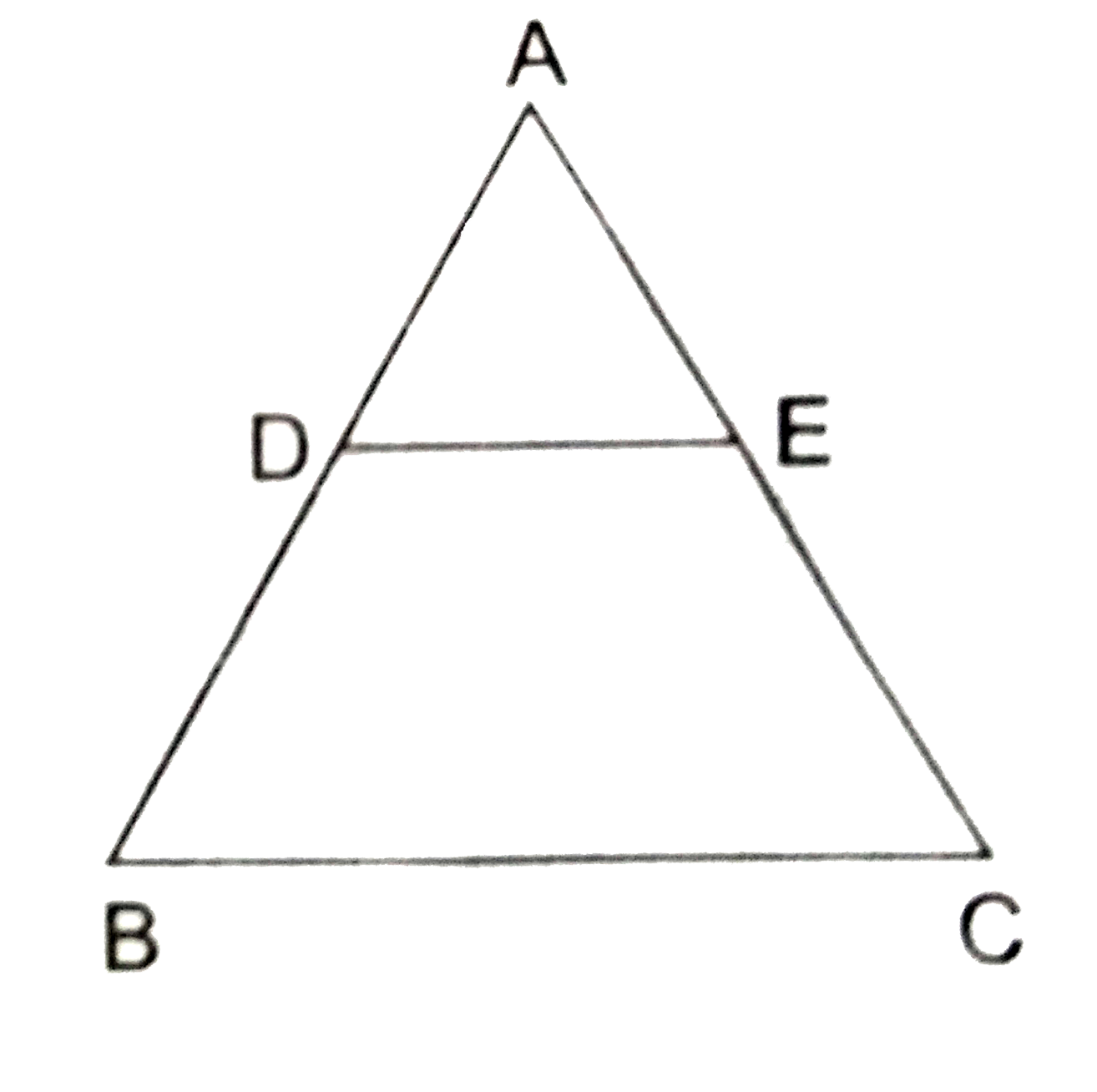
View SolutionIf the anngle A,B,C of a ΔABC are in A.P then :-
View SolutionIn the adjoining figure, P and Q are two points on equal sides AB and AC of a isosceles triangle ABC such that AP = AQ, then
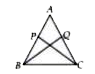
View Solution
