Given that x∈[0,1] and y∈[0,1]. Let A be the event of selecting a point (x,y) satisfying y2≥x and B be the event selecting a point (x,y) satisfying x2≥y, thenSolution in Bengali
P(A∩B)=13
A⊂B
2P(A)=3P(B)
P(B)<P(A)
The correct Answer is:A
(a) 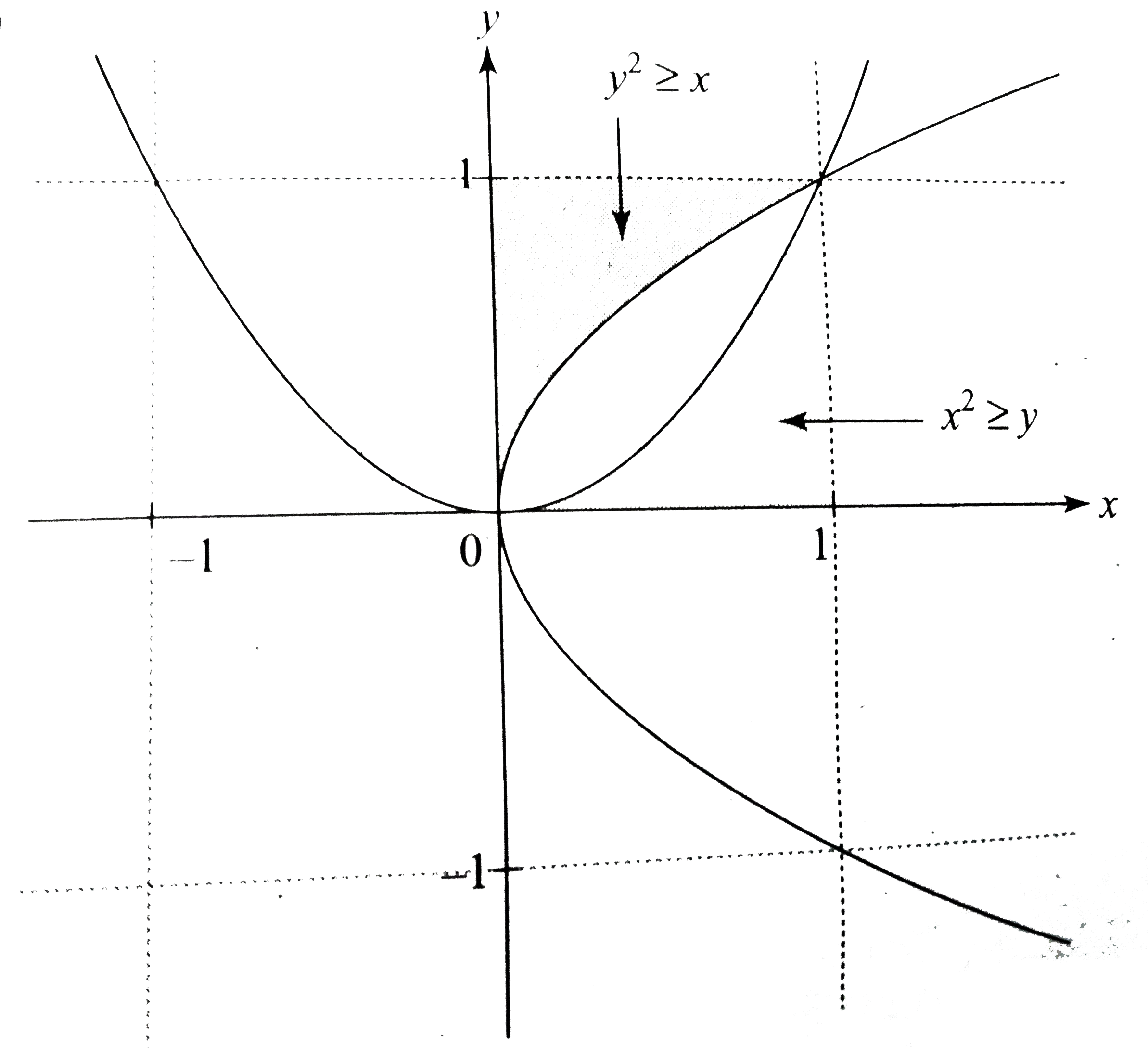
P(A)=∫10√xdx∫10dx=23 and P(B)=∫10√ydy∫10dy=23
∴P(A∩B)=∫10−√xdx−∫10x2dx∫10dx=23−13=13
Topper's Solved these Questions
Similar Questions
Prove the following by using the principle of mathematical induction for all n∈N:13+23+33+...........+n3=(n(n+1)2)2
View SolutionLet ω be a complex cube root unity with ω≠1. A fair die is thrown three times. If r1,r2andr3 are the numbers obtained on the die, then the probability that ωr1+ωr2+ωr3=0 is 1/18 b. 1/9 c. 2/9 d. 1/36
View SolutionThe area of the region containing the points (x, y) satisfy-ing 4<=x^(2)+y^(2)<=2(|x|+|y|) is
View SolutionThe number of function f:[0,1]→[0,1] satisfying |f(x)−f(x)|=|x−y| for all x,y in [0,1]is
View SolutionArea bounded by the region consisting of points (x,y) satisfying y≤√2−x2,y2≥x,√y≥−x is
View SolutionSketch the regions which points satisfy |x+y|≥2.
View SolutionWhat is the set of points (x,y) satisfying the equations x2+y2=4andx+y=2?
View SolutionGiven that x∈[0,1] and y∈[0,1] . Let A be the event of (x,y) satisfying y2≤x and B be the events of (x,y) satisfying x2≤y . Then .
View SolutionAn ordered triplet solution (x,y,z) with x,y,z ∫ (0,1) and satisfying x2+y2+z2+2xyz=1 is
View SolutionThe area {xy|x∣≥y≥x2} is equal to :
View SolutionThe area (in sq. units) of the region {(x,y),x≥0,y≥0,y≥x−2,andy≤√x} is
View SolutionSketch the regions which points satisfy |x+y|≥2.
View Solutiony=(x+y)−1/2 के बिंदु (0,1) पर स्पर्श रेखा का समी है
View SolutionGiven that x in [0,1] and y in [0,1] . Let A be the event of (x,y) satisfying y^(2) le x and B be the events of (x,y) satisfying x^(2) le y . Then .
View Solutionदिया है x∈[0,1] तथा y∈[0,1] माना (x,y)की घटना A समीकरण y2≤xको संतुष्ट करती है तथा (x,y)की घटना B समीकरण x2≤y को संतुष्ट करती है , तब
View SolutionAn ordered triplet solution (x,y,z) with x,y,z ∫ (0,1) and satisfying x2+y2+z2+2xyz=1 is
View Solution
