How many roots does the following equation possess $3^{| x |} (| 2 - | x ∣ ∣) = 1$ ?

A

1

B

2

C

3

D

4

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

We have,
$3^{| x |} {2 - | x |} = 1$ ..........(i)
$\Rightarrow 2 - | x | = 3^{- | x |}$
In order to determine the number of roots of (i), it is sufficient to find the points of intersection of the curves $y = 2 - | x |$ and $y = 3^{- | x |}$ . Graphs of these two curves are shown in Fig.2.

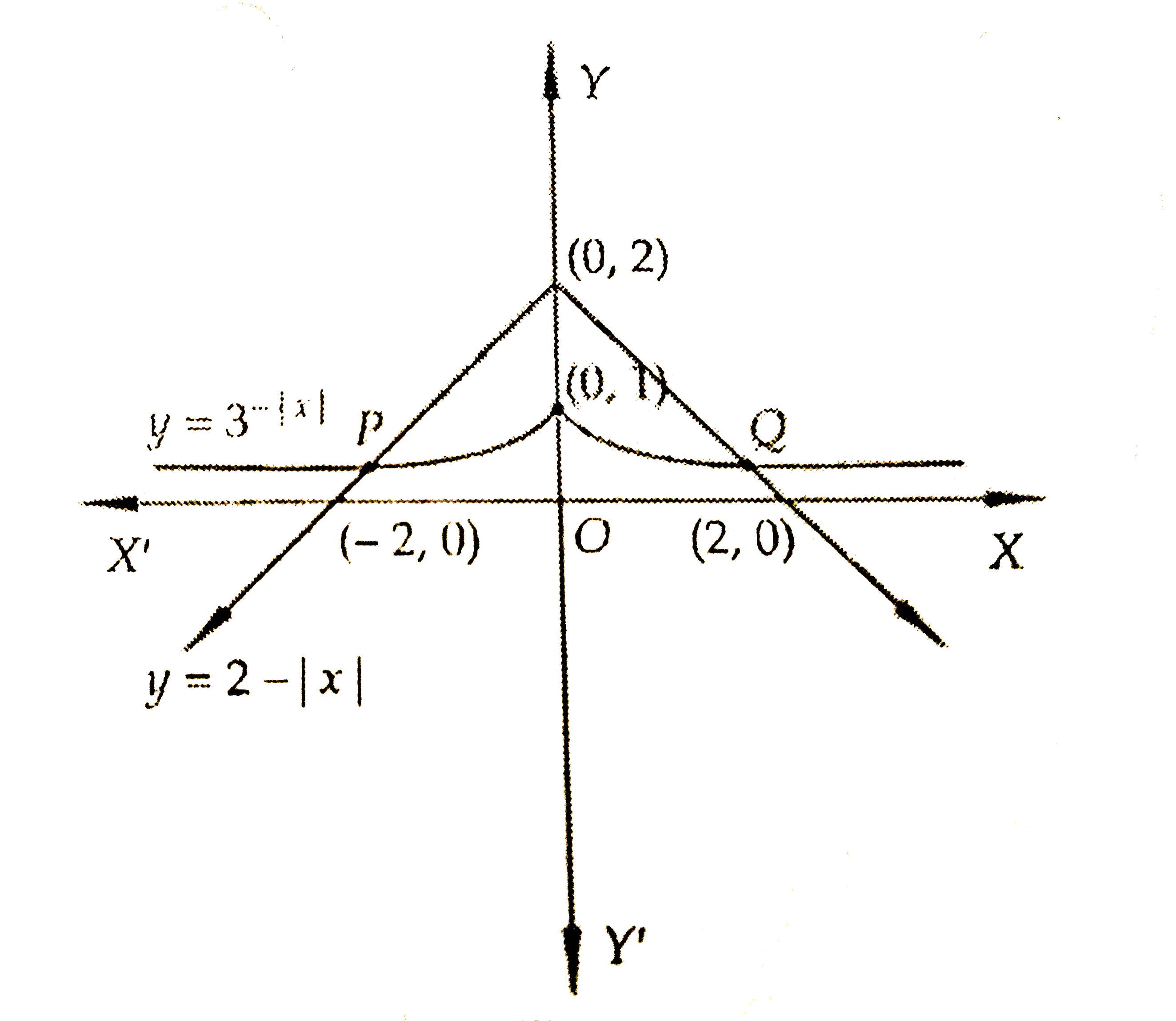
We observe that the two curves intersect at two points P and Q. Hence, the equation (i) has two real solutions. Also the solutions belong to the interval (-2,2).

|

Updated on:21/07/2023

Class 12 MATHS MISCELLANEOUS EQUATIONS AND INEQUATIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
How many roots foes the following equation possess $3^{| x |} (| 2 - x | ∣) = 2$ ?
A2
B3
C4
D6
Question 2 - Select One
For $x > 1$ , how many roots/solutions of the following equation exist.
${log}_{2 x} (\frac{2}{x}) {log}_{2}^{2} x + {log}_{2}^{4} x = 1$
ANone
BOne
CTwo
DInfinitely many
Question 3 - Select One
How many real roots does the quadratic equation $f (x) = x^{2} + 3 | x | + 2 = 0$ have ?
AOne
BTwo
CFore
DNo real root

OBJECTIVE RD SHARMA-MISCELLANEOUS EQUATIONS AND INEQUATIONS -Chapter Test

Home
Ask Doubt
Profile