Identify the term containing u2 in the expression u3+3u2υ+3uυ2+υ3 and write its coefficient.
To solve the question, we need to identify the term containing u2 in the expression u3+3u2v+3uv2+v3 and write its coefficient.
1. Identify the Expression:
The given expression is:
u3+3u2v+3uv2+v3
2. Locate the Term with u2:
We need to look for the term that contains u2. In the expression, we can see that:
- The first term is u3 (does not contain u2).
- The second term is 3u2v (contains u2).
- The third term is 3uv2 (does not contain u2).
- The fourth term is v3 (does not contain u2).
Therefore, the term containing u2 is 3u2v.
3. Identify the Coefficient:
The coefficient of a term is the numerical factor that multiplies the variable part. In the term 3u2v:
- The coefficient of u2 is 3v.
Final Answer:
The term containing u2 is 3u2v and its coefficient is 3v.
---
The given expression is:
u3+3u2v+3uv2+v3
We need to look for the term that contains u2. In the expression, we can see that:
- The first term is u3 (does not contain u2).
- The second term is 3u2v (contains u2).
- The third term is 3uv2 (does not contain u2).
- The fourth term is v3 (does not contain u2).
Therefore, the term containing u2 is 3u2v.
The coefficient of a term is the numerical factor that multiplies the variable part. In the term 3u2v:
- The coefficient of u2 is 3v.
The term containing u2 is 3u2v and its coefficient is 3v.
Topper's Solved these Questions
- ALGEBRAIC EXPRESSIONSBOOK - NCERT EXEMPLARCHAPTER - ALGEBRAIC EXPRESSIONSEXERCISE - Exercise(Write the correct answer.)
16 Videos
- ALGEBRAIC EXPRESSIONSBOOK - NCERT EXEMPLARCHAPTER - ALGEBRAIC EXPRESSIONSEXERCISE - Exercise(Fill in the blanks)
15 Videos
- COMPARING QUANTITIESBOOK - NCERT EXEMPLARCHAPTER - COMPARING QUANTITIESEXERCISE - THINK AND DISCUSS
24 Videos
Similar Questions

A disc is rolling (without slipping) on a horizontal surface. C is its centre and Q and P are two point equidistanec from C. let υp,υQ and υC be the magnitude of velocities of points P, Q, and C repsectively,
(a). υQ>υC>υP
(b). υQ<υC<υP
(c). υQ=υP,υC=12υP
(d). υQ<υC>υP
View SolutionWrite the following in their standard form:
4u2+u−3u6+2
View Solution
Knowledge Check
A vessel of volume V contains a mixture of 1 mole of hydrogen and 1 mole oxygen (both considered as ideal). Let f1(v)dv, denote the fraction of molecules with speed between v and (v+ dv) with f2(v)dv, similarly for oxygen . Then ,
Af1(υ)+f2(υ)=f(υ) obeys the Maxwell's distribution law.Bf1(υ),f2(υ) will obey the maxwell's distribution law seperately.CNeither f1(υ), nor f2(υ) will obey the Maxwell's distribution law.Df2(υ) and f1(υ) will be the same.
Find the zeros of the polynomial f(u)=4u2+8u , and verify the relationship between the zeros and its coefficients.
View SolutionIdentify the physical quantity x defined as x=IFυ2WI3, where I is moment of inertia, F is force, υ is veloicty, W is work and I is length.
View SolutionIdentify the physical quantity x defined as x=IFυ2WI3, where I is moment of inertia, F is force, υ is veloicty, W is work and I is length.
View SolutionWhat is the value of u in the system of equations 3 (2u + v) = 7uv, 3 (u + 3v) = 11uv ?
View SolutionThe SI unit of energy is J=kgm2s−2, that of speed υ is ms−1 and of acceleration a is ms−2 which of the formulae for kinetic energy (K) given below can you rule out on the basis of dimensional arguments (m stands for the mass of the body).
(a) K=m2υ3 (b) K=12mυ2 (c ) K= ma
(d) K=316mυ2 (e ) K=12mυ2+ma
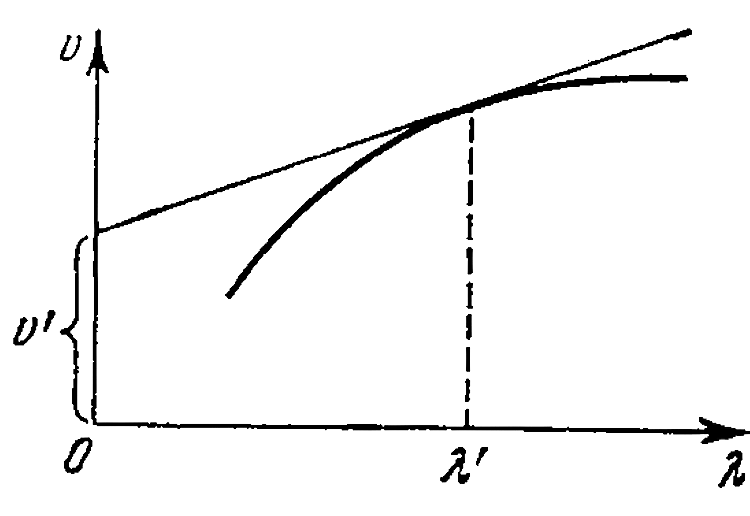
View SolutionUsing the definition of the group velocity u, derive Rayleigh's fromula (5.5d). Demonstrate that in the vicinity of λ=λ' the velocity u is equal to the segment υ' cut by the tangent of the curve υ(λ) at the point λ' (Fig.)
View SolutionTwo bodies of equal masses move with uniform velocities υand3υ respectively. Find the ratio of their kinetic energies.
View Solution
