If 2x+3y−1=0 is the regression line of y on x, then byx is ……………..
The correct Answer is:−23
To find the regression coefficient byx from the given regression line equation 2x+3y−1=0, we can follow these steps:
Step 1: Rearrange the equation to express y in terms of x.
Starting with the equation:
2x+3y−1=0
We can rearrange it to isolate y:
3y=−2x+1
Step 2: Solve for y.
Now, divide each term by 3 to solve for y:
y=−23x+13
Step 3: Identify the slope of the regression line.
In the equation y=mx+c, m represents the slope. From our equation:
y=−23x+13
we can see that the slope m is:
m=−23
Step 4: Conclude the value of byx.
The slope of the regression line byx is equal to the slope we found:
byx=−23
Thus, the value of byx is:
−23
---
Starting with the equation:
2x+3y−1=0
3y=−2x+1
Now, divide each term by 3 to solve for y:
y=−23x+13
In the equation y=mx+c, m represents the slope. From our equation:
y=−23x+13
we can see that the slope m is:
m=−23
The slope of the regression line byx is equal to the slope we found:
byx=−23
−23
Topper's Solved these Questions
- CORRELATION AND REGRESSION Book:ML KHANNAChapter:CORRELATION AND REGRESSION Exercise:PROBLEM SET (1) (TRUE AND FALSE)
Explore 9 Videos
- CORRELATION AND REGRESSION Book:ML KHANNAChapter:CORRELATION AND REGRESSION Exercise:SELF ASSESSMENT TEST
Explore 10 Videos
- CORRELATION AND REGRESSION Book:ML KHANNAChapter:CORRELATION AND REGRESSION Exercise:PROBLEM SET (1) TRUE/FALSE
Explore 6 Videos
- CONCEPTS OF SET THEORY Book:ML KHANNAChapter:CONCEPTS OF SET THEORY Exercise:Self Assessment Test
Explore 13 Videos
- DEFINITE INTEGRALBook:ML KHANNAChapter:DEFINITE INTEGRALExercise:Miscellaneous Questions (Assertion/Reason)
Explore 1 Video
Similar Questions
Knowledge Check
If the regression equation x on y is 3x+2y=26 then bxy equals to
A3/2B2/3C−3/2D−2/3If y=5−2.8x and x=3–0.5y be the regression lines ,then the value of byx is
A−0.5B−2.8C−0.5D−2
Among the given regression lines 6x+y−31=0 and 3x+2y−26=0,the regression line of x on y is…........
View Solution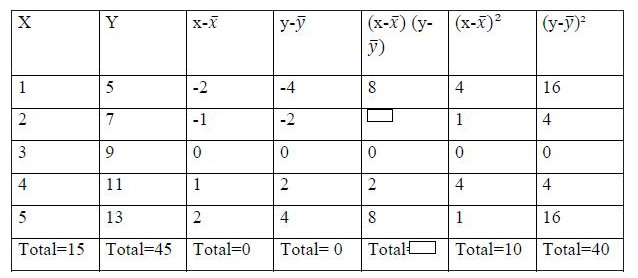
Mean of x=¯x=□
Mean y=¯y=□
bxy=□□
byx=□□
Regression equation of x on y is x−¯x=bxy(y−¯y)
∴ Regression equation of x on y is □
Regression equation of y on x is y−¯y=byx(x−¯x)
∴ Regression equation of y on x is □
View SolutionThe regression equation of x on y is 40x-18y = 214… (i)
The regression equation of y on x is 8x -10y +66 =0….. (ii)
Solving equations i and ii,
¯x=□
¯y=□
∴byx=□□
∴bxy=□□
∴r=□
View SolutionFor the data {x,y}=(1,5),(3,7),(5,9),(6,12),(8,15), find
(a) regression line of Y on X in the form y−y=bx(x−y)
(b) regression line of X on Y in the form x−x=bxy(y−y)
Estimate the value of y when x=4 and the value of x when y=11
View SolutionThe equations of the two lines of regression are 3x+y=5 and 2x+3y=6. Find
(a) The regression line of Y and X.
(b) mean values of x and y
(c) correlation coefficient x and y
(d) the angle between the regression lines.
View SolutionTwo regression lines are given as 3x-4y+8=0 and 4x-3y-1 = 0
Consider the following statements :
1. The regression line of y on x is y=34x+2
2. The regression line of x on y is x=34y+14.
Which of the above statements is/are correct ?
View Solution