If A. B and C are three points on a line, and B lies between A and C then prove that AB+BC=AC.
To prove that AB+BC=AC when points A, B, and C are collinear and B lies between A and C, follow these steps:
1. Identify the Points on the Line:
Let A, B, and C be three points on a straight line such that B is between A and C.
2. Visual Representation:
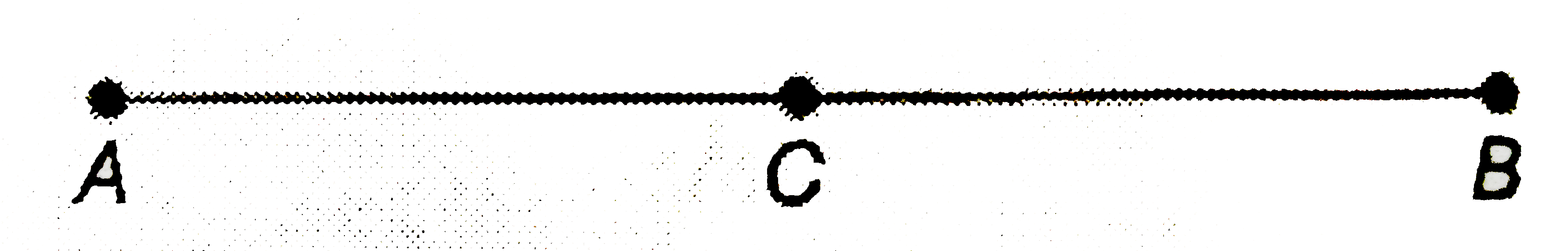
Draw a straight line and mark the points A, B, and C on it. The arrangement should be A, B, C from left to right.
A−−−−B−−−−C
3. Define the Lengths:
Let \( AB \) be the distance from point A to point B, and \( BC \) be the distance from point B to point C. The total distance from A to C is denoted as \( AC \).
4. Express the Total Length:
According to the arrangement of the points on the line, the distance \( AC \) can be expressed as the sum of the distances \( AB \) and \( BC \):
\(
AC = AB + BC
\)
5. Apply Euclid's Fourth Axiom:
According to Euclid's Fourth Axiom, if two segments coincide (i.e., they lie on the same line and occupy the same space), then they are equal. Since the segments \( AB + BC \) coincide with segment \( AC \), we can conclude:
\(
AB + BC = AC
\)
6. Conclusion:
Therefore, we have proved that:
\(
AB + BC = AC
\)
Let A, B, and C be three points on a straight line such that B is between A and C.
Draw a straight line and mark the points A, B, and C on it. The arrangement should be A, B, C from left to right.
Let \( AB \) be the distance from point A to point B, and \( BC \) be the distance from point B to point C. The total distance from A to C is denoted as \( AC \).
According to the arrangement of the points on the line, the distance \( AC \) can be expressed as the sum of the distances \( AB \) and \( BC \):
\(
AC = AB + BC
\)
According to Euclid's Fourth Axiom, if two segments coincide (i.e., they lie on the same line and occupy the same space), then they are equal. Since the segments \( AB + BC \) coincide with segment \( AC \), we can conclude:
\(
AB + BC = AC
\)
Therefore, we have proved that:
\(
AB + BC = AC
\)
Topper's Solved these Questions
- INTRODUCTION TO EUCLIDS GEOMETRYBOOK - NCERT ENGLISHCHAPTER - INTRODUCTION TO EUCLIDS GEOMETRYEXERCISE - Exercise 5.2
2 Videos
- INTRODUCTION TO EUCLIDS GEOMETRYBOOK - NCERT ENGLISHCHAPTER - INTRODUCTION TO EUCLIDS GEOMETRYEXERCISE - Exercise 5.1
7 Videos
- HERONS FORMULABOOK - NCERT ENGLISHCHAPTER - HERONS FORMULAEXERCISE - Solved Examples
6 Videos
- LINEAR EQUATIONS IN TWO VARIABLESBOOK - NCERT ENGLISHCHAPTER - LINEAR EQUATIONS IN TWO VARIABLESEXERCISE - Exercise 4.1
2 Videos
Similar Questions
If A, B and C are three poins on a line and B lies between A and C, then prove that AB + BC = AC.

View SolutionIf A, B and C are three sets such that A∪B=A∪CandA∩B=A∩C, then prove that B=C.
View SolutionIf a point C lies between two points A and B such that AC = BC, then prove that AC=12AB. Explain by drawing the figure.
View SolutionIf A,B and C are three sets such that A∪B=CandA∩B=ϕ, then prove A=C−B.
View SolutionIf A, B and C are three matrices of the same order, then prove that A=B⇒A+C=B+C .
View SolutionIn ABC , if BD⊥AC and BC2=2 ACCD , then prove that AB=AC .
View SolutionIn Figure, ABCD is a parallelogram. E and F are the mid-points of the sides AB and CD respectively. Prove that the line segments AF and CE triset (divide into three equal parts) the diagonal BD.
View SolutionA, B, C, D are four consecutive points on a circle such that AB=CD. Prove that AC=BD
View SolutionABC is a triangle in which AB=AC and D is any point in BC . Prove that AB2−AD2=BDCD .
View SolutionIn ABC, D and E are points on sides AB and AC respectively such that AD×EC=AE×DB . Prove that DEBC .
View Solution
