If a, b c are in GP and a1x=b1y=c1z, then x, y, z are in
AP
GP
HP
None of these
The correct Answer is:A
To solve the problem, we need to show that if a,b,c are in geometric progression (GP) and a1x=b1y=c1z, then x,y,z are in arithmetic progression (AP).
1. Understanding GP:
Since a,b,c are in GP, we can express b in terms of a and c:
b=√ac
2. Setting up the equation:
Given that a1x=b1y=c1z, let's denote this common value as k:
a1x=k,b1y=k,c1z=k
3. Expressing a,b,c in terms of k:
From the equations above, we can express a,b,c as:
a=kx,b=ky,c=kz
4. Substituting b in terms of a and c:
Since b=√ac, we substitute the expressions for a,b,c:
ky=√kx⋅kz
Simplifying the right side:
ky=√kx+z=kx+z2
5. Equating the exponents:
Since the bases are the same (both are k), we can equate the exponents:
y=x+z2
6. Rearranging the equation:
Rearranging gives us:
2y=x+z
7. Conclusion:
The equation 2y=x+z indicates that x,y,z are in arithmetic progression (AP).
Final Result:
Thus, we conclude that if a,b,c are in GP and a1x=b1y=c1z, then x,y,z are in AP.
Since a,b,c are in GP, we can express b in terms of a and c:
b=√ac
Given that a1x=b1y=c1z, let's denote this common value as k:
a1x=k,b1y=k,c1z=k
From the equations above, we can express a,b,c as:
a=kx,b=ky,c=kz
Since b=√ac, we substitute the expressions for a,b,c:
ky=√kx⋅kz
Simplifying the right side:
ky=√kx+z=kx+z2
Since the bases are the same (both are k), we can equate the exponents:
y=x+z2
Rearranging gives us:
2y=x+z
The equation 2y=x+z indicates that x,y,z are in arithmetic progression (AP).
Thus, we conclude that if a,b,c are in GP and a1x=b1y=c1z, then x,y,z are in AP.
Topper's Solved these Questions
Similar Questions
Knowledge Check
If a,b,c are in G.P. and a1x=b1y=c1z, then x,y,z are in-
AH.P.BG.P.CA.P.DNone of theseIf x,y and z are in G.P. and ax=by=cz then
Alogba=logacBlogcb=logacClogba=logcbDnone of these
IF a,b,c are in G.P and a1x=b1y=c1z then x,y,z are in
View SolutionIf a,b,c are in G.P. and a1/x=b1/y=c1/z, then xyz are in AP (b) GP (c) HP (d) none of these
View SolutionIf x,y,z are in GP andax=by=cz then
View SolutionWhich of the foliowing statement(s) is/are true? (A) If ax=by=czanda,b,c are in GP, then x, y, z are in HP (B) If a1x=b1y=c1z and a,b,c are in GP, then x,y,z are in AP
View SolutionIf x,y,z are in G.P and ax=by=cz,then
View Solution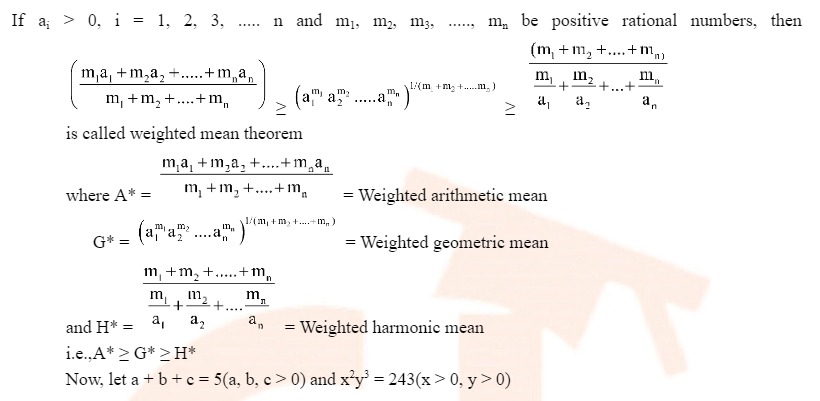
1. The greatest value of ab3c is -
(a) 3
(b) 9
(c) 27
(d) 81
2. Which statement is correct -
(a) 15≥11a+3b+1c
(b) 125≥11a+9b+1c
(c) 15≥11a+9b+1c
(d) 125≥11a+6b+1c
3. The least value of x2+3y+1 is -
(a) 15
(b) greater than 15
(c) 3
(d) less than 15
View Solution
