If a pair of variable straight lines x2+4y2+αxy=0 (where α is a real parameter) cut the ellipse x2+4y2=4 at two points A and B, then the locus of the point of intersection of tangents at A and B is
x−2y=0
2x−y=0
x+2y=0
2x+y=0
Let the point of intersection of tangents A and B be P(h,k).Then the equation of AB is
xh4+yk1=1 (1)
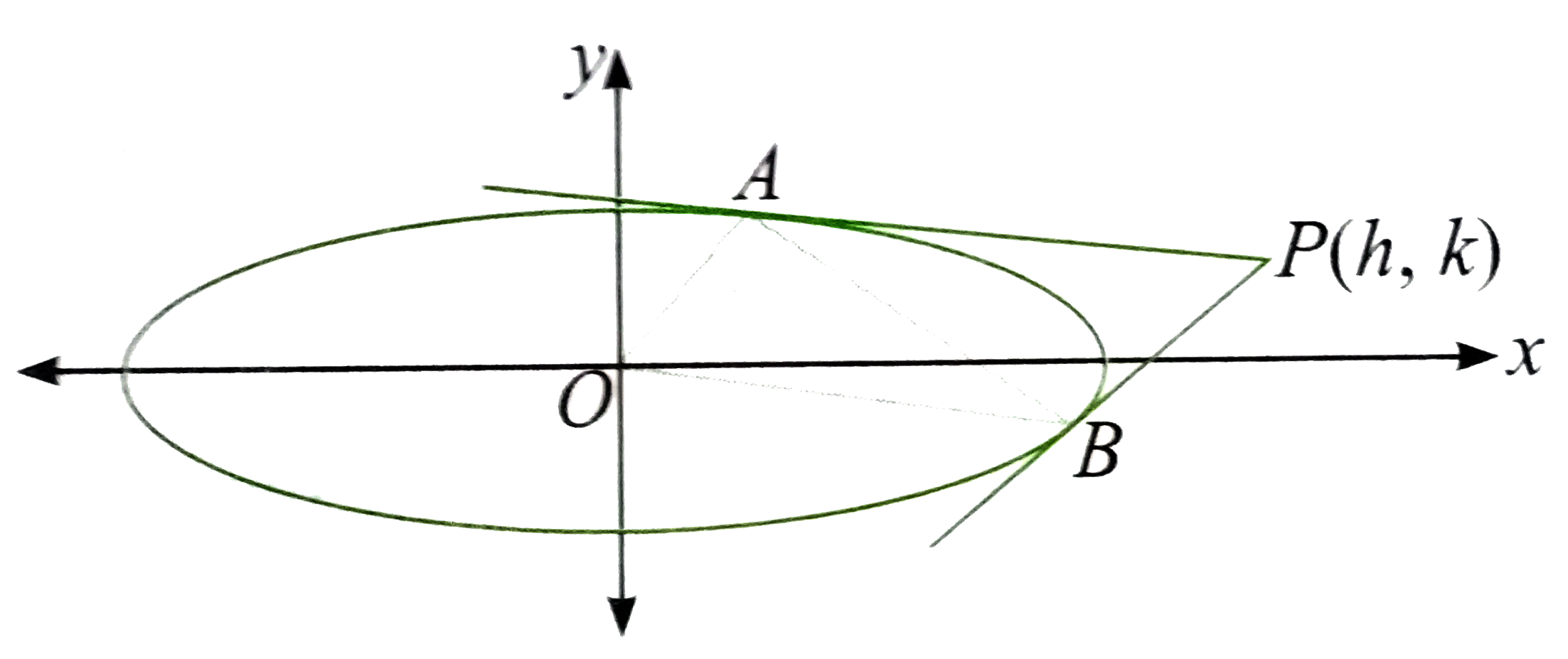
Homogennizing ithe equation of ellipse using (1), we get
x24+y21=(xh4+yk1)2
or x2(h2−h16)+y2(k2−1)+2hk4xy=0 (2)
The given equation of OA and OB is
x2+4y2+αxy=0 (3)
Sinc (2) and (3) repreetn the same line,
h2−416+k2−14=hk2a
orh2−4=4(k2−1)
or h2−4k2=0
Therefore, the locus is (x−2y)(x+2y)=0
Similar Questions
If a pair of variable straight lines x2+4y2+αxy=0 (where α is a real parameter) cut the ellipse x2+4y2=4 at two points A and B, then the locus of the point of intersection of tangents at A and B is
View SolutionIf the line 5x−4y−12=0 meets the parabola x2−8y in A and B then the point of intersection of the two tangents at A and B is
View Solution
Knowledge Check
If a pair of variable straight lines x2+4y2+αxy=0 (where α is a real parameter) cut the ellipse x2+4y2=4 at two points A and B, then the locus of the point of intersection of tangents at A and B is
Ax−2y=0B2x−y=0Cx+2y=0D2x+y=0If a pair of variable straight lines x2+Ay2+axy=0 (where a is a real parameter) cut the ellipse x2+Ay2=4 at two points, then locus of the point of intersection of tangents at A and B is:
A4x2−y2=0Bx2−4y2=0Cx2+4y2−16=0Dx2−4y2−32=0If the line pass through the point(-3,-5) and intersect the ellipse x24+y29=1 at a point A and B . Then the locus of mid point of line joining A and B is
A4x2+9y2+20x+27y=0B9x2+4y2+20x+27y=0C4x2+9y2+27x+20y=0D9x2+4y2+27x+20y=0
if a variable tangent of the circle x2+y2=1 intersects the ellipse x2+2y2=4 at P and Q. then the locus of the points of intersection of the tangents at P and Q is
View SolutionIf a tangent to the parabola y2=4ax intersects the x2a2+y2b2=1 at Aand B, then the locus of the point of intersection of tangents at A and B to the ellipse is
View SolutionA variable parabola y2=4ax,a (where a≠−14) being the parameter, meets the curve y2+x−2=0 at two points. The locus of the point of intersecion of tangents at these points is
View SolutionThe line 2x+y=3 intersects the ellipse 4x2+y2=5 at two points. The point of intersection of the tangents to the ellipse at these point is
View SolutionThe line 2x + y = 3 intersects the ellipse 4x2+y2=5 at two points. The tangents to the ellipse at these two points intersect at the point:
View Solution
