If A={a,b,c}, then the relation R={(b,c)} on A is
reflexive only
symmetric only
transitive only
reflexive and transitive only
The correct Answer is:C
Clearly, R is not reflexive, because (a, a), (b, b), (c, c) cancelin R.
R is not symmetric because (b,c)∈R but (c,b)cancelin R.
However, R is a transitive relation on set A.
Hence, option (c ) is correct.
Topper's Solved these Questions
- BOOK - OBJECTIVE RD SHARMACHAPTER - CARTESIAN PRODUCT OF SETS AND RELATIONS EXERCISE - Section II - Assertion Reason Type6videos
CARTESIAN PRODUCT OF SETS AND RELATIONS
- BOOK - OBJECTIVE RD SHARMACHAPTER - CARTESIAN PRODUCT OF SETS AND RELATIONS EXERCISE - Exercise38videos
CARTESIAN PRODUCT OF SETS AND RELATIONS
- BOOK - OBJECTIVE RD SHARMACHAPTER - CARTESIAN PRODUCT OF SETS AND RELATIONS EXERCISE - Chapter Test31videos
CARTESIAN PRODUCT OF SETS AND RELATIONS
- BOOK - OBJECTIVE RD SHARMACHAPTER - AREAS OF BOUNDED REGIONSEXERCISE - Chapter Test60videos
AREAS OF BOUNDED REGIONS
- BOOK - OBJECTIVE RD SHARMACHAPTER - CIRCLESEXERCISE - Chapter Test55videos
CIRCLES
Similar Questions
Knowledge Check
- Question 1 - Select One
If a,b and c are the sides of a traiangle such that b.c=λ2, then the relation is a,λandA is
Ac≥2λsin(C2)Bb≥2λsin(A2)Ca≥2λsin(A2)DNone of these - Question 2 - Select One
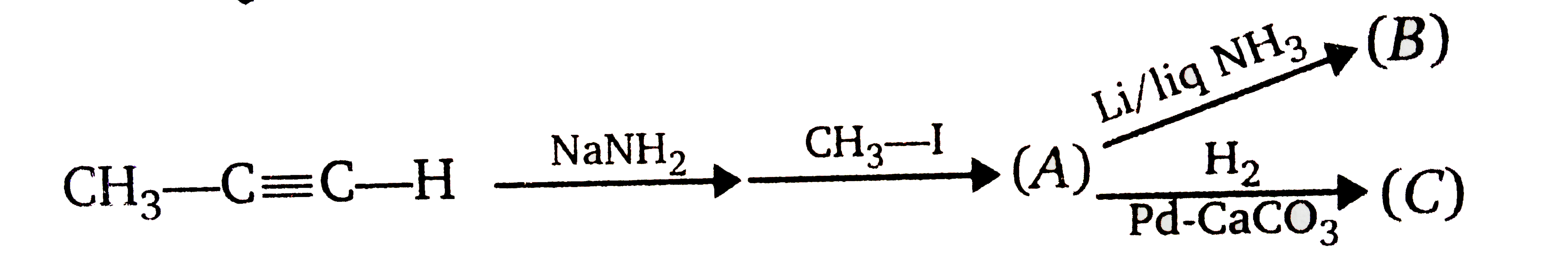
Relation between (B) and (C) is:AEnantiomerBDiastereomersCGeometrical isomerDMeso - Question 3 - Select One
Let R be a relation on A = {a, b, c} such that R = {(a, a), (b, b), (c, c)}, then R is
AReflexive onlyBSymmetric onlyCNon - transitiveDEquivalence
Given a set A = {a, b, c, d}, then the relation:
R={(a,a),(b,b),(c,c),(d,d)} is reflexive?.View SolutionIf A={a, b, c, d}, then a relation R={(a, b), (b, a), (a, a)} on A is (a)symmetric and transitive only (b)reflexive and transitive only (c) symmetric only (d) transitive only
View SolutionLet A={a,b,c) and the relation R be defined on A as follows: R={(a,a),(b,c),(a,b)}. Then, write minimum number of ordered pairs to be added in R to make it reflexive and transitive.
View SolutionLet R={(a,a),(b,c),(a,b)} be a relation on a set A={a,b,c}. Then the minimum number of ordered pairs which when added to R make it an equivalence realtion are ...
View SolutionIf A = {3, 5, 7, 9} and B = {a, b, c}, then which of the following are relations from A to B ? Give reasons for your answer.
(i) R1={(3,a),(5,b),(5,c)}
(ii) R2={(3,c),(9,b),(5,a)}
(iii) R3={(3,c),(b,c),(7,b),(9,a)}
(iv) R4={(3,b),(7,c),(c,a),(b,9)}View Solution

