If α and β are the complex roots of the equation (1+i)x2+(1−i)x−2i=0 where i=√−1, the value of |α−β|2 isSolution in Punjabi
The correct Answer is:5
∵(1+i)x2+(1−i)x−2i=0
⇒x2+(1−i)(1+i)x−2i(1+i)=0
⇒x2−ix−(1+i)=0
∴α+β=i and αβ=−(1+i)
∴α−β=√(α+β)2−4αβ=√i2+4(1+i)=√(3+4i)
|α−β|=√√9+16=√5
∴|α−β|2=5
Topper's Solved these Questions
- BOOK - ARIHANT MATHSCHAPTER - THEORY OF EQUATIONSEXERCISE - Exercise (Matching Type Questions)2videos
THEORY OF EQUATIONS
- BOOK - ARIHANT MATHSCHAPTER - THEORY OF EQUATIONSEXERCISE - MATCH TYPE2videos
THEORY OF EQUATIONS
- BOOK - ARIHANT MATHSCHAPTER - THEORY OF EQUATIONSEXERCISE - Exercise (Passage Based Questions)21videos
THEORY OF EQUATIONS
- BOOK - ARIHANT MATHSCHAPTER - THE STRAIGHT LINESEXERCISE - The Straight Lines Exercise 8 : (Questions Asked in Previous 13 years Exams)1videos
THE STRAIGHT LINES
- BOOK - ARIHANT MATHSCHAPTER - THREE DIMENSIONAL COORDINATE SYSTEMEXERCISE - Three Dimensional Coordinate System Exercise 12 : Question Asked in Previous Years Exam2videos
THREE DIMENSIONAL COORDINATE SYSTEM
Similar Questions
Column I contain rational algebraic expressions and ColumnII contains possible integers which lie in their range. Match the entries of Column I with one or more entries of the elements of Column II.
02:43View Solution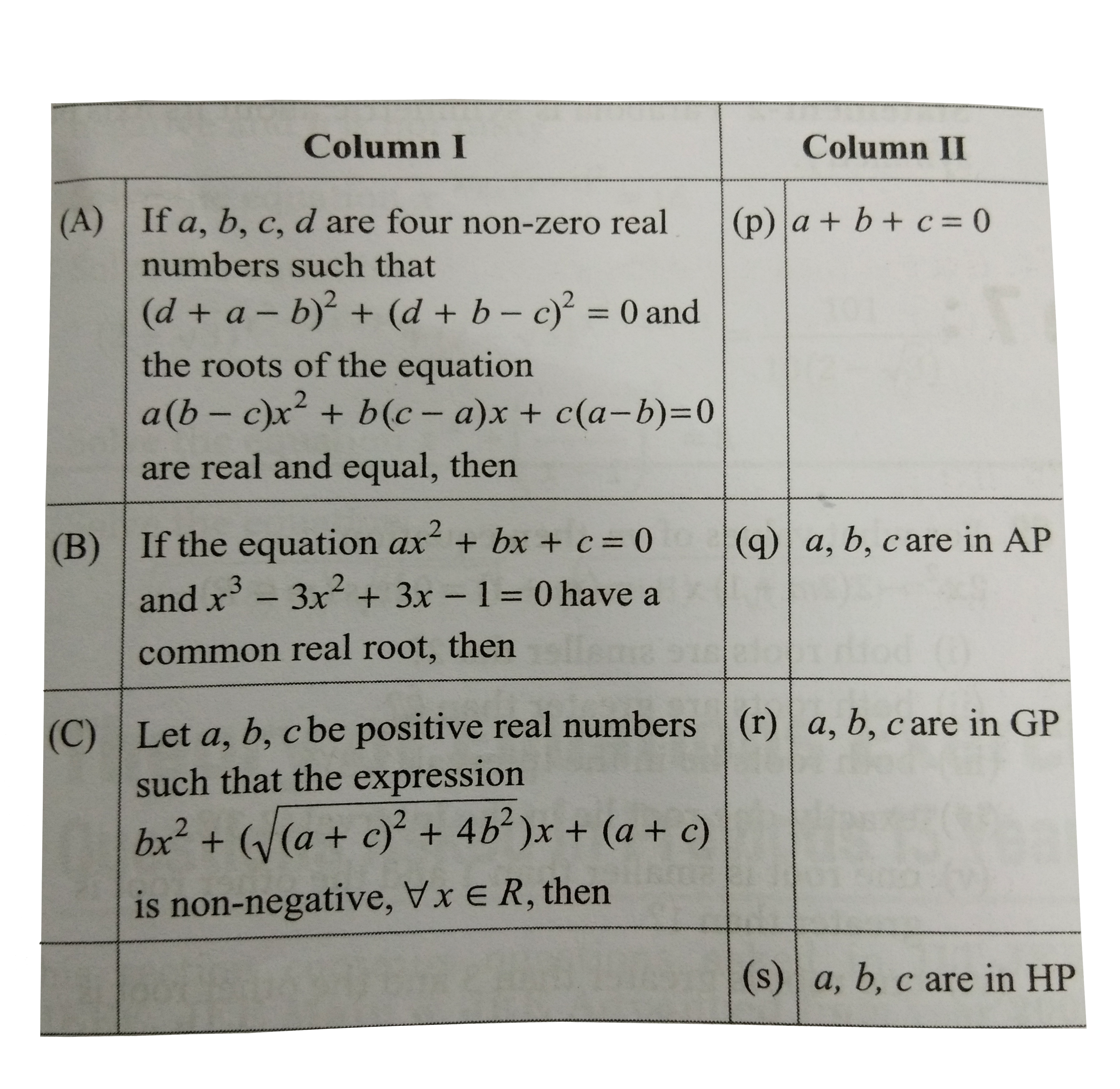
01:59View SolutionIf G and L are the greatest and least values of the expression2x2−3x+22x2+3x+2,xεR respectively.
The least value of G100+L100 is01:21View SolutionSolve the following system of inequalities graphically
x+5y≤25,2x+2y≥2,2x−2y≤0,x≥0,y≥005:13View SolutionConsider the line L1:x−12=y−1=z+31,L2:x−41=y+31=z+32 find the angle between them.
02:35View Solution(1+i)(1+2i)(1+3i)............(1+ni)=alpha+i beta, then the value of 2.5.10......(1+n^(2))=alpha^(2)+beta^(2)
02:20View SolutionIf alpha and beta are the roots of equation x^(2)-a(x+1)-b=0 where a,beR-{0} and a+b!=0 then the value of (1)/(alpha^(2)-a alpha)+(1)/(beta^(2)-a beta)-(2)/(a+b)
05:28View SolutionIf α,β , are roots of the equation x2+√xα+β=0,ββ then values of αandβ are 1.α=1andβ=1 2. α=1andβ=−2 3. α=2andβ=1 α=2andβ=−2
02:44View SolutionIf alpha,beta are roots of the equation x^(2)-px+q=0, then find the value of (i)alpha^(2)(alpha^(2)beta^(-1)-beta)+beta^(2)(beta^(2)alpha^(-1)-alpha)
03:46View SolutionIf alpha,beta are the roots of x^(2)-2x+4=0 ,then (alpha)/(beta) equal to (1)/(2)(-1+-sqrt(3)i) .
04:43View SolutionIf α,β are roots of the equation x2+x+1=0, then the equation whose roots are αβandβα, is
03:53View SolutionIf α and β(α>β) are roots of the equation x2−√2x+√3−2√2=0, then the value of (cos−1α+tan−1α+tan−1β) is equal to
02:26View SolutionIf α and β are the roots of equation x2−4x+1=0, find (i) α2+β2 (ii) α3+β3
05:07View SolutionIf 1∈(α,β) where α,β are the roots of the equation x2−a(x+1)+3=0, then
04:04View SolutionIf the roots αandβ of the equation , x2−√2x+c=0 are complex for some real number c≠1 and ∣∣∣α−β1−αβ∣∣∣ = 1, then a value of c is :
03:46View SolutionIf α,β are the roots of the equation x2+x+1=0, then the equation whose roots are αβ and βα is
03:13View Solution