If α−β= constant, then the locus of the point of intersection of tangents at P(acosα,bsinα) and Q(acosβ,bsinβ) to the ellipse x2a2+y2b2=1 is a circle (b) a straight line an ellipse (d) a parabolaSolution in Bengali
a cricle
a straigth line
an ellipse
a parabola
Tangents to the ellipse at P and Q are, respectively,
xacosα+ybsinα=
andxacosβ+ybsinβ=1
Solving, (1) and (2) , we get
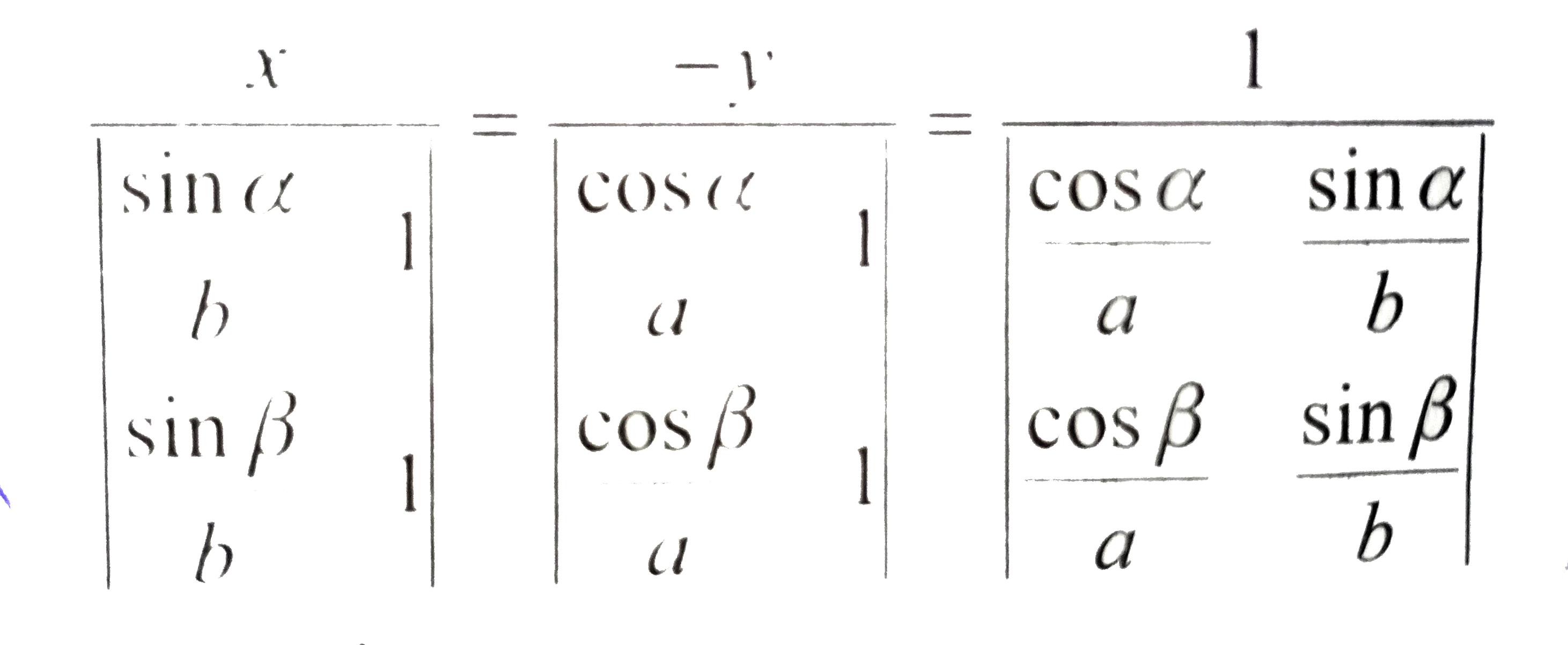
or x=a(sinα−sinβ)sin(β−α)
and y=−b(cosα−cosβ)sin(β−α)
and=−xsin(β−α)a=sinα−sinβ
y=−ysin(β−α)a=(cosα−cosβ)
Saqring and adding. we get
sin2(β−α)(x2a2+y2b2)=2
x2a2+y2b2=2sin2c [ where β−α=c (constant) given]
which is an ellipse
Similar Questions
If α−β= constant, then the locus of the point of intersection of tangents at P(acosα,bsinα) and Q(acosβ,bsinβ) to the ellipse x2a2+y2b2=1 is: (a) a circle (b) a straight line (c) an ellipse (d) a parabola
View SolutionThe locus of the point of intersection of the tangent at the endpoints of the focal chord of the ellipse x2a2+y2b2=1(b<a)(a) is a an circle (b) ellipse (c) hyperbola (d) pair of straight lines
View SolutionIf a tangent to the parabola y2=4ax intersects the x2a2+y2b2=1 at Aand B, then the locus of the point of intersection of tangents at A and B to the ellipse is
View SolutionIf xacosα+ybsinα=1,xacosβ+ybsinβ=1andcosαcosβa2+sinαsinβb2=0, then
View SolutionThe locus of the poles of the tangents to the ellipse x2a2+y2b2=1 w.r.t. the circle x2+y2=a2 is: (a) parabola (b) ellipse (c) hyperbola (d) circle
View SolutionIf xα+yβ=1 touches the circle x2+y2=a2 then point (1α,1β) lies on (a) straight line (b) circle (c) parabola (d) ellipse
View SolutionIf xα+yβ=1 touches the circle x2+y2=a2 then point (1α,1β) lies on (a) straight line (b) circle (c) parabola (d) ellipse
View SolutionIf alpha-beta= constant, then the locus of the point of intersection of tangents at P(acosalpha,bsinalpha) and Q(acosbeta,bsinbeta) to the ellipse (x^2)/(a^2)+(y^2)/(b^2)=1 is: (a) a circle (b) a straight line (c) an ellipse (d) a parabola
View Solution
