If (b2−b1)(b3−b1)+(a2−a1)(a3−a1)=0 , then prove that the circumcenter of the triangle having vertices (a1,b1),(a2,b2) and (a3,b3) is (a2+a32,b2+b32)Solution in Bengali
Given (b2−b1)(b3−b1)+(a2−a1)(a3−a1)=0
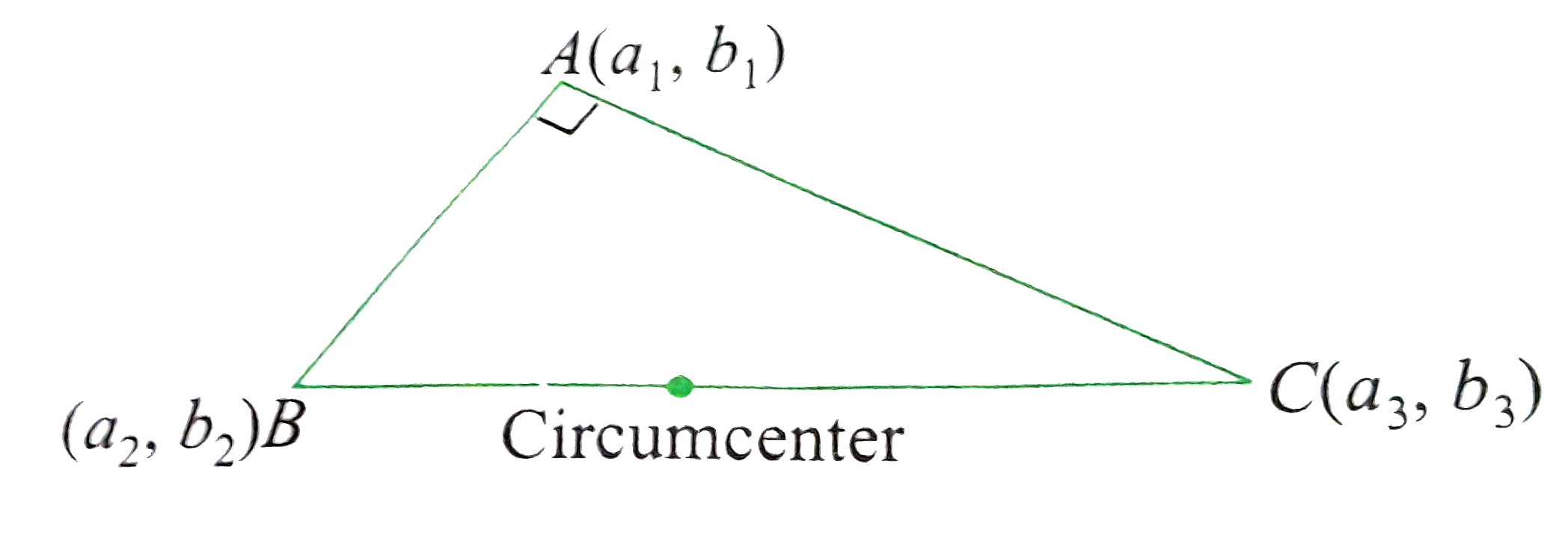
or (b2−b1)(b3−b1)=−(a2−a1)(a3−a1)
or (b2−b1a2−a1)(b3−b1a3−a1)=−1
or (slope of AB)×(slope of AC)=−1
Therefore, the triangle is right -angled at point (a1,b2). Hence, the circumcenter is the midpoint of (a2b2) and (a3,b3) which is (a2+a32,b2+b32)
Topper's Solved these Questions
- COORDINATE SYSYEM BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Illustration1.48
1 Videos
- COORDINATE SYSYEM BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Illustration1.49
1 Videos
- COORDINATE SYSYEM BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Illustration1.46
1 Videos
- COORDINATE SYSTEMBOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type
2 Videos
- CROSS PRODUCTS BOOK - CENGAGE PUBLICATIONCHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.2
13 Videos
Similar Questions
Find the orthocentre of ABC with vertices A(1,0),B(−2,1), and C(5,2)
View SolutionTwo medians drawn from the acute angles of a right angled triangle intersect at an angle π6. If the length of the hypotenuse of the triangle is 3units, then the area of the triangle (in sq. units) is √3 (b) 3 (c) √2 (d) 9
View SolutionIf A(2,−1)andB(6,5) are two points, then find the ratio in which the foot of the perpendicular from (4,1) to AB divides it.
View SolutionCoordinates of points on curve 5x2−6xy+5y2−4=0 which are nearest to origin are
View SolutionLet →a and →b be two vectors of equal magnitude 5 units. Let →p,→q be vectors such that →p=→a−→b and →q=→a+→b. If |→p×→q|=2{λ−(→a.→b)2}12, then value of λ is
View SolutionThe determinant |(b_1+c_1,c_1+a_1,a_1+b_1),(b_2+c_2,c_2+a_2,a_2+b_2),(b_3+c_3,c_3+a_3,a_3+b_3)|=
View SolutionIf Delta=|[a_1,b_1,c_1],[a_2,b_2,c_2],[a_3,b_3,c_3]| and A_1,B_1,C_1 denote the co-factors of a_1, b_1, c_1 respectively, then the value of the determinant |[A_1,B_1,C_1],[A_2,B_2,C_2],[A_3,B_3,C_3]| is-
View SolutionIf A_1, B_1, C_1,... are respectively the co-factors of the elements a_1,b_1, c_1,... of the determinant Delta=|[a_1, b_1,c_1] , [a_2, b_2, c_2] , [a_3, b_3,c_3]| then |[B_2,C_2] , [B_3,C_3]|=
View SolutionIf (b2−b1)(b3−b−1)+(a2−a1)(a3−a1)=0, then prove that the circumcenter of the triangle having vertices (a1,b1),(a2,b2) and (a3,b3) is (a2+a32,b2+b32).
View SolutionLet a1,a2,a3 ….and b1,b2,b3… be two geometric progressions with a1=2√3 and b1=529√3 If 3a99b99=104 then find the value of a1b1+a2b2+…+anbn
View SolutionSuppose four distinct positive numbers a1,a2,a3,a4 are in G.P. Let b1=a1,b2=b1+a2,b3=b2+a3 and b4=b3+a4.
Statement -1 : The numbers b1,b2,b4 are neither in A.P. nor in G.P. and
Statement -2 : The numbers b1,b2,b3,b4 are in H.P.
View Solutionसिद्ध कीजिए- Δ=∣∣ ∣∣a1a2a3b1b2b3Ka1Ka2Ka3∣∣ ∣∣=0
(पंक्तियाँ R2 व R3 समानुपाती है )
View Solutionযে কোনো তিনটি সেট A1,A2,A3-এর B1=A1,B2=A2−A1এবং B3=A3−(A1∪A2) হলে, নীচের কোন বিবৃতিটি সর্বদাই সত্য
View SolutionIf (b2−b1)(b3−b1)+(a2−a1)(a3−a1)=0 , then prove that the circumcenter of the triangle having vertices (a1,b1),(a2,b2) and (a3,b3) is (a2+a32,b2+b32)
View SolutionIf (b2−b1)(b3−b1)+(a2−a1)(a3−a1)=0, then prove that the circumcenter of the triangle having vertices (a1,b1),(a2,b2) and (a3,b3) is (a2+a32,b2+b32).
View SolutionLet a1,a2,a3 ….and b1,b2,b3… be two geometric progressions with a1=2√3 and b1=529√3 If 3a99b99=104 then find the value of a1b1+a2b2+…+anbn
View Solution
