If coefficient of correlation between two variables X and Y is 0.64,cov(X,Y)=16 and Var(X)=9, then the standard deviation of Y series is
1.2
8.33
24
25
The correct Answer is:B
To find the standard deviation of the Y series given the coefficient of correlation, covariance, and variance of X, we can follow these steps:
Step 1: Identify the given values
- Coefficient of correlation (r) = 0.64
- Covariance (cov(X, Y)) = 16
- Variance of X (Var(X)) = 9
Step 2: Calculate the standard deviation of X
The standard deviation (SD) of X can be calculated using the formula:
SD(X)=√Var(X)
Substituting the given variance:
SD(X)=√9=3
Step 3: Use the formula for the coefficient of correlation
The formula for the coefficient of correlation is:
r=cov(X,Y)SD(X)×SD(Y)
We can rearrange this formula to solve for the standard deviation of Y (SD(Y)):
SD(Y)=cov(X,Y)r×SD(X)
Step 4: Substitute the known values into the formula
Now, substituting the values we have:
SD(Y)=160.64×3
Step 5: Calculate SD(Y)
First, calculate the denominator:
0.64×3=1.92
Now substitute this back into the equation:
SD(Y)=161.92
Calculating this gives:
SD(Y)≈8.33
Final Result
Thus, the standard deviation of the Y series is approximately 253 or 8.33.
---
- Coefficient of correlation (r) = 0.64
- Covariance (cov(X, Y)) = 16
- Variance of X (Var(X)) = 9
The standard deviation (SD) of X can be calculated using the formula:
SD(X)=√Var(X)
Substituting the given variance:
SD(X)=√9=3
The formula for the coefficient of correlation is:
r=cov(X,Y)SD(X)×SD(Y)
We can rearrange this formula to solve for the standard deviation of Y (SD(Y)):
SD(Y)=cov(X,Y)r×SD(X)
Now, substituting the values we have:
SD(Y)=160.64×3
First, calculate the denominator:
0.64×3=1.92
Now substitute this back into the equation:
SD(Y)=161.92
Calculating this gives:
SD(Y)≈8.33
Thus, the standard deviation of the Y series is approximately 253 or 8.33.
Topper's Solved these Questions
Similar Questions
The coefficient of correlation between the values denoted by X and Y is 0.5. The mean of X is 3 and that of Y is 5. Their standard deviations are 5 and 4 respectively. Find
(i) the two lines of regression.
(ii) the expected value of Y, when X is given 14
(iii) the expected value of when Y is given 9
View SolutionThe coefficient of correlation between the values denoted by x and y is 0.5. The standard deviation of x is 5 and that of y is 4. Find the angle between the lnes of regression.
View Solution
Knowledge Check
If coefficient of correlation is -0.6, standard deviation of x is 5 and variance of y is 16, then what is the co-variance of x and y?
A0.75B0.48C-0.03D-0.75IF cov(X,Y)=−8,Var(X)=1.44 and Var(Y)=100, then coefficient of correlation between X and Y is
A23B34C−34D−23
The coefficient of correlation between X and Y is 0.6 U and V are two variables defined as U=x−32,V=y−23, then the coefficient of correlation between U and V is
View SolutionFind the coefficient of correlation, when Cov(x, y) = -16.5, Var(x)= 100, Var(y)=2.89.
View SolutionFind the Karl Pearson's coefficient of correlation between X and Y for the following data :
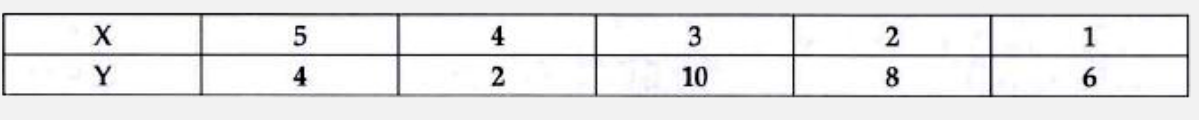
View SolutionCaculate Karl Pearson 's coefficient of correlation between X and Y from the following data :
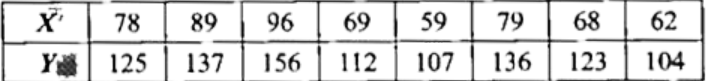
Assume 80 and 130 as the mean values for X and Y respectively .
View SolutionYou are given the following results on two variables X and Y:
¯x=36,¯y=85,σx=11, Var (Y) = 64 and r (X, Y) = 0.66. Find the regression equations and estimate the value of x when y= 75.
View SolutionFind the cov (X, Y) between X and Y, if ∑u1v1=55andn=11, where u1andv1 are deviation of X and Y series from their respective means.
View Solution
