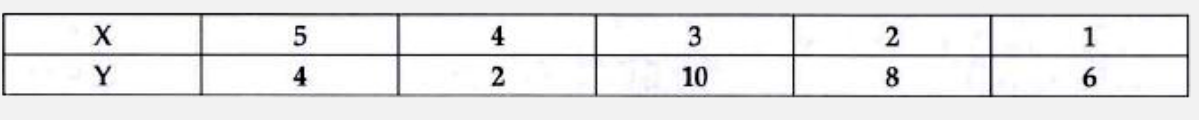
IF cov(X,Y)=−8,Var(X)=1.44 and Var(Y)=100, then coefficient of correlation between X and Y is
23
34
−34
−23
The correct Answer is:D
To find the coefficient of correlation between X and Y, we can use the formula:
r=cov(X,Y)√Var(X)⋅Var(Y)
1. Identify the given values:
- Covariance cov(X,Y)=−8
- Variance Var(X)=1.44
- Variance Var(Y)=100
2. Substitute the values into the formula:
r=−8√1.44⋅100
3. Calculate the product of the variances:
1.44⋅100=144
4. Calculate the square root of the product:
√144=12
5. Substitute back into the formula:
r=−812
6. Simplify the fraction:
r=−23
Final Answer:
The coefficient of correlation r between X and Y is −23.
---
- Covariance cov(X,Y)=−8
- Variance Var(X)=1.44
- Variance Var(Y)=100
r=−8√1.44⋅100
1.44⋅100=144
√144=12
r=−812
r=−23
The coefficient of correlation r between X and Y is −23.
Topper's Solved these Questions
Similar Questions
Knowledge Check
If cov(X,Y)=16.5,Var(X)=8.25 and Var(Y)=33, then Karl Pearson's coefficient of correlation between X and Y is
A12B23C34D1If the regression equation of y on x is −2x+5y=14 and the regression equation of x on y is given by mx−y+10=0. If the coefficient of correlation between x and y is 1√10, then the value of m is
A6B4C8D2If coefficient of correlation between two variables X and Y is 0.64,cov(X,Y)=16 and Var(X)=9, then the standard deviation of Y series is
A1.2B8.33C24D25
By using the data ¯x=25,¯y=30,byx=1.6andbxy=0.4 find
(a) The regression equation y on x.
(b) What is the most likely value of y when x=60?
(c ) What is the coefficient of correlation between x and y ?
View SolutionFind the coefficient of x6y3 in (x+y)9
View SolutionFor 50 students of a class, regression equation of marks in statistics (x) on marks in Accountancy (y) in 3y−5x+180=0. The mean in Accoutancy is 44 and the variance of marks in statics is 9/16 of the varience of marks in Accountancy. Find the mean marks in Statistics and coefficient of correlation between marks in two subjects.
View SolutionFind r(x,y) if Cov (x,y) = -165 , Var( x)= 2.25 and Var(y)= 144.
View SolutionCaculate Karl Pearson 's coefficient of correlation between X and Y from the following data :
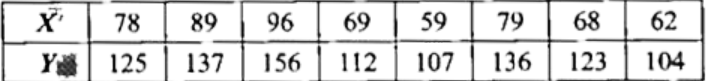
Assume 80 and 130 as the mean values for X and Y respectively .
View Solution