If each diagonals of a quadrilateral separates it into two triangles of equal area then show that the quadrilateral is a parallelogram.
GIVEN A quad. ABCD in which diagonals AC and BD are such that
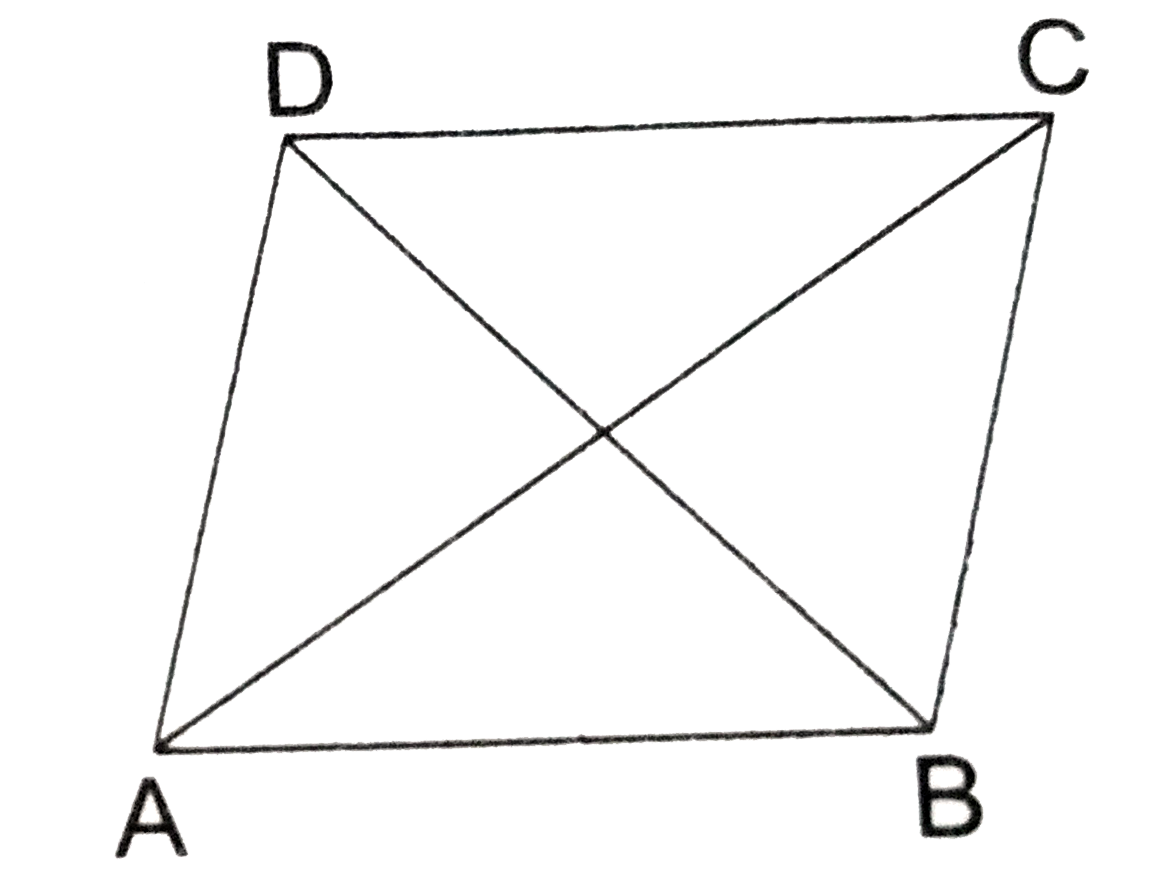
ar(△ABD)=ar(△CDB)andar(△ABC)=ar(△ACD).
TO PROVE ABCD is a parallelogram.
PROOF ar(quad. ABCD)=ar(△ABC)+ar(△ACD)
=2ar(△ABC) [∴(△ACD)=ar(△ABC)].
∴ar(△ABC)=12ar(quad. ABCD). ...(i)
Again, ar(quad. ABCD)=ar(△ABD)+ar(△CDB)=2ar(△ABD)
[∴ar(△CDB)=ar(△ABD)].
∴ar(△ABD)=12ar(quad. ABCD). ...(ii)
From (i) and (ii), we get
ar(△ABC)=ar(△ABD). Also, △ABCand△ABD have the same base AB.
∴ they lie between the same parallels AB and DC [ Theorem 9 ]
i.e., AB||DC.
Similarly, AD||BC.
Hence, ABCD is a ||gm.
Topper's Solved these Questions
- AREAS OF PARALLELOGRAMS AND TRIANGLES BOOK - RS AGGARWALCHAPTER - AREAS OF PARALLELOGRAMS AND TRIANGLES EXERCISE - Exercise 11
38 Videos
- AREAS OF PARALLELOGRAMS AND TRIANGLES BOOK - RS AGGARWALCHAPTER - AREAS OF PARALLELOGRAMS AND TRIANGLES EXERCISE - MULTIPLE-CHOICE QUESTIONS (MCQ)
32 Videos
- AREAS OF TRIANGLES AND QUADRILATERALSBOOK - RS AGGARWALCHAPTER - AREAS OF TRIANGLES AND QUADRILATERALSEXERCISE - Multiple Choice Questions (Mcq)
16 Videos
Similar Questions
Prove that If each diagonal of a quadrilateral separates it into two triangles of equal area then show that the quadrilateral is a parallelogram.
View SolutionA diagonal of a parallelogram divides it into two triangles of equal area.
View SolutionIf both the diagonals of a quadrilateral divides it into four triangles of equal area, then the quadrilateral is a rhombus.
View SolutionIf the diagonals AC, BD of a quadrilateral ABCD, intersect at O, and separate the quadrilateral into four triangles of equal area, show that quadrilateral ABCD is a parallelogram.
View SolutionIf the diagonals AC,BD of a quadrilateral ABCD, intersect at O, and seqarate the quadrilateral into four triangles of equal area, show that quadrilateral ABCD is a parallelogram. GIVEN : A quadrilateral ABCD such that its diagonals AC and BD intersect at O and separate it into four parts such that ar(AOB)=ar(BOC)=ar(COD)=ar(AOD) TO PROVE : Quadrilateral ABCD is a parallelogram.
View SolutionShow that the diagonals of a parallelogram divide it into four triangles of equal area.
View SolutionShow that the diagonals of a parallelogram divide it into four triangles of equal area.
View SolutionShow that the diagonals of a parallelogram divide it into four triangles of equal area.
View SolutionIf the diagonals of a quadrilateral bisect each other, then the quadrilateral is a parallelogram.
View SolutionIf the opposite sides of a quadrilateral are equal, prove that the quadrilateral is a parallelogram.
View Solution
