If from an external point B of a circle with centre O, two tangents BC and BD are drawn such that ∠DBC=120∘, prove that BC+BD=BO.
To prove that BC+BD=BO given that ∠DBC=120∘, we can follow these steps:
Step 1: Understand the Geometry
We have a circle with center O and an external point B from which two tangents BC and BD are drawn to the circle. By the properties of tangents, we know that BC=BD.
Step 2: Identify Angles
Given ∠DBC=120∘, we can denote ∠CBA (the angle between the two tangents at point B) as 120∘. Therefore, the angle ∠ABC can be calculated as:
∠ABC=180∘−∠DBC=180∘−120∘=60∘
Step 3: Use Right Triangles
Since OC and OD are radii of the circle and are perpendicular to the tangents at points C and D respectively, we have:
∠OCB=90∘and∠ODB=90∘
Step 4: Apply Cosine Rule in Triangle OBC
In triangle OBC, we can use the cosine rule. We know:
cos(60∘)=BCOB
Since cos(60∘)=12, we can write:
12=BCOB⟹OB=2×BC
Step 5: Relate BC and BD
Since BC=BD (the lengths of tangents from an external point to a circle are equal), we can write:
OB=2×BC=2×BD
Step 6: Combine the Lengths
Now, we can express BC+BD:
BC+BD=BC+BC=2×BC
Thus, we have:
BC+BD=OB
Conclusion
We have proved that:
BC+BD=BO
We have a circle with center O and an external point B from which two tangents BC and BD are drawn to the circle. By the properties of tangents, we know that BC=BD.
Given ∠DBC=120∘, we can denote ∠CBA (the angle between the two tangents at point B) as 120∘. Therefore, the angle ∠ABC can be calculated as:
∠ABC=180∘−∠DBC=180∘−120∘=60∘
Since OC and OD are radii of the circle and are perpendicular to the tangents at points C and D respectively, we have:
∠OCB=90∘and∠ODB=90∘
In triangle OBC, we can use the cosine rule. We know:
cos(60∘)=BCOB
Since cos(60∘)=12, we can write:
12=BCOB⟹OB=2×BC
Since BC=BD (the lengths of tangents from an external point to a circle are equal), we can write:
OB=2×BC=2×BD
Now, we can express BC+BD:
BC+BD=BC+BC=2×BC
Thus, we have:
BC+BD=OB
We have proved that:
BC+BD=BO
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CIRCLESEXERCISE - Problems From NCERT/exemplar11videos
CIRCLES
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CIRCLESEXERCISE - Exercise32videos
CIRCLES
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - ARITHMETIC PROGRESSIONEXERCISE - Revision Exercise Long Answer Question5videos
ARITHMETIC PROGRESSION
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - Revision Exercise Short Answer Questions10videos
CO-ORDINATE GEOMETRY
Similar Questions
If from an extrenal point B of a circle with centre 0, two tangents BC and BD are drawn such that ∠DBC=120∘, prove that BC+BD=BO i.e., BO=2BC.
View SolutionTwo tangent segments BC and BD are drawn to a circle with centre O and radius r such that ∠DBC=120∘. Prove that BO=2BC.
View SolutionTwo tangents TP and TQ are drawn from an external point T to a circle with centre O as shown in figure. If they are inclined to each other at an angle of 100∘, then what is the value of ∠POQ?
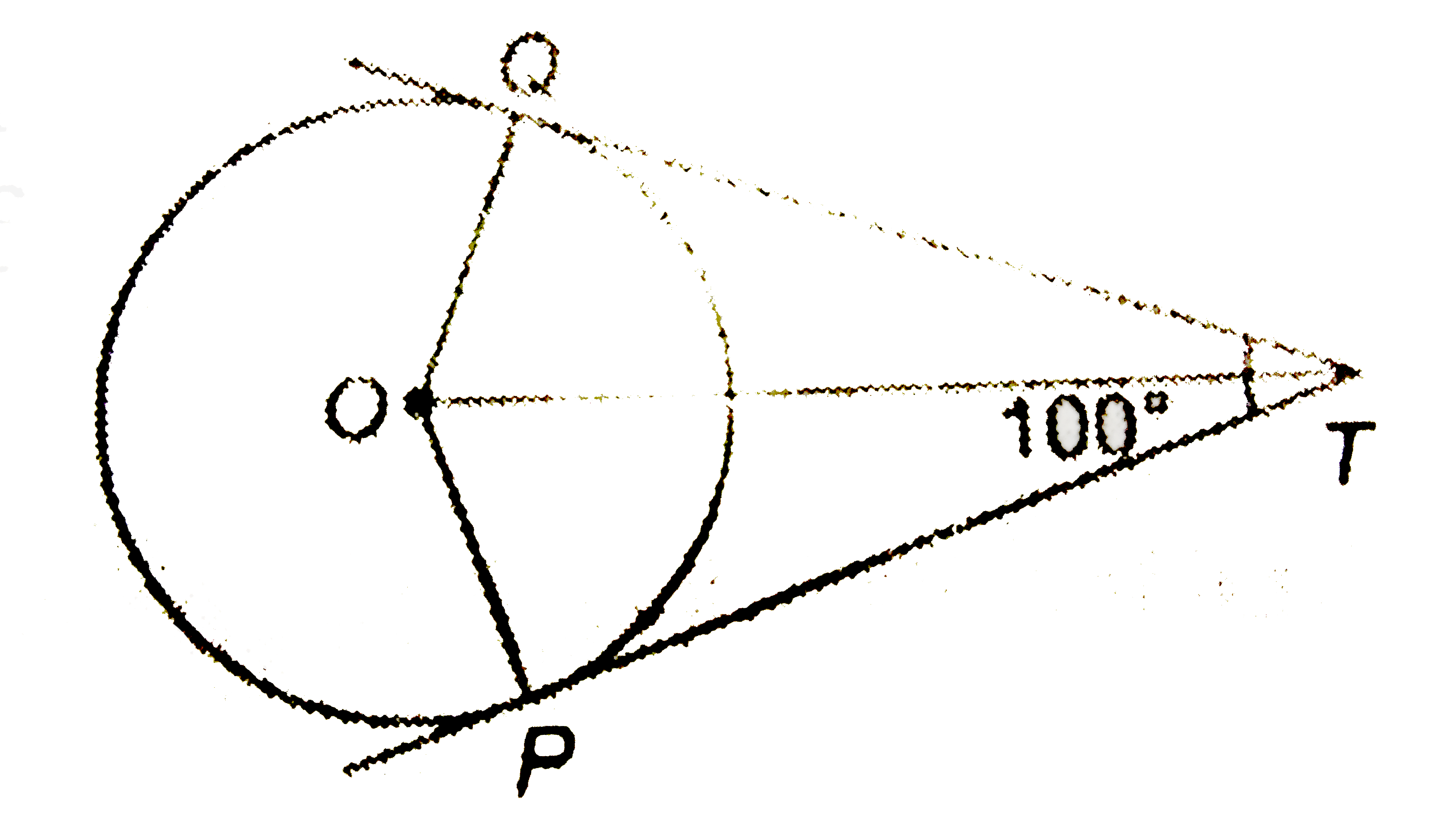 View Solution
View SolutionIn Figure, PA and PB are tangents from an external point P to a circle with centre O . LN touches the circle at M . Prove that PL+LM=PN+MN .
View SolutionA point P lies outside the circle with centre O. two lines PAOB and PDC are drawn on the circle from P. if PD =OD, then prove that arc BC=3×ArcAD.
View SolutionPA and PB are two tangents drawn from an external point P to a circle with centre C and radius=4cm If PA⊥PB then length of each tangent is
View SolutionFrom a point P outside a circle, with centre O, tangents PA and PB are drawn. Prove that
(i) ∠AOP=∠BOP
(ii) OP is the ⊥ bisector of chord AB.View SolutionIf Figure-1, from an external point P, two tangents PQ and PR are drawn to a circle of radius 4 cm with centre O. if ∠QPR=90∘, then length of PQ is
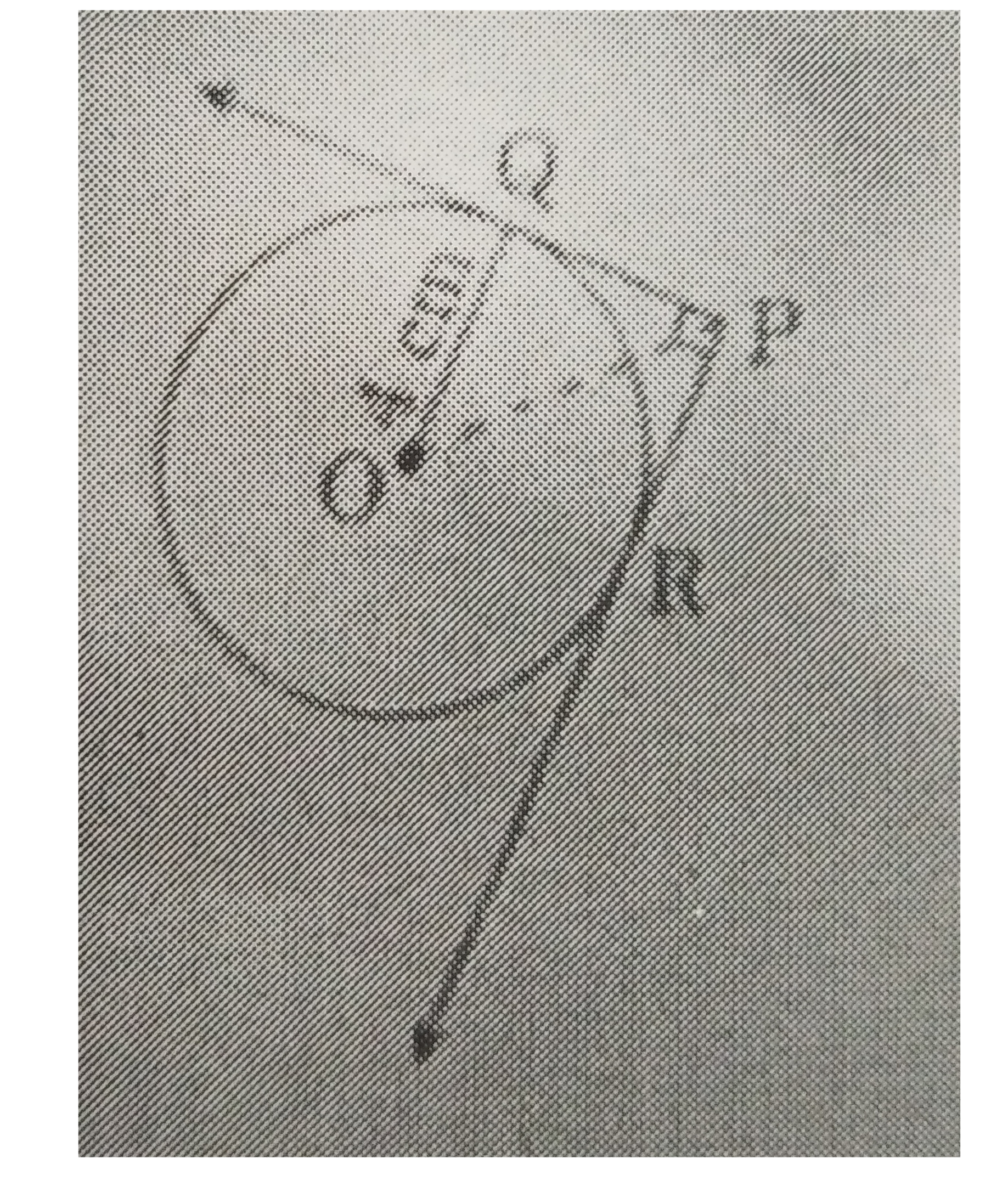 View Solution
View SolutionIn Figure, two tangents ABandAC are drawn to a circle with centre O such that ∠BAC=1200. Prove that OA=2AB
View SolutionIn the given figure, TP and TQ are tangents drawn from an external point T to a circle with centre O such that ∠TQP=60∘. Find angelOPQ.
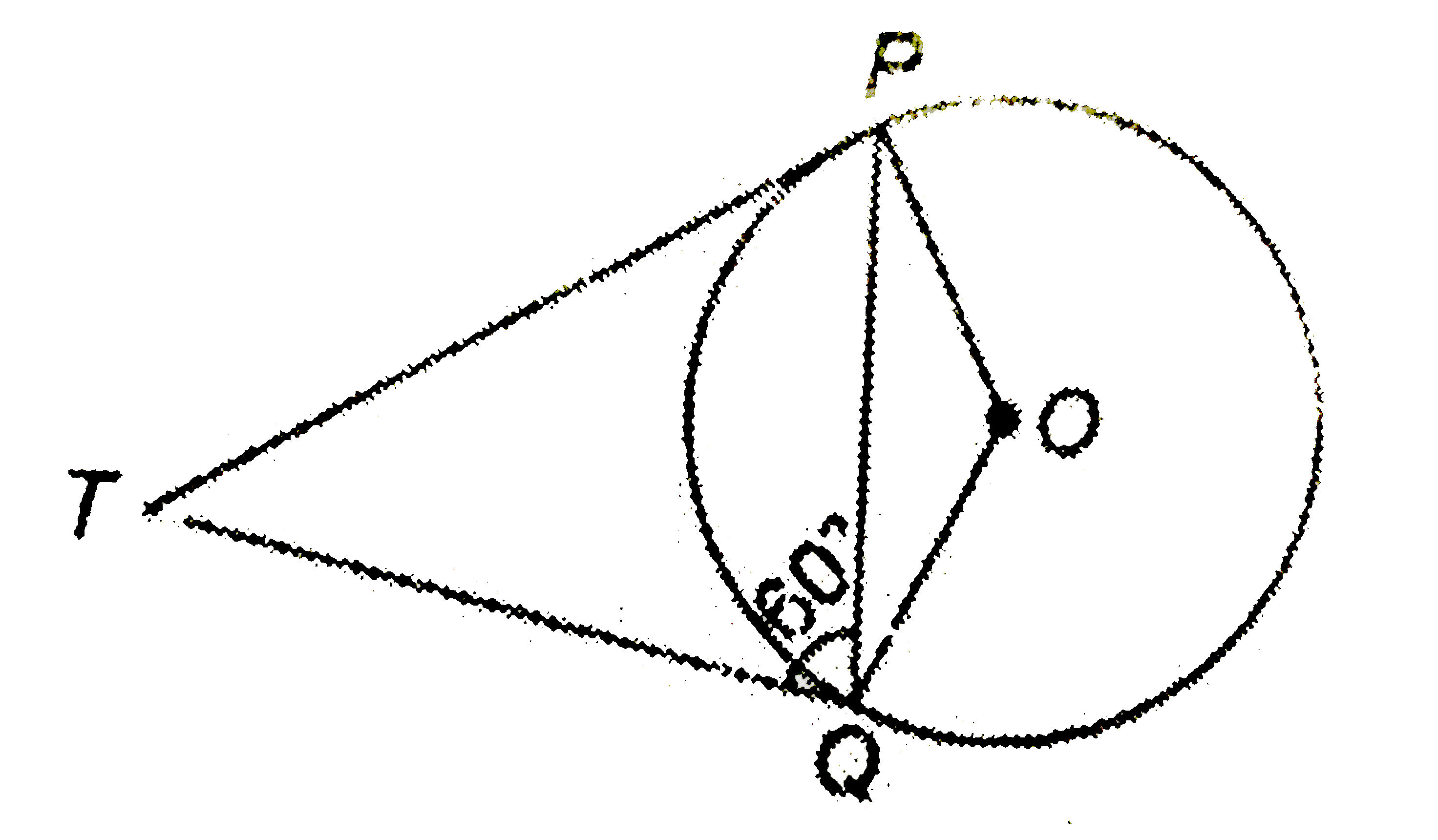 View Solution
View Solution
