If f(x)=x3+bx2+cx+d and 0<b2<c, thenSolution in Punjabi
f(x) is strictly increasing function
f(x) has a local maxima
f(x) is strictly decreasing function
f(x) is bounded
The correct Answer is:A
Step by step video & image solution for If f(x)=x^3+bx^2+cx+d and 0<b^2<c, then by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MONOTONICITY MAXIMA AND MINIMABOOK - ARIHANT MATHSCHAPTER - MONOTONICITY MAXIMA AND MINIMAEXERCISE - MAXIMA AND MINIMA EXERCISE 6
2 Videos
- MATRICES BOOK - ARIHANT MATHSCHAPTER - MATRICES EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
49 Videos
- PAIR OF STRAIGHT LINES BOOK - ARIHANT MATHSCHAPTER - PAIR OF STRAIGHT LINES EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
2 Videos
Similar Questions
The figure shows a right triangle with its hypotenuse OB along the y-axis and its vertex A on the parabola y=x2.
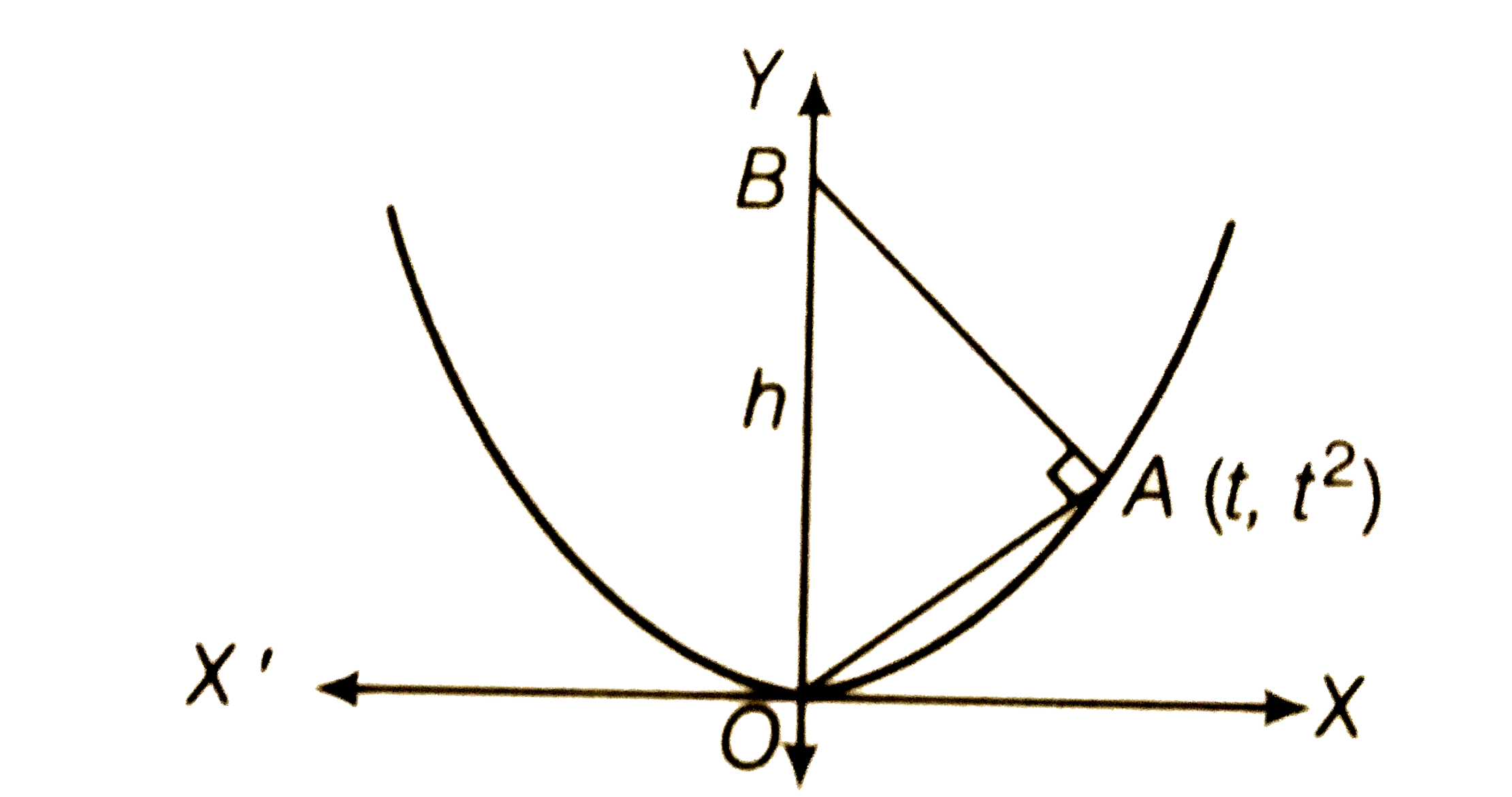
Let h represents the length of the hypotenuse which depends on the x-coordinate of the point A. The value of limt→o(h) is equal to
View SolutionLet A=⎡⎢⎣1000110−24⎤⎥⎦,I=⎡⎢⎣100010001⎤⎥⎦andA−1=[16(A2+cA+dI)] Then value of candd are (a) (−6,−11) (b) (6,11) (c) (−6,11) (d) (6,−11)
View SolutionIf the pair of lines ax2+2(a+b)xy+by2=0 lie along diameters of a circle and divide the circle into four sectors such that the area of one of the sectors is thrice the area of another sector then
View Solutionif x^(2)+x+1 is a factor of ax^(3)+bx^(2)+cx+d then the real root of ax^(3)+bx^(2)+cx+d=0 is : (a) -(d)/(a)(B)(d)/(a) (C) (a)/(b) (D)none of these
View SolutionIf (x2−x+1) is a factor of f(x)=ax3+bx2+cx+d where a≠0 and a,b,c,d are real, then the real root of f(x)=0 is
View SolutionIf f(x)=x3+bx3+cx+dand0<b2<cthen in(−∞,∞)
View SolutionIf (a + bx)/(a - bx) = (b + cx)/(b - cx) = (c + dx)/(c - dx)( x ne 0), then a ,b ,c ,d are in
View SolutionSuppose a, b, c,d be non-zero real number and ab>0, and ∫10(1+ex2)(ax3+bx2+cx+d)dx=∫20(1+ex2)
(ax3+bx2+cx+d)dx=0
If f(x)=∫f(x)dx=∫10f(x)dx=∫20f(x)dx=0
then in which of the following can Rolle's theorem can be applied for F(x), where
f(x)=(1+ex2)(ax3+bx2+cx+d)
View SolutionLet f(x)=x3+bx2+cx+d,0<b2<c. Then f(x)-
View SolutionIf f(x)=x3+bx2+cx+d and 0<b2<c.then in (−∞,∞)
View Solutionयदि f(x)=x3+bx2+cx+d और 0<b2<c, तब (−∞,∞) में
View SolutionFor f(x)=x3+bx2+cx+d, if b2>4c>0 and b,c,d∈R, then f(x)
View Solution( a+bx) /( a-bx) = ( b+cx)/( b-cx) = ( c +dx)/( c-dx), ( x cancel(=)0) then a,b,c,d are in :
View SolutionIf f(x)=x^(3)+bx^(2)+cx+d and 0 lt b^(2) lt c , then in (-oo, oo), f(x) :
View Solutionমনে করো f(x)=x^3+bx^2+cx+d, 0
View Solutionयदि f(x)=x3+bx2+cx+d,0<b2<c है, तो f(x) -
View Solution
