If in a series which is not highly skewed the mean deviation is 7⋅8, the approximate value of its standard deviation is
9⋅75
8⋅37
10⋅2
none of these
The correct Answer is:A
To find the approximate value of the standard deviation given that the mean deviation is 7.8 in a moderately skewed distribution, we can follow these steps:
Step 1: Understand the relationship between Mean Deviation and Standard Deviation
For a moderately skewed distribution, the relationship between the mean deviation (MD) and the standard deviation (SD) is given by the formula:
MD=45×SD
Step 2: Rearrange the formula to find Standard Deviation
We can rearrange the formula to express the standard deviation in terms of the mean deviation:
SD=54×MD
Step 3: Substitute the value of Mean Deviation
Now, we substitute the given value of mean deviation (MD = 7.8) into the equation:
SD=54×7.8
Step 4: Calculate the Standard Deviation
Now, we perform the multiplication:
SD=5×7.84=394=9.75
Step 5: Final Calculation
To express it in decimal form:
SD=9.75
Thus, the approximate value of the standard deviation is 9.75.
For a moderately skewed distribution, the relationship between the mean deviation (MD) and the standard deviation (SD) is given by the formula:
MD=45×SD
We can rearrange the formula to express the standard deviation in terms of the mean deviation:
SD=54×MD
Now, we substitute the given value of mean deviation (MD = 7.8) into the equation:
SD=54×7.8
Now, we perform the multiplication:
SD=5×7.84=394=9.75
To express it in decimal form:
SD=9.75
Topper's Solved these Questions
- MEASURES OF CENTRAL TENDENCY BOOK - ML KHANNACHAPTER - MEASURES OF CENTRAL TENDENCY EXERCISE - SELF ASSESSMENT TEST
5 Videos
- MEASURES OF CENTRAL TENDENCY BOOK - ML KHANNACHAPTER - MEASURES OF CENTRAL TENDENCY EXERCISE - ASSERTION / REASON
1 Videos
- MEASURES OF CENTRAL TENDENCY BOOK - ML KHANNACHAPTER - MEASURES OF CENTRAL TENDENCY EXERCISE - Problem Set (1) (Measures of Central Tendency)
25 Videos
- MAXIMA AND MINIMA BOOK - ML KHANNACHAPTER - MAXIMA AND MINIMA EXERCISE - MISCELANEOUS EXERCISE (COMPREHENSION)
3 Videos
- NUMERICAL METHODSBOOK - ML KHANNACHAPTER - NUMERICAL METHODSEXERCISE - SELF ASSESSMENT TEST
10 Videos
Similar Questions
Knowledge Check
If mean deviation is 12 the value of standard deviation will be-
A15B12C24DNone of theseFor a series the value of men deviation is 15. The most likely value of its quartile deviation is -
A12⋅5B11⋅6C13D9⋅7For a series the value of men deviation is 15. The most likely value of its quartile deviation is -
A12.5B11.6C13D9.7
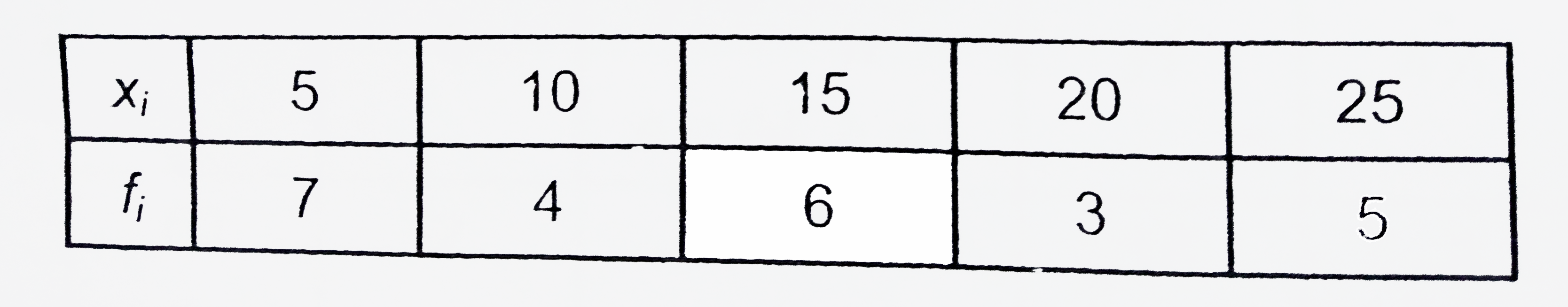
Find the mean , mean deviation about the mean , variance and standard deviation for the following data
View SolutionWhich of these is the merit of standard deviation?
View SolutionThe standard deviationof a variable x is 10.Then the standard deviation of 50+5x is :
View SolutionThe standard deviation of a variable x is 10. Then , the standard deviation of 50+5x is
View SolutionThe standard deviation of 25 numbers is 40. If each of the numbers in increased by 5, then the new standerd deviation will be -
View Solution
