If is the line whose equation is ax+by=c. Let M be the reflection of 'L through the y-axis and let N be the reflection of L through the x-axis. Which of the following must be true about M and N for choices of a,b and c?
The x- intercepts of M and N are equal
The y- intercepts of M and N are equal
The slopes of M and N are equal
The slopes of M and N are reciprocal
The correct Answer is:C
Step by step video, text & image solution for If is the line whose equation is ax +by=c. Let M be the reflection of 'L through the y-axis and let N be the reflection of L through the x-axis. Which of the following must be true about M and N for choices of a, b and c? by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- STRAIGHT LINES BOOK - VIKAS GUPTA (BLACK BOOK)CHAPTER - STRAIGHT LINES EXERCISE - Exercise-2 : One or More than One Answer is/are Correct
12 Videos
- STRAIGHT LINES BOOK - VIKAS GUPTA (BLACK BOOK)CHAPTER - STRAIGHT LINES EXERCISE - Exercise-3 : Comprehension Type Problems
4 Videos
- SOLUTION OF TRIANGLESBOOK - VIKAS GUPTA (BLACK BOOK)CHAPTER - SOLUTION OF TRIANGLESEXERCISE - Exercise-5 : Subjective Type Problems
12 Videos
- TRIGONOMETRIC EQUATIONS BOOK - VIKAS GUPTA (BLACK BOOK)CHAPTER - TRIGONOMETRIC EQUATIONS EXERCISE - Exercise-5 : Subjective Type Problems
9 Videos
Similar Questions
If C is the reflection of A(2,4) in x-axis and B is the reflection of C in y-axis then |AB| is 4√k then k=
View SolutionLet P be the point (3, 2). Let Q be the reflection of P about the x-axis. Let R be the reflection of Q about the line y=−x and let S be the reflection of R through the origin, PQRS is a convex quadrilateral . Find the area of PQRS.
View Solution
Knowledge Check
If C is the reflection of A (2,4) in X-axis and B is the reflection of C in Y-axis, then |AB| is equal to
A20B2√5C4√5D4Which of the following transformations is equivalent to get reflection through x -axis
A(XY)=(cos2θsin2θ−sin2θ−cos2θ)(xy),θ=45∘B(XY)=(cos2θsin2θ−sin2θ−cos2θ)(xy),θ=0∘C(XY)=(sin2θ2θcos2θ−sin2θ)(xy),θ=90∘Dnone of theseThe line segment shown in the figure is reflected through x - axis. The equation of reflected line segment will be
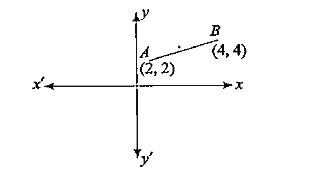 Ay = xBy = x + 2Cy = - xDnone of these
Ay = xBy = x + 2Cy = - xDnone of these
Let m,n are integers with 0<n<m. A is the point (m,n) on the Cartesian plane. B is the reflection of A in the line y=x. C is the reflection of B in the y-axis, D is the reflection of C in the x-axis and E is the reflection of D in the y-axis. The area of the pentagon ABCDE is 2m(m+n) b. m(m+3n) c. m(2m+3n) d. 2m(m+3n)
View SolutionLet m,n are integers with 0<n<m. A is the point (m,n) on the Cartesian plane. B is the reflection of A in the line y=x. C is the reflection of B in the y-axis. D, is the reflection of C in the x-axis and E is the reflection of D in the y-axis. The area of the pentagon ABCDE is 2m(m+n) (b) m(m+3n) m(2m+3n) (d) 2m(m+3n)
View SolutionA is the point (1,6) on the cartesian plane. B is the reflection of A in the line y=x. C is the reflection of B in the y-axis, D is the reflection of C in the x-axis and E is the reflection of D in the y-axis. The area of the pentagon ABEDC is S then find value of S−50.
View SolutionConsider a point A(m,n) , where m and n are positve intergers. B is the reflection of A in the line y=x, C is the reflaction of B in the y axis, D is the reflection of C in the x axis and E is the reflection of D is the y axis. The area of the pentagon ABCDE is.
View SolutionTriangle ABC is reflected through x - axis as shown in the figure . The coordinates of A' , B' and C' are
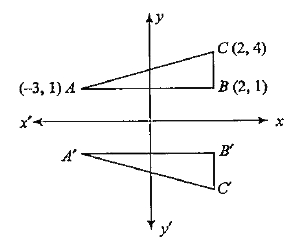
View Solution
