If n>0 and exactly 15 integers satisfying (x+6)(x-4 ) (x-5) (2x−n)≤0 then least possible value of n is Solution in Bengali
a. 10
b. 12
c. 14
d. 16
The correct Answer is:D
(x+6)(x−4)(x−5)(2x−n)≤0
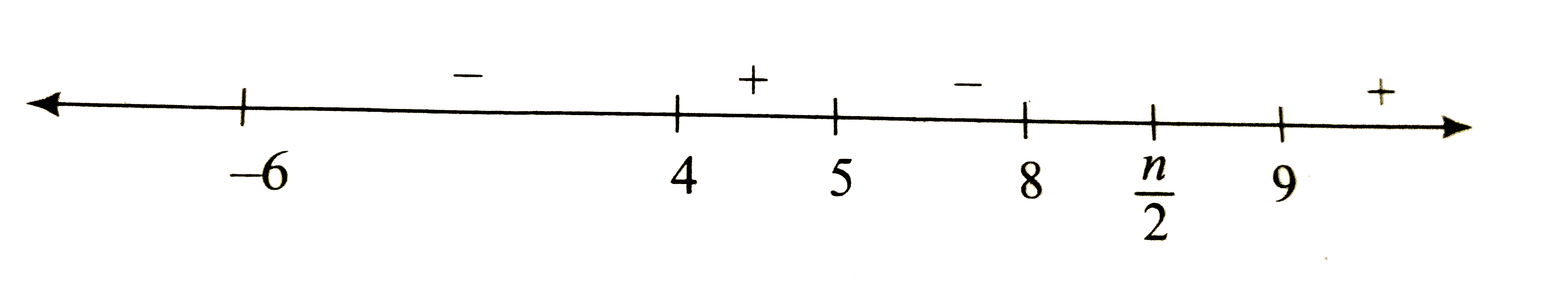
Hence , x∈[−6,4]∪[5,n2]
so n2∈[8,9)
⇒n∈[16,18)
Topper's Solved these Questions
- SET THEORY AND REAL NUMBER SYSTEM BOOK - CENGAGE PUBLICATIONCHAPTER - SET THEORY AND REAL NUMBER SYSTEM EXERCISE - Archives
1 Videos
- SET THEORY AND REAL NUMBER SYSTEM BOOK - CENGAGE PUBLICATIONCHAPTER - SET THEORY AND REAL NUMBER SYSTEM EXERCISE - Concept Application Exercise 1.5
12 Videos
- SCALER TRIPLE PRODUCTS BOOK - CENGAGE PUBLICATIONCHAPTER - SCALER TRIPLE PRODUCTS EXERCISE - DPP 2.3
11 Videos
- SOLUTIONS AND PROPERTIES OF TRIANGLE BOOK - CENGAGE PUBLICATIONCHAPTER - SOLUTIONS AND PROPERTIES OF TRIANGLE EXERCISE - Comprehension Type
6 Videos
Similar Questions
If A,B and C are three sets such that A∩B=A∩CandA∪B=A∪C then
View SolutionIf ∣∣x2−7∣∣≤9 then find the values of x
View SolutionNumber of integral value(s) of λ for which vectors x2ˆi−ˆj+xˆk,(λ−1)ˆi−2λˆj−ˆk and ˆi−ˆj+ˆk, in the order form right-handed system ∀x ∈R, is
(a) 0 (b) 2 (c) 4 (d) 6
View SolutionLet ABC be a triangle in which the line joining the circumecentre and incentre is parallel to base BC of the triangle. Then answer the following questions :
Then range of ∠A is
View SolutionIf sin2x−2sinx−1=0 has exactly four different solutions in x∈[0,nπ] , then value/values of n is/are (n∈N) 5 (b) 3 (c) 4 (d) 6
View SolutionIf n>0 and exactly 15 integers satisfying (x+6)(x-4 ) (x-5) (2x−n)≤0 then sum of digits of the least possible value of n is
View SolutionIf x is an integer satisfying x2−6x+5≤0 and x2−2x>0, then the number of possible values of x, is
View SolutionIf f(x)=[2+5|n|sinx], where n∈I has exactly 9 points of non-derivability in (0,π), then possible values of n are (where [x] dentoes greatest integer function)
View Solutionयदि f(x)=(a−xn)n, जहाँ a>0 और n एक धनात्मक पूर्णांक है, तब f{f(x)}=x होगा।
View SolutionIf x satisfies x2−2xcosθ+1=0, then the value of xn+(1xn) is
View SolutionThe least value of n∈N for which (n−4)x2+8x+n+2>0∀x∈R, is
View SolutionIf sin2x−2sinx−1=0 has exactly four different solutions in x∈[0,nπ] , then value/values of n is/are (n∈N) 5 (b) 3 (c) 4 (d) 6
View SolutionIf n>0 and exactly 15 integers satisfying (x+6)(x-4 ) (x-5) (2x−n)≤0 then least possible value of n is
View SolutionIf f(x)=(a−xn)1/n, where a>0 and n is a positive integer, then f[f(x)]=
View SolutionIf f(x)=(a−xn)1n,a>0 , n is a postive integer thenf[f(x)]=
View SolutionIf f(x)=[2+5|n|sinx], where n∈I has exactly 9 points of non-derivability in (0,π), then possible values of n are (where [x] dentoes greatest integer function)
View Solution
