If points $A a n d B$ are (1, 0) and (0, 1), respectively, and point $C$ is on the circle $x^{2} + y^{2} = 1$ , then the locus of the orthocentre of triangle $A B C$ is $x^{2} + y^{2} = 4$ $x^{2} + y^{2} - x - y = 0$ $x^{2} + y^{2} - 2 x - 2 y + 1 = 0$ $x^{2} + y^{2} + 2 x - 2 y + 1 = 0$

Video Solution

Text Solution

Verified by Experts

The correct Answer is: $x^{2} + y^{2} - 2 x - 2 y + 1 = 0$

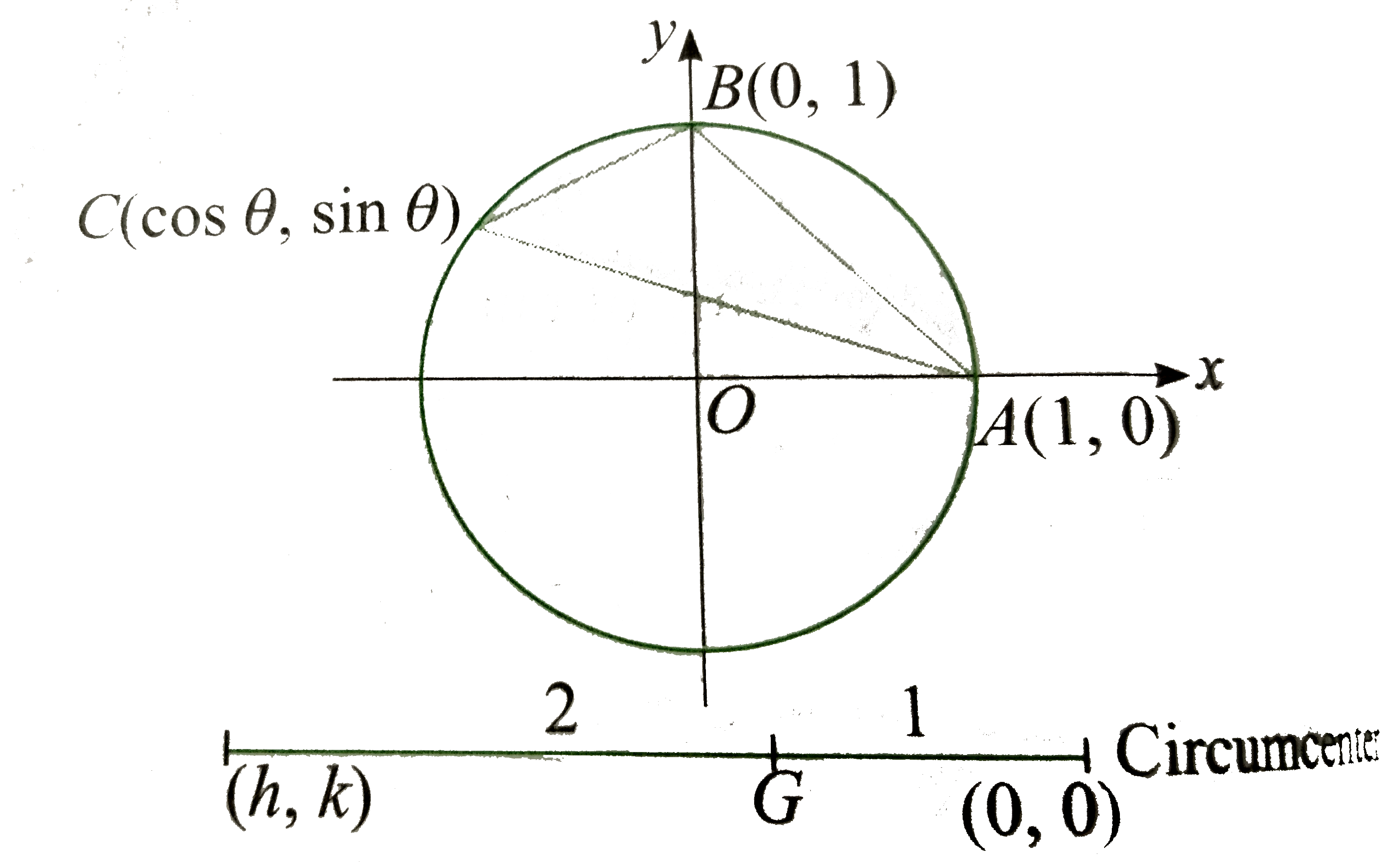
Let $C \equiv (cos θ, sin θ), H (h, k)$ is the orthocenter of $Δ A B C$ .
Since the circumcenter of the triangle is (0,0), for the orthocenter $h = 1 + cos θ$ and $k = 1 + sin θ$
Eliminating $θ$
${(x - 1)}^{2} + {(y - 1)}^{2} = 1$
or $x^{2} + y^{2} - 2 x - 2 y + 1 = 0$

|

Updated on:21/07/2023

Class 12 MATHS CIRCLE

Topper's Solved these Questions

Similar Questions

CENGAGE-CIRCLE -Exercise 4.2

Home
Ask Doubt
Profile