If points AandB are (1, 0) and (0, 1), respectively, and point C is on the circle x2+y2=1 , then the locus of the orthocentre of triangle ABC is (a) x2+y2=4 (b) x2+y2−x−y=0 (c) x2+y2−2x−2y+1=0 (d) x2+y2+2x−2y+1=0Solution in Tamil
The correct Answer is:x2+y2−2x−2y+1=0
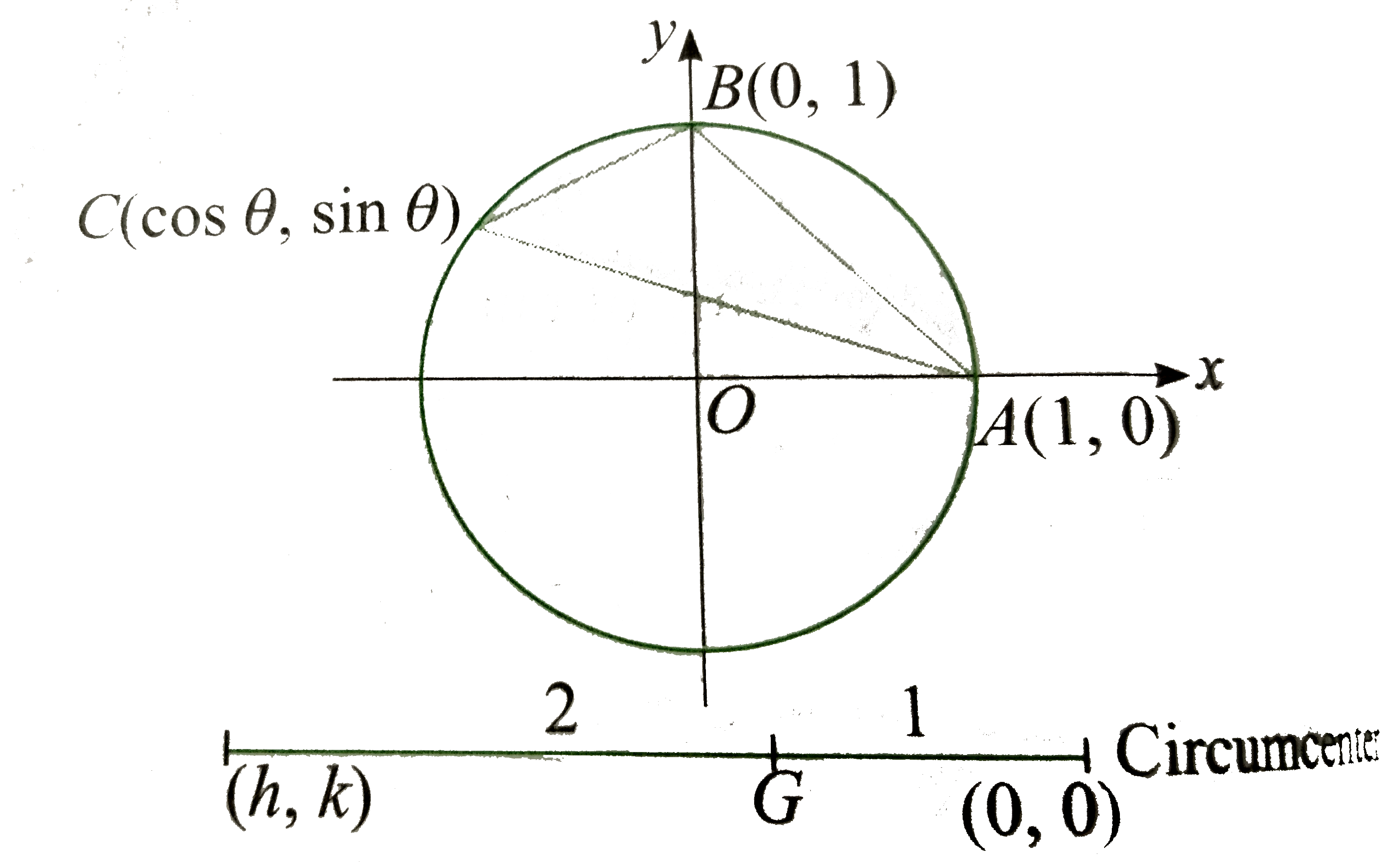
Let C≡(cosθ,sinθ),H(h,k) is the orthocenter of ΔABC.
Since the circumcenter of the triangle is (0,0), for the orthocenter h=1+cosθ and k=1+sinθ
Eliminating θ
(x−1)2+(y−1)2=1
or x2+y2−2x−2y+1=0
Topper's Solved these Questions
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise 4.3
18 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise 4.4
14 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise 4.1
14 Videos
- BINOMIAL THEOREMBOOK - CENGAGECHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension
11 Videos
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank
16 Videos
Similar Questions
Find the angle between the two tangents from the origin to the circle (x−7)2+(y+1)2=25
View SolutionFind the equation of the tangent to the circle x2+y2+4x−4y+4=0 which makes equal intercepts on the positive coordinates axes.
View SolutionIf a circle whose center is (1,−3) touches the line 3x−4y−5=0 , then find its radius.
View SolutionConsider a G.P. with first term (1+x)n, |x|<1, common ratio 1+x2 and number of terms (n+1). Let 'S' be sum of all the terms of the G.P., then
The coefficient of xn is 'S' is
View SolutionIf ax+by=10 is the chord of minimum length of the circle (x−10)2+(y−20)2=729 and the chord passes through (5,15) then the value of (4a+2b) is
View SolutionFrom point P on the circle x^(2)-y^(2)-2x+6y-8=0 .tangents PA and PB are drawn to the circle x^(2)+y^(2)-2x+6y+1=0 ,then the locus of orthocentre of Delta PAB is x^(2)+y^(2)+ax+by+c=0, where (a+b-c) is equal to -
View SolutionThe equation of the normal to the curve y=x(2−x) at the point (2, 0) is x−2y=2 (b) x−2y+2=0 (c) 2x+y=4 (d) 2x+y−4=0
View SolutionThe locus of the mid-point of a chord of the circle x2+y2−2x−2y−23=0, of length 8 units is : (A) x2+y2−x−y+1=0 (B) x2+y2−2x−2y−7=0 (C) x2+y2−2x−2y+1=0 (D) x2+y2+2x+2y+5=0
View SolutionThe circles x2+y2+2x−2y+1=0 and x2+y2−2x−2y+1=0 touch each other
View Solutionxe−ydx+ydy=0 A)2x2+(y−1)ey=c B)x22+(y−1)ey=c C)y22+(x−1)ex=c D)2y2+(x−1)ex=0
View SolutionIf y=c is a tangent to the circle x2+y2–2x+2y–2=0 at (1, 1), then the value of c is
View SolutionThe point of contact of the circle x2+y2+2x+2y+1=0andx2+y2−2x+2y+1=0
View SolutionOrthocentre of the triangle formed by the lines x+y+1=0 and 2x2+y2+x+2y−1=0 is
View SolutionIf points AandB are (1, 0) and (0, 1), respectively, and point C is on the circle x2+y2=1 , then the locus of the orthocentre of triangle ABC is x2+y2=4 x2+y2−x−y=0 x2+y2−2x−2y+1=0 x2+y2+2x−2y+1=0
View SolutionLet P be any moving point on the circle x2+y2−2x=1.AB be the chord of contact of this point w.r.t. the circle x2+y2−2x=0 . The locus of the circumcenter of triangle CAB(C being the center of the circle) is a.2x2+2y2−4x+1=0 b.x2+y2−4x+2=0 c.x2+y2−4x+1=0 d.2x2+2y2−4x+3=0
View SolutionThe equation of the normal to the curve y=x(2−x) at the point (2, 0) is
(a)x−2y=2
(b) x−2y+2=0
(c) 2x+y=4
(d) 2x+y−4=0
View Solution
