If the angle between two lines is π4and slope of one of the lines is 12, find the slope of the other line.
To find the slope of the other line given that the angle between two lines is π4 and the slope of one line (m1) is 12, we can use the formula for the tangent of the angle between two lines:
tan(θ)=|m2−m1|1+m1m2
1. Identify Given Values:
- The angle θ=π4
- The slope of the first line m1=12
2. Calculate tan(θ):
tan(π4)=1
3. Substitute Values into the Formula:
1=|m2−12|1+12m2
4. Cross Multiply to Eliminate the Fraction:
1+12m2=|m2−12|
5. Consider Two Cases for the Absolute Value:
- Case 1: m2−12≥0 (i.e., m2≥12)
- Case 2: m2−12<0 (i.e., m2<12)
Case 1: m2−12≥0
1. Remove the Absolute Value:
1+12m2=m2−12
2. Rearrange the Equation:
1+12m2+12=m2
32=m2−12m2
32=12m2
3. Solve for m2:
m2=3
Case 2: m2−12<0
1. Remove the Absolute Value:
1+12m2=−(m2−12)
2. Rearrange the Equation:
1+12m2=−m2+12
1−12=−m2−12m2
12=−32m2
3. Solve for m2:
m2=−13
Final Result:
The slope of the other line can be either m2=3 or m2=−13.
- The angle θ=π4
- The slope of the first line m1=12
tan(π4)=1
1=|m2−12|1+12m2
1+12m2=|m2−12|
- Case 1: m2−12≥0 (i.e., m2≥12)
- Case 2: m2−12<0 (i.e., m2<12)
1+12m2=m2−12
1+12m2+12=m2
32=m2−12m2
32=12m2
m2=3
1+12m2=−(m2−12)
1+12m2=−m2+12
1−12=−m2−12m2
12=−32m2
m2=−13
The slope of the other line can be either m2=3 or m2=−13.
Topper's Solved these Questions
- STRAIGHT LINESBOOK - NCERT ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE 10.3
18 Videos
- STRAIGHT LINESBOOK - NCERT ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - MISCELLANEOUS EXERCISE
24 Videos
- STATISTICSBOOK - NCERT ENGLISHCHAPTER - STATISTICSEXERCISE - EXERCISE 15.2
10 Videos
- TRIGONOMETRIC FUNCTIONSBOOK - NCERT ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONSEXERCISE - All Questions
110 Videos
Similar Questions
If the angle between two lines is 45∘ and the slope of one line is 2, then the product of possible slopes of other line is
View SolutionTwo lines passing through the point (2, 3) make an angle of 450 . If the slopeof one of the lines is 2, find the slope of the other
View SolutionCalculate slope of shown line
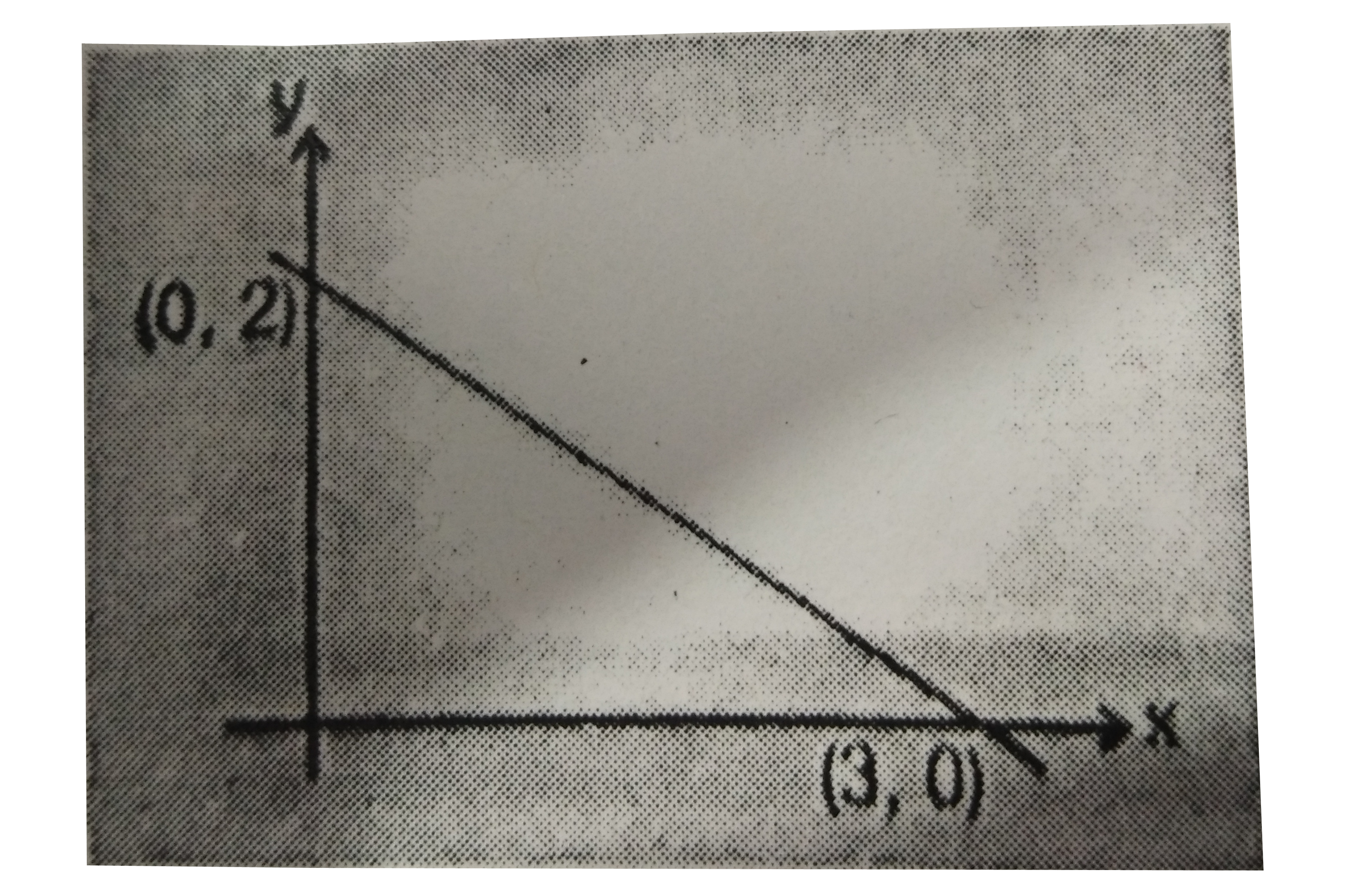
View SolutionFind the angle between the line whose slope are 2 and -1 .
View SolutionThe slope of a line is double of the slope of another line. If tangents of the angle between the is find the slopes of the other line.
View SolutionThe slope of a line is double of the slope of another line. If tangent of the angle between them is 13, find the slopes of the lines.
View SolutionTwo lines passing through the point (2,3) intersect each other at an angle 600 . If slope of one line is 2, find the equation of the other line.
View SolutionThe equation of a line is 3x+4y−7=0. Find:
the slope of the line.
View SolutionFind the angle between the lines whose slopes are -3 and −12.
View SolutionTwo lines passing through the point (2, 3) intersects each other at an angle of 60o. If slope of one line is 2, find equation of the other line.
View Solution
