If the displacement of a particle is givne by s=(12t2+4√t)m. Find the velocity and acceleration at t = 4 seconds.
The correct Answer is:a=78m/s2
To solve the problem step by step, we will first find the velocity and then the acceleration of the particle at t=4 seconds.
Step 1: Write down the displacement equation
The displacement of the particle is given by:
s=12t2+4√t meters
Step 2: Differentiate the displacement to find velocity
Velocity v is the rate of change of displacement with respect to time, which can be expressed as:
v=dsdt
Now, we differentiate s with respect to t:
v=ddt(12t2+4√t)
Using the power rule for differentiation:
- The derivative of 12t2 is t.
- The derivative of 4√t (which is 4t1/2) is 4⋅12t−1/2=2√t.
So, we have:
v=t+2√t
Step 3: Calculate the velocity at t=4 seconds
Now, we substitute t=4 into the velocity equation:
v(4)=4+2√4=4+22=4+1=5 m/s
Step 4: Differentiate the velocity to find acceleration
Acceleration a is the rate of change of velocity with respect to time, which can be expressed as:
a=dvdt
Now, we differentiate v with respect to t:
a=ddt(t+2√t)
Differentiating term by term:
- The derivative of t is 1.
- The derivative of 2√t (which is 2t−1/2) is −12⋅2t−3/2=−1t3/2.
So, we have:
a=1−1t3/2
Step 5: Calculate the acceleration at t=4 seconds
Now, we substitute t=4 into the acceleration equation:
a(4)=1−143/2=1−18=1−0.125=0.875 m/s2
Final Results
- The velocity at t=4 seconds is 5 m/s.
- The acceleration at t=4 seconds is 0.875 m/s².
The displacement of the particle is given by:
s=12t2+4√t meters
Velocity v is the rate of change of displacement with respect to time, which can be expressed as:
v=dsdt
Now, we differentiate s with respect to t:
v=ddt(12t2+4√t)
- The derivative of 12t2 is t.
- The derivative of 4√t (which is 4t1/2) is 4⋅12t−1/2=2√t.
v=t+2√t
Now, we substitute t=4 into the velocity equation:
v(4)=4+2√4=4+22=4+1=5 m/s
Acceleration a is the rate of change of velocity with respect to time, which can be expressed as:
a=dvdt
Now, we differentiate v with respect to t:
a=ddt(t+2√t)
- The derivative of t is 1.
- The derivative of 2√t (which is 2t−1/2) is −12⋅2t−3/2=−1t3/2.
a=1−1t3/2
Now, we substitute t=4 into the acceleration equation:
a(4)=1−143/2=1−18=1−0.125=0.875 m/s2
- The velocity at t=4 seconds is 5 m/s.
- The acceleration at t=4 seconds is 0.875 m/s².
Topper's Solved these Questions
- DY / DX AS A RATE MEASURER AND TANGENTS, NORMALS BOOK - ARIHANT MATHS ENGLISHCHAPTER - DY / DX AS A RATE MEASURER AND TANGENTS, NORMALS EXERCISE - EXAMPLE
6 Videos
- DY / DX AS A RATE MEASURER AND TANGENTS, NORMALS BOOK - ARIHANT MATHS ENGLISHCHAPTER - DY / DX AS A RATE MEASURER AND TANGENTS, NORMALS EXERCISE - SINGLE OPTION CORRECT TYPE QUESTIONS
9 Videos
- DIFFERENTIATION BOOK - ARIHANT MATHS ENGLISHCHAPTER - DIFFERENTIATION EXERCISE - Exercise For Session 10
4 Videos
- ELLIPSEBOOK - ARIHANT MATHS ENGLISHCHAPTER - ELLIPSEEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
27 Videos
Similar Questions
At time t , the displacement of a particle moving in a straight line by x=−4t2+2t . Find the velocity and acceleration a when t = (1/2) s .
View SolutionThe displacement of a particle along the x-axis is given by x=3+8t+7t2. Obtain its velocity and acceleration at t=2s.
View SolutionThe velocity of a particle is given by v=(2t2−3t+10)ms−1. Find the instantaneous acceleration at t = 5 s.
View SolutionA particle is moving in a straight line. Its displacement at time t is given by s(Inm)=4t2+2t, then its velocity and acceleration at time t=12 second are
View SolutionThe velocity of a particle is given by v=(2t2−4t+3)m/s where t is time in seconds. Find its acceleration at t=2 second.
View SolutionThe velocity of a particle is given by v=(4t2−4t+3)m/s where t is time in seconds. Find its acceleration at t=1 second.
View SolutionA particle moves along a staight line such that its displacement at any time t is given by s=t3−6t2+3t+4m. Find the velocity when the acceleration is 0.
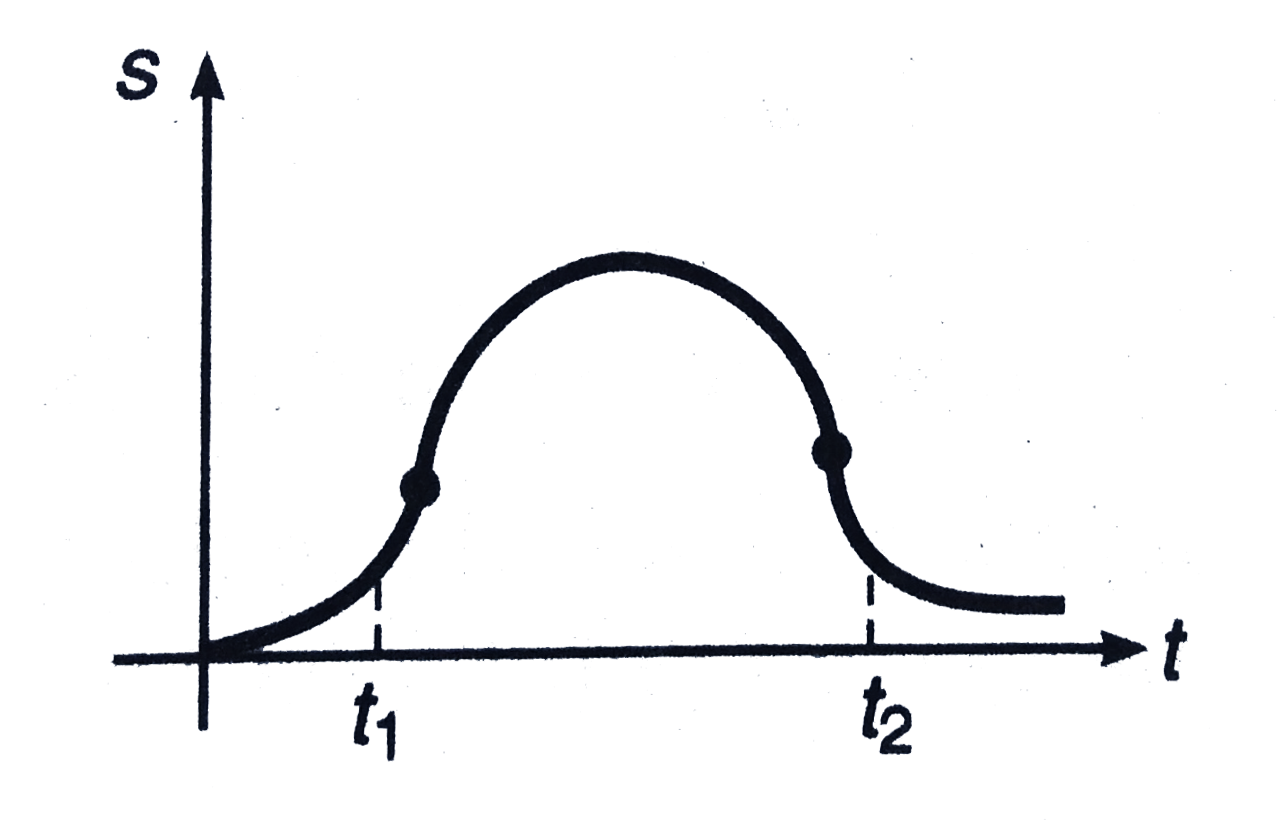
View SolutionFigure shows the displacement-time graph of a particle moving in a straight line. Find the signs of velocity and acceleration of particle at time t=t1andt=t2.
View SolutionFor a particle moving in a straight line, the displacement of the particle at time t is given by
S=t3−6t2+3t+7
What is the velocity of the particle when its acceleration is zero?
View SolutionFor a particle moving in a straight line, the displacement of the particle at time t is given by
S=t3−6t2+3t+7
What is the velocity of the particle when its acceleration is zero?
View Solution
