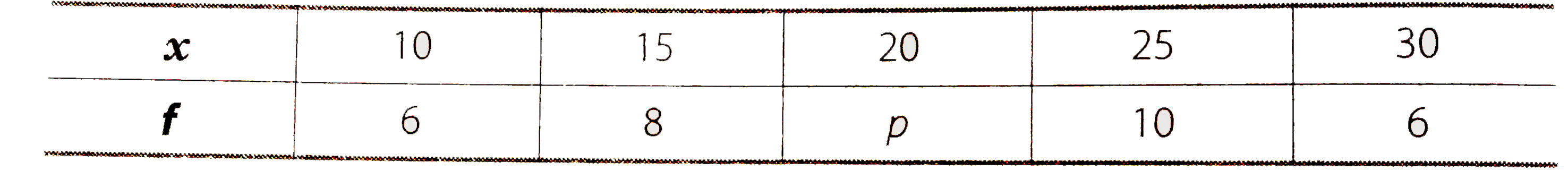
If the mean of the following data is 15, find
To solve the problem where the mean of the given data is 15, we need to find the value of p in the frequency distribution. The data provided is as follows:
- 5 occurs 6 times
- 10 occurs p times
- 15 occurs 6 times
- 20 occurs 10 times
- 35 occurs 5 times
1. Understand the Mean Formula:
The mean (¯x) is calculated using the formula:
¯x=∑(f⋅x)∑f
where f is the frequency and x is the observation.
2. Set Up the Equation:
Given that the mean is 15, we can set up the equation:
(6⋅5)+(p⋅10)+(6⋅15)+(10⋅20)+(5⋅35)(6+p+6+10+5)=15
3. Calculate the Numerator:
Calculate each term in the numerator:
- 6⋅5=30
- p⋅10=10p
- 6⋅15=90
- 10⋅20=200
- 5⋅35=175
So, the numerator becomes:
30+10p+90+200+175=495+10p
4. Calculate the Denominator:
Calculate the total frequency:
6+p+6+10+5=27+p
5. Set Up the Equation with the Mean:
Now we substitute the numerator and denominator into the mean equation:
495+10p27+p=15
6. Cross-Multiply:
Cross-multiply to eliminate the fraction:
495+10p=15(27+p)
7. Expand the Right Side:
Expand the right side:
495+10p=405+15p
8. Rearrange the Equation:
Rearranging gives:
495−405=15p−10p
Simplifying this results in:
90=5p
9. Solve for p:
Divide both sides by 5:
p=905=18
Conclusion:
The value of p is 18.
- 10 occurs p times
- 15 occurs 6 times
- 20 occurs 10 times
- 35 occurs 5 times
The mean (¯x) is calculated using the formula:
¯x=∑(f⋅x)∑f
where f is the frequency and x is the observation.
Given that the mean is 15, we can set up the equation:
(6⋅5)+(p⋅10)+(6⋅15)+(10⋅20)+(5⋅35)(6+p+6+10+5)=15
Calculate each term in the numerator:
- 6⋅5=30
- p⋅10=10p
- 6⋅15=90
- 10⋅20=200
- 5⋅35=175
30+10p+90+200+175=495+10p
Calculate the total frequency:
6+p+6+10+5=27+p
Now we substitute the numerator and denominator into the mean equation:
495+10p27+p=15
Cross-multiply to eliminate the fraction:
495+10p=15(27+p)
Expand the right side:
495+10p=405+15p
Rearranging gives:
495−405=15p−10p
Simplifying this results in:
90=5p
Divide both sides by 5:
p=905=18
The value of p is 18.
Topper's Solved these Questions
Similar Questions
If the mean of the following data is 5.6, find p :

View SolutionFind the mean of the following data :

View SolutionFind the mean of the following data :
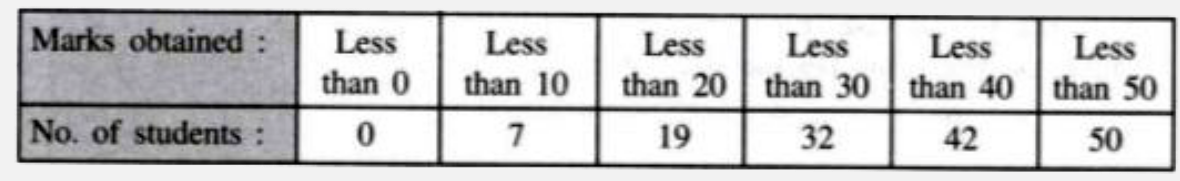
View SolutionFind the mean of the following data:
View SolutionIf the mean of the following data is 26, then find the value of p:
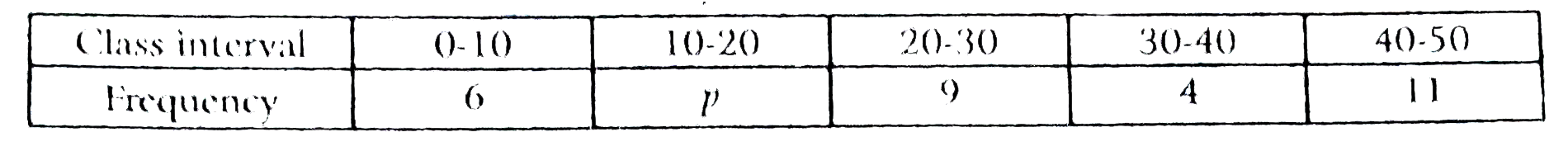
View SolutionIf the mean of the following data is 20.6. Find the value of p . x : 10 15 p 25 35 f : 3 10 25 7 5
View SolutionThe arithmetic mean of the following data is 25, find the value of k . xi : 5 15 25 35 45 fi : 3 k 3 6 2
View SolutionFind the mean for the following data
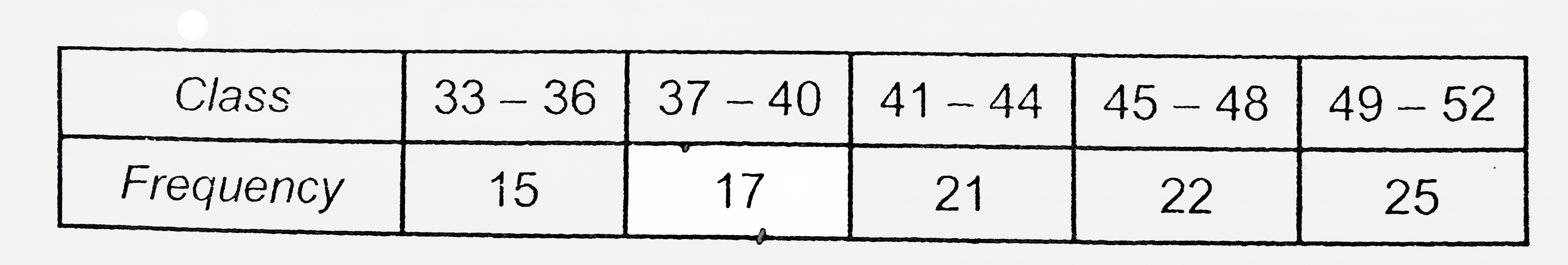
View SolutionIf the mean of the following data is 20.2, then find the value of p.
View SolutionFind the mean for the following data:
View Solution
