If the probability distribution of a random variable X is given by X=xi: 1 2 3 4 P(X=xi): 2k 4k 3k k Write the value of k .
The correct Answer is:The value of k=110
To have distribution of probabilities becomes a probability distribution, then the Sum of probability distribution is 1, then,
ΣP(xi)=1
P(X=1)+P(X=2)+P(X=3)+P(X=4)=1
⇒2k+4k+3k+k=1
⇒10k=1⇒k=110
Thus the value of k is:110
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
The probability distribution of a discrete random variable X is given below
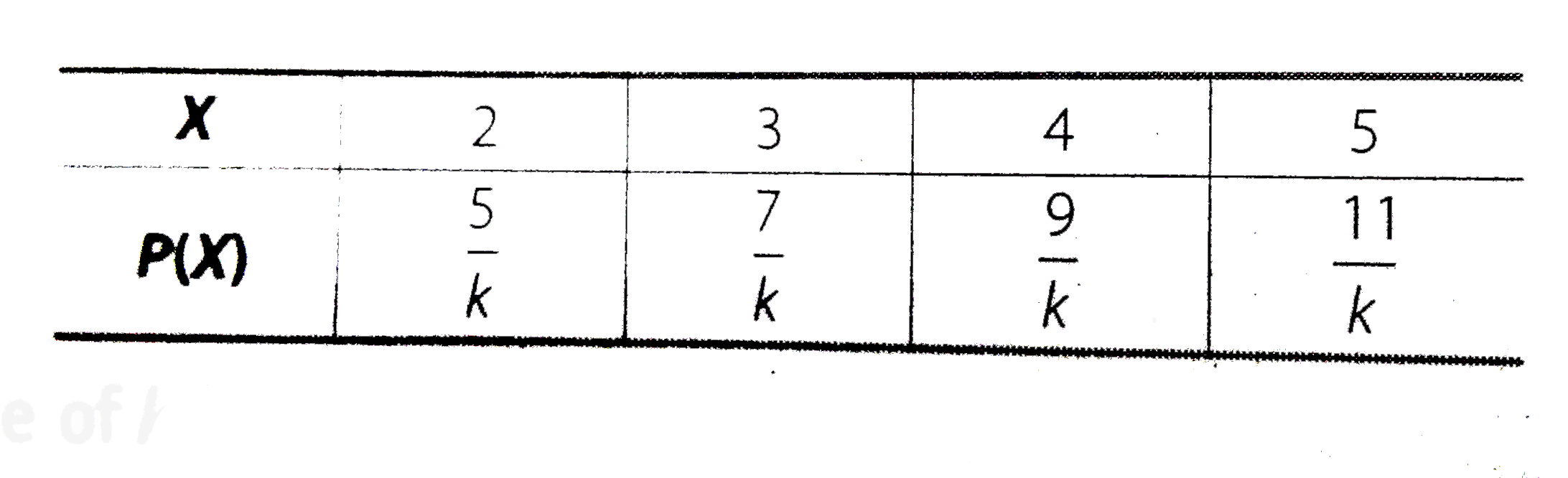
The value of k isA8B16C32D48 - Question 2 - Select One
The probability distribution of a random variable X is given as follows:
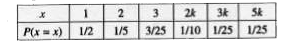
If E(X)=2.3 , then value of k isA1/2B5/4C3D2
The probability of a random variable X is given below
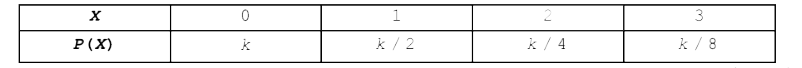
Determine the value of kView SolutionThe probability distribution of a discrete random variable X is given below: X: 2 3 4 5 P(X):5k 7k 9k 11k The value of E(X) is a. 8 b. 16 c. 32 d. 48
View SolutionThe probability distribution of a discrate random variable X is :
X=x12345P(X=x)k2k3k4k5k
Find P(X≤4).View SolutionThe probability distribution of a random variable X is given below: X: 0 1 2 3 P(X):k k2 k4 k8 (i) Determine the value of k (ii) Determine P(X≤2)and P(X>2) (iii) Find P(X≤2)+P(X>2)
View SolutionThe probability distribution of random variable X is given by :
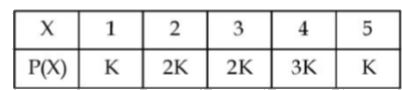
Let p=P(1<X<4|X<3). If 5p=λK, then λ is equal to _________.View SolutionThe probability distribution of discrete random variable X is given below
x2345P(x)5K7k9k11k
find the value of K.View Solution

