If the probability function of a random variable X is defined by P(X=k)=a(k+12k) for k=0,1,2,3,4,5 then the probability that X takes a prime value is
1320
2360
1120
1960
The correct Answer is:B
To solve the problem, we need to find the probability that the random variable X takes a prime value, given the probability function P(X=k)=a(k+1)2k for k=0,1,2,3,4,5.
1. Identify Prime Values:
The prime numbers in the range of k=0,1,2,3,4,5 are 2,3, and 5.
2. Write the Probability for Prime Values:
We need to find P(X=2)+P(X=3)+P(X=5).
3. Calculate Each Probability:
Using the given probability function:
- P(X=2)=a(2+1)22=a34
- P(X=3)=a(3+1)23=a48=a12
- P(X=5)=a(5+1)25=a632=a316
4. Sum the Probabilities:
Now, we sum these probabilities:
P(X=2)+P(X=3)+P(X=5)=a34+a12+a316
5. Find a Common Denominator:
The common denominator for 4,2, and 16 is 16:
- P(X=2)=a3×416=a1216
- P(X=3)=a1×816=a816
- P(X=5)=a316
Thus,
P(X=2)+P(X=3)+P(X=5)=a(12+8+316)=a2316
6. Determine the Value of a:
To find a, we use the fact that the total probability must equal 1:
P(X=0)+P(X=1)+P(X=2)+P(X=3)+P(X=4)+P(X=5)=1
Calculate each term:
- P(X=0)=a11=a
- P(X=1)=a22=a
- P(X=2)=a34
- P(X=3)=a12
- P(X=4)=a516
- P(X=5)=a316
Therefore,
a+a+a34+a12+a516+a316=1
Combine the terms:
3a+a(1216+816+516+316)=1
3a+a2816=1
a(3+2816)=1
a(48+2816)=1
a7616=1⟹a=1676=419
7. Substitute a back into the Probability of Prime Values:
Now substitute a into the sum of probabilities for prime values:
P(X is prime)=4192316=4×2319×16=92304=2376
Final Answer:
The probability that X takes a prime value is 2376.
The prime numbers in the range of k=0,1,2,3,4,5 are 2,3, and 5.
We need to find P(X=2)+P(X=3)+P(X=5).
Using the given probability function:
- P(X=2)=a(2+1)22=a34
- P(X=3)=a(3+1)23=a48=a12
- P(X=5)=a(5+1)25=a632=a316
Now, we sum these probabilities:
P(X=2)+P(X=3)+P(X=5)=a34+a12+a316
The common denominator for 4,2, and 16 is 16:
- P(X=2)=a3×416=a1216
- P(X=3)=a1×816=a816
- P(X=5)=a316
P(X=2)+P(X=3)+P(X=5)=a(12+8+316)=a2316
To find a, we use the fact that the total probability must equal 1:
P(X=0)+P(X=1)+P(X=2)+P(X=3)+P(X=4)+P(X=5)=1
Calculate each term:
- P(X=0)=a11=a
- P(X=1)=a22=a
- P(X=2)=a34
- P(X=3)=a12
- P(X=4)=a516
- P(X=5)=a316
a+a+a34+a12+a516+a316=1
3a+a(1216+816+516+316)=1
3a+a2816=1
a(3+2816)=1
a(48+2816)=1
a7616=1⟹a=1676=419
Now substitute a into the sum of probabilities for prime values:
P(X is prime)=4192316=4×2319×16=92304=2376
The probability that X takes a prime value is 2376.
Topper's Solved these Questions
- PROBABILITY DISTRIBUTIONBOOK - TARGET PUBLICATIONCHAPTER - PROBABILITY DISTRIBUTIONEXERCISE - Evaluation test
5 Videos
- PROBABILITY DISTRIBUTIONBOOK - TARGET PUBLICATIONCHAPTER - PROBABILITY DISTRIBUTIONEXERCISE - Critical Thinking
33 Videos
- PLANEBOOK - TARGET PUBLICATIONCHAPTER - PLANEEXERCISE - EVALUATION TEST
14 Videos
- THREE DIMENSIONAL GEOMETRY BOOK - TARGET PUBLICATIONCHAPTER - THREE DIMENSIONAL GEOMETRY EXERCISE - Evaluation Test
9 Videos
Similar Questions
Knowledge Check
If the probability mass function of a discrete random variable X is
P(x)=Cx3,x=1,2,3
=0, otherwise
Then E(X)=A343297B294251C297294D251294If the probability density function of a random variable X is f(x)=π2 in 0≤x≤2, then P(X>1.5X>1) is equal to
A716B34C712D2164If the range of a random variable X is {0,1,2,3,4….}with P(X)=k)=(k+1)α3k fork≥0, then α is equal to
A23B49C827D1681
If the probability distribution of a random variable X is given by X=xi: 1 2 3 4 P(X=xi): 2k 4k 3k k Write the value of k .
View SolutionIf the range ot a random vaniabie X is 0,1,2,3, at P(X=K)=(K+13k) a for k≥0, then a equals
View SolutionThe range of a random variable X is 1,2,3,..., and P(X=k)=ckk! where k=1,2,3... .Find the value of P(0<X<3)
View SolutionThe probability distribution of a discrete random variable X is given below
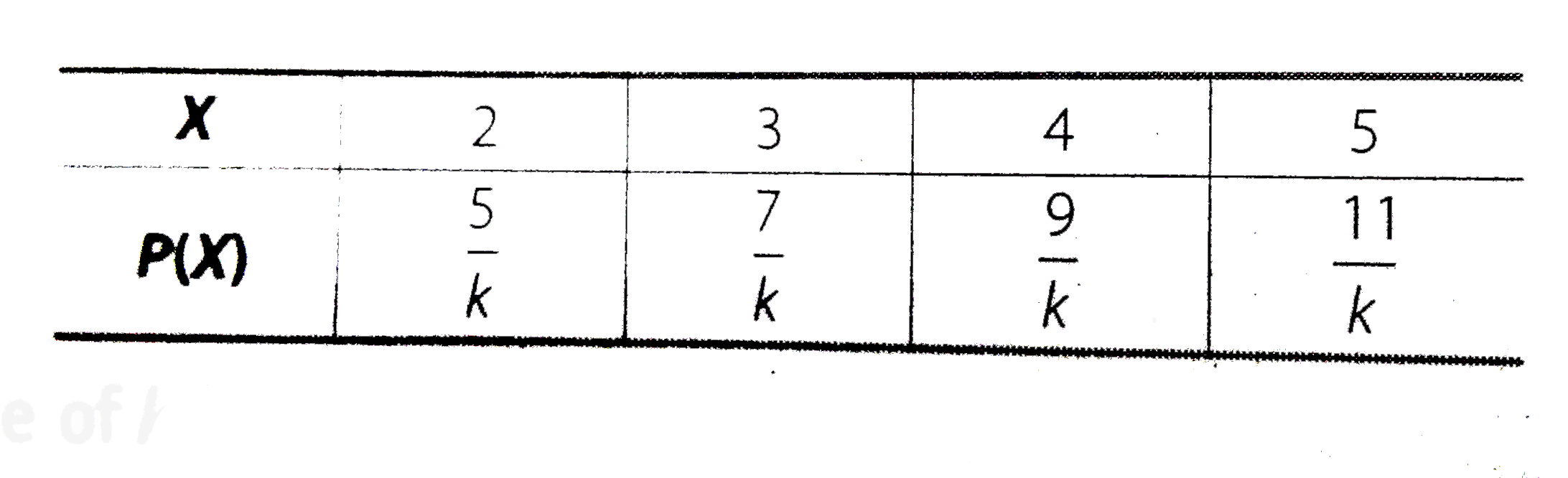
The value of k is
View SolutionThe probability distribution of a random variable X is given as follows:
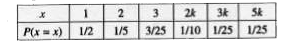
If E(X)=2.3 , then value of k is
View Solution