If the quadratic equation $x^{2} - 36 x + λ = 0$ has roots $α$ and $β$ such that $α$ , $β \in N$ and (lambda)/(5) cancel in Z and $λ$ assumes minimum possible value then $\frac{\sqrt{α + 2} \sqrt{β + 2}}{| α - β |}$ is equal to

A

$\frac{3}{8}$

B

$\frac{3}{16}$

C

$\frac{\sqrt{111}}{34}$

D

$\frac{\sqrt{111}}{17}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

$(a)$ $x^{2} - 36 x + λ = 0$
$∴ Λ = 36 x - x^{2}$
$α = 1$ , $λ = 5$ , $β = 35$ (rejected)

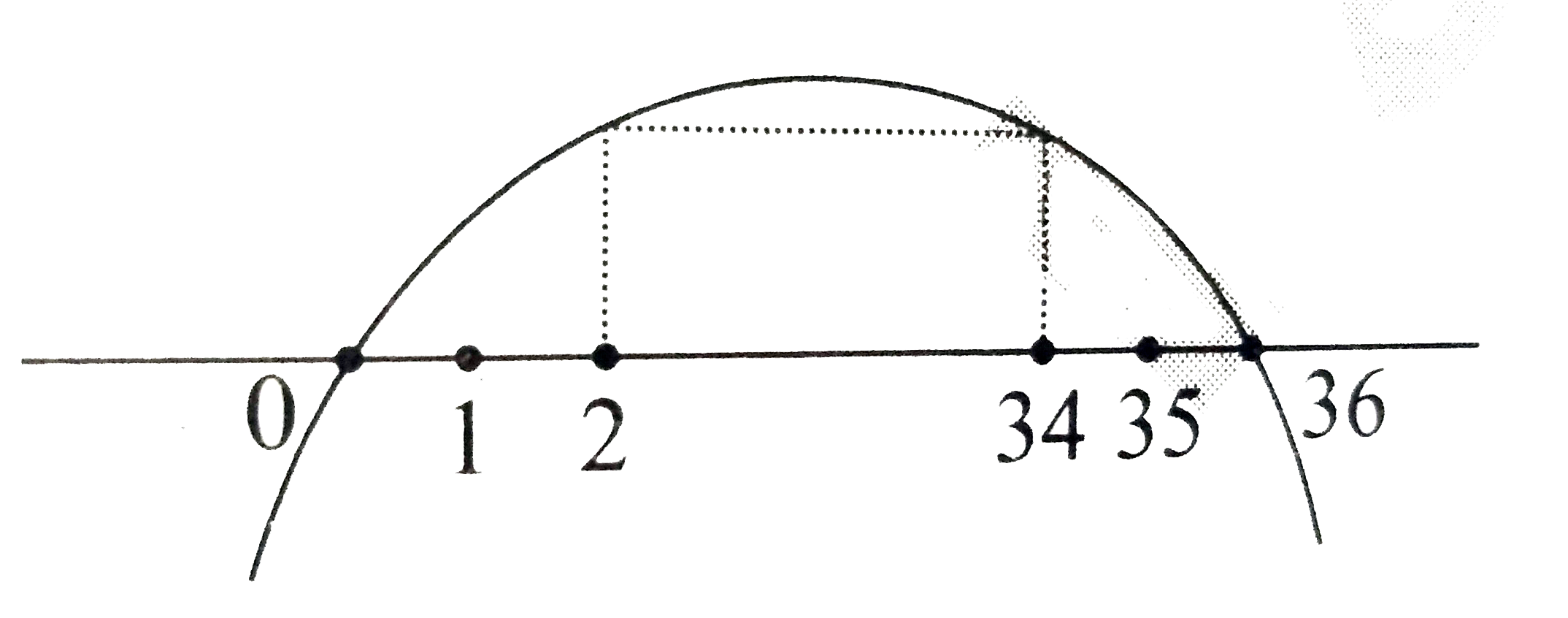
$∴ α = 2$ , $λ = 68$ , $β = 34$ or $α = 34$ , $β = 2$
$∴ \frac{\sqrt{36} \sqrt{4}}{32} = \frac{3}{8}$

|

Updated on:21/07/2023

Class 12 MATHS QUADRATIC EQUATIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
If the equation $2 x^{2} - 7 x + 12 = 0$ has two roots $α and β$ then the value of $\frac{α}{β} + \frac{β}{α}$ is
A $\frac{7}{2}$
B $\frac{1}{24}$
C $\frac{7}{24}$
D $\frac{97}{24}$

CENGAGE-THEORY OF EQUATIONS-JEE Advanced Previous Year

Home
Ask Doubt
Profile