If three square are chosen at random on a chess Board, show that chance that they should be in a diagonal line 7/744.

A

$\frac{7}{744}$

B

$\frac{5}{744}$

C

$\frac{7}{544}$

D

$\frac{11}{744}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

We can choose three squares in a diagonal line parallel to BD in the $Δ A B D$ . Clearly, three squares in $Δ A B D$ and in a diagonal line parallel to BD can be chosen in

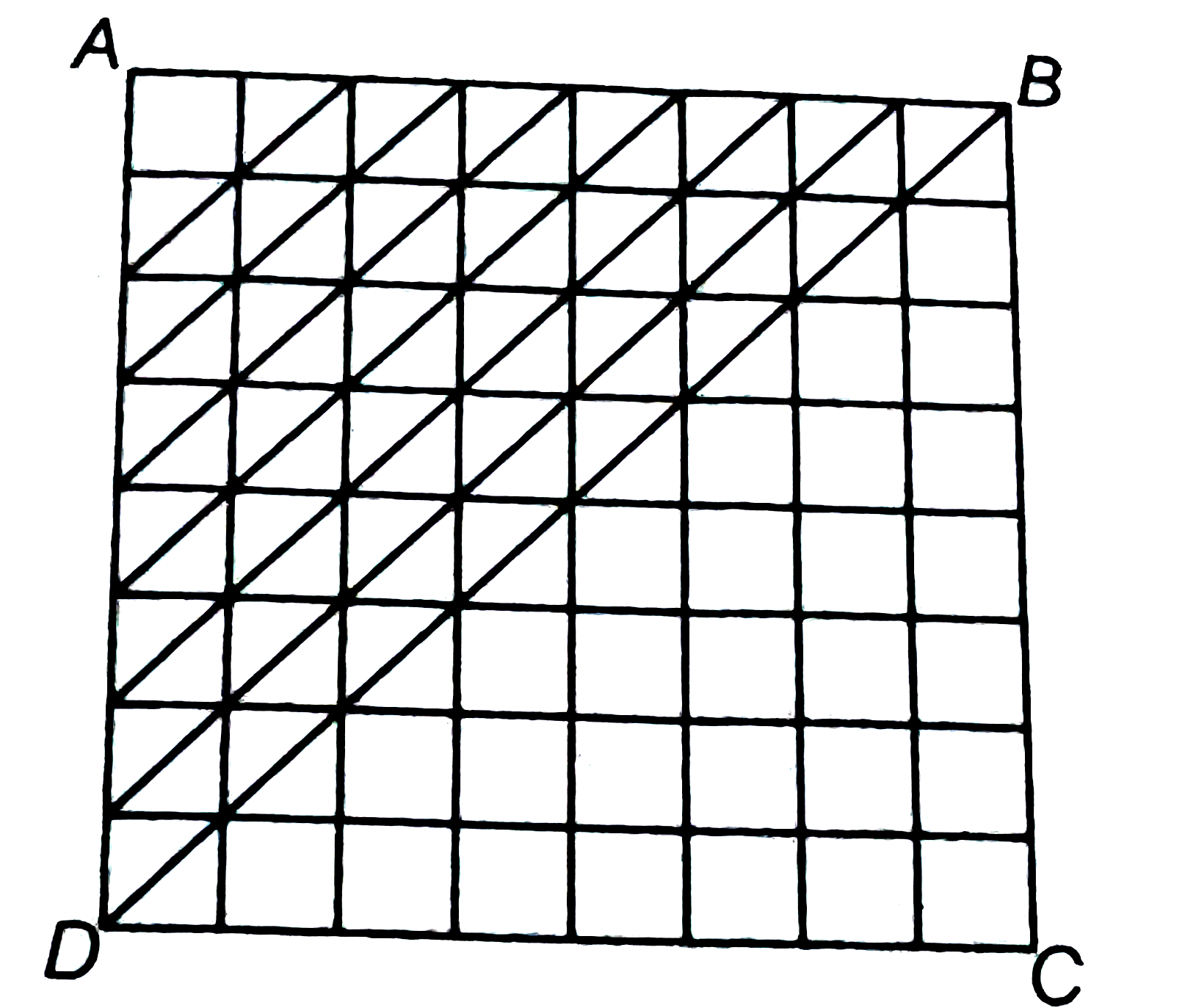
$.^{3} C_{3} + .^{4} C_{3} + .^{5} C_{3} + .^{6} C_{3} + .^{7} C_{3} + .^{8} C_{3}$ ways
Similarly, in $Δ B C D$ , the squares can be chosen parallel to BD in an equal number of ways. Hence, the total number of ways in which three squares can be chosen in a diagonal line parallel to BD is
$2 (.^{3} C_{3} + .^{4} C_{3} + .^{5} C_{3} + .^{6} C_{3} + .^{7} C_{3}) + .^{8} C_{3}$ [ $∵$ BD is common to both the triangles]
Similarly, squares can be chosen in a diagonal line parallel to AC and hence the total number of favourable ways
$= 4 (.^{3} C_{3} + .^{4} C_{3} + .^{5} C_{3} + .^{6} C_{3} + .^{7} C_{3}) + 2 .^{8} C_{3} = 392$
Hence, the required probability $= \frac{392}{.^{64} C_{3}}$
$= \frac{392 \times 6}{64.63 .62} = \frac{7}{744}$

|

Updated on:21/07/2023

Class 12 MATHS PROBABILITY

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Two squares are chosen at random on a chessboard, the probability that they have a side in common is:
A $\frac{3}{32}$
B $\frac{1}{32}$
C $1 / 18$
Dnone of these
Question 2 - Select One
Two squares are chosen at random on a chessboard, the probability that they have a side in common is:
A $\frac{3}{32}$
B $\frac{1}{32}$
C $1 / 18$
Dnone of these
Question 3 - Select One
The number of squares that we can find on a chess board is
A64
B160
C224
D204

MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERS-PROBABILITY-PRACTICE EXERCISE (Exercies 2 (MISCELLANEOUS PROBLEMS))

Home
Ask Doubt
Profile