If two sides of a triangle are represented by x2_7xy+6y2=0 and the centroid is (1,0) then the equation of third side is
2x+7y+3=0
2x−7y+3=0
2x+7y−3=0
2x−7y−3=0
The correct Answer is:D
Given , two lines are x−6y=0andx−y=0.
We know that , 0+x1+x23=1
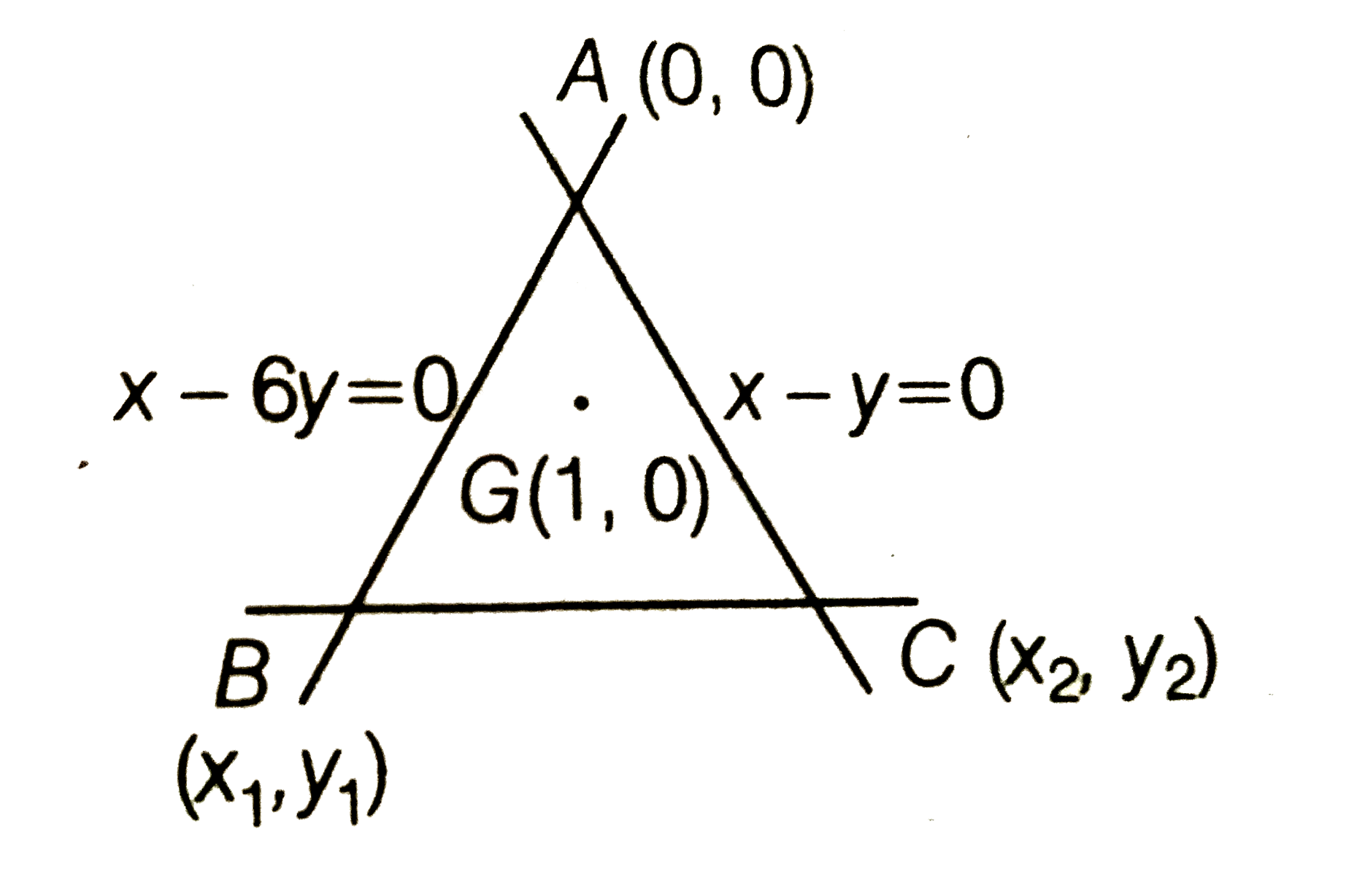
and 0+y1+y23=0
⇒x1+x2=3andy1,y2=0 ......(i)
Also , x1−6y1=0 ......(ii)
and x2−y2=0 ......(iii)
On solving , coordinates of B and C are (185,35) and (−35,−35) , respectively.
Hence , the equation of third side i.e., BC is 2x−7y−3=0.
Topper's Solved these Questions
- PAIR OR STRAIGHT LINES BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - PAIR OR STRAIGHT LINES EXERCISE - MHT CET Corner
13 Videos
- PAIR OR STRAIGHT LINES BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - PAIR OR STRAIGHT LINES EXERCISE - PRACTICE EXCERCISE (Excercise 1) (Topical Problems) (General Equation of Second Degree)
20 Videos
- MOCK TEST 5BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - MOCK TEST 5EXERCISE - MCQS
50 Videos
- PLANEBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - PLANEEXERCISE - MHT CET Corner
9 Videos
Similar Questions
If two sides of a triangle lie along 3x2−5xy+2y2=0 and its orthocenter is (2,1) then the equation to the third side is
View SolutionLet equation of two sides of a triangle are 4x+5y=20 and 3x−2y+6=0 If orthocentre of triangle is (1,1) then the equation of third side of triangle is (a) y+10=−1361(x+352) (2) y+10=−1361(x−352) (3) y+10=1361(x−352) (4) y−10=1361(x−352)
View Solution
Knowledge Check
Two lines represented by equation x2+xy+y2=0 are
AcoincidentBparallelCmutually perpendicularDimaginaryIf two sides of a triangle are roots of the equation x2−7x+8=0 and the angle between these sides is 60∘ then the product of inradius and circumradius of the triangle is
A87B53C5√23D8
The equations of two sides of a triangle are 3x−2y+6=0 and 4x+5y−20 and the orthocentre is (1,1). Find the equation of the third side.
View Solutionnd (, The eqn of two sides of a triangle are 3x-2y + 6-0 and 4x + 5y = 20 and the orthocentre is (1,1). Find the equation of the thired side.
View Solution. If 2x2−5xy+2y2=0 represents two sides of triangle whose centroid is (1, 1) then the equation of the third side is (A) x+y−3=0 (B) x−y−3=0 (C) x+y+3=0 (D) x−y+3=0
View SolutionIf two equal sides of a triangle are x2+4xy+y2=0 then locus of mid point of third side is
View SolutionIf two sides of a triangle are the roots of theequation 4x2−(2√6)x+1=0 and the included angle is 60∘ , then the third side is
View SolutionTwo equal sides of an isosceles triangle are 7x−y+3=0,x−y−3=0 and its third side passes through the point (1,0) the equation of the third side is (A) 3x+y+7=0 (B) x−3y+29=0 (C) 3x+y+3=0 (D) 3x+y−3=0
View Solution
