If X= {a,b,c,d} , Y = {f , b,d,g} , find:
(i) X-Y
(ii) Y-X.
The correct Answer is:(i) {a,c}
(ii) {f,g}
To solve the problem, we need to find the difference between the two sets X and Y.
Given:
- X={a,b,c,d}
- Y={f,b,d,g}
1. Definition: X−Y means we take all the elements from set X and remove any elements that are also in set Y.
2. Check each element of X:
- Element a:
- Is a in Y? No.
- So, include a in X−Y.
- Element b:
- Is b in Y? Yes.
- So, do not include b in X−Y.
- Element c:
- Is c in Y? No.
- So, include c in X−Y.
- Element d:
- Is d in Y? Yes.
- So, do not include d in X−Y.
3. Result:
- After checking all elements, we find that X−Y={a,c}.
(ii) Finding Y−X
1. Definition: Y−X means we take all the elements from set Y and remove any elements that are also in set X.
2. Check each element of Y:
- Element f:
- Is f in X? No.
- So, include f in Y−X.
- Element b:
- Is b in X? Yes.
- So, do not include b in Y−X.
- Element d:
- Is d in X? Yes.
- So, do not include d in Y−X.
- Element g:
- Is g in X? No.
- So, include g in Y−X.
3. Result:
- After checking all elements, we find that Y−X={f,g}.
Final Answers:
- X−Y={a,c}
- Y−X={f,g}
- X={a,b,c,d}
- Y={f,b,d,g}
2. Check each element of X:
- Element a:
- Is a in Y? No.
- So, include a in X−Y.
- Element b:
- Is b in Y? Yes.
- So, do not include b in X−Y.
- Element c:
- Is c in Y? No.
- So, include c in X−Y.
- Element d:
- Is d in Y? Yes.
- So, do not include d in X−Y.
- After checking all elements, we find that X−Y={a,c}.
- Element f:
- Is f in X? No.
- So, include f in Y−X.
- Element b:
- Is b in X? Yes.
- So, do not include b in Y−X.
- Element d:
- Is d in X? Yes.
- So, do not include d in Y−X.
- Element g:
- Is g in X? No.
- So, include g in Y−X.
- After checking all elements, we find that Y−X={f,g}.
- X−Y={a,c}
- Y−X={f,g}
Topper's Solved these Questions
- SETS BOOK - MODERN PUBLICATIONCHAPTER - SETS EXERCISE - CHECK YOUR UNDERSTANDING
10 Videos
- SETS BOOK - MODERN PUBLICATIONCHAPTER - SETS EXERCISE - COMPETITION FILE
9 Videos
- SETS BOOK - MODERN PUBLICATIONCHAPTER - SETS EXERCISE - OBJECTIVE TYPE QUESTIONS (C ) TRUE / FALSE QUESTINS
5 Videos
- SEQUENCES AND SERIESBOOK - MODERN PUBLICATIONCHAPTER - SEQUENCES AND SERIESEXERCISE - CHAPTER TEST
12 Videos
- STATISTICSBOOK - MODERN PUBLICATIONCHAPTER - STATISTICSEXERCISE - Chapter Test
7 Videos
Similar Questions
Knowledge Check
If ax+y=a6andx>y by 2 find x.
A. 1
B. 2
C. 3
D. 4ADBCCADBIf ax=by=cz=du and a, b, c, d are in G.P. then x, y, z, u are in
AA.P.BG.P.CH.P.Dnone of thesev49_newFlow
AaB0C2aD2/a
If a,b,c,d,e, and fare in G.P. then the value of ∣∣ ∣ ∣∣(a2)(d2)x(b2)(e2)y(c2)(f2)z∣∣ ∣ ∣∣ depends on (A) x and y (B) x and z (C) y and z (D) independent of x ,y ,and z
View SolutionEvaluate each of the following algebraic expressions for x=1, y=−1,z=2, a=−2, b=1, c=−2: (i) ax+by+cz (ii) ax2+by2−cz2 (iii) axy+byz+cxy
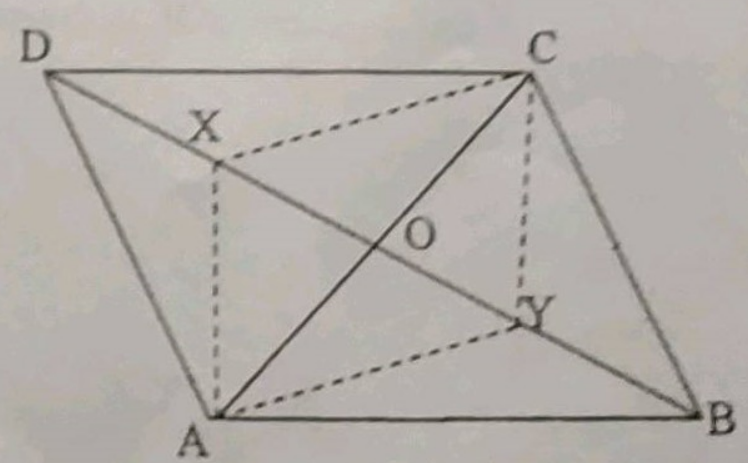
View SolutionIn Figure, ABCD is a parallelogram and X and Y are points on the diagonal BD such that DX=BY . Prove that AXCY is a parallelogram (ii) AX=CY, AY=CX AYB ≅CXD (iv) AXD≅ CYB
View SolutionIf a,b,c,d are in A.P. and x,y,z are in G.P., then show that xb−c.yc−a.za−b=1.
View SolutionIf d is the H.C.F. of 30, 72, find the value of x & y satisfying d=30x+72y.
View Solution
