If x1,x2andy1,y2 are the roots of the equations
3x2−18x+9=0andy2−4y+2=0 the value of the determinant ∣∣
∣
∣∣x1x2y1y21x1+x2y1+y22sin(πx1x2)cos(π/2y1y2)1∣∣
∣
∣∣ is
(a)0
(b)1
(c)2
(d)None of these
The correct Answer is:A
To solve the given problem, we will follow these steps:
Step 1: Find the roots of the equations
1. For the equation 3x2−18x+9=0:
- The sum of the roots x1+x2=−ba=−−183=6.
- The product of the roots x1x2=ca=93=3.
2. For the equation y2−4y+2=0:
- The sum of the roots y1+y2=−ba=−−41=4.
- The product of the roots y1y2=ca=21=2.
Step 2: Substitute the values into the determinant
We need to evaluate the determinant:
D=∣∣
∣
∣∣x1x2y1y21x1+x2y1+y22sin(πx1x2)cos(π2y1y2)1∣∣
∣
∣∣
Substituting the values we found:
- x1x2=3
- y1y2=2
- x1+x2=6
- y1+y2=4
- sin(πx1x2)=sin(3π)=0
- cos(π2y1y2)=cos(π)=−1
So the determinant becomes:
D=∣∣
∣∣3216420−11∣∣
∣∣
Step 3: Calculate the determinant
Using the formula for the determinant of a 3x3 matrix:
D=a(ei−fh)−b(di−fg)+c(dh−eg)
where:
- a=3, b=2, c=1
- d=6, e=4, f=2
- g=0, h=−1, i=1
Calculating each part:
1. ei−fh=4⋅1−2⋅(−1)=4+2=6
2. di−fg=6⋅1−2⋅0=6−0=6
3. dh−eg=6⋅(−1)−4⋅0=−6−0=−6
Now substituting back:
D=3⋅6−2⋅6+1⋅(−6)
D=18−12−6=0
Final Answer
The value of the determinant is 0.
1. For the equation 3x2−18x+9=0:
- The sum of the roots x1+x2=−ba=−−183=6.
- The product of the roots x1x2=ca=93=3.
- The sum of the roots y1+y2=−ba=−−41=4.
- The product of the roots y1y2=ca=21=2.
We need to evaluate the determinant:
D=∣∣
∣
∣∣x1x2y1y21x1+x2y1+y22sin(πx1x2)cos(π2y1y2)1∣∣
∣
∣∣
- x1x2=3
- y1y2=2
- x1+x2=6
- y1+y2=4
- sin(πx1x2)=sin(3π)=0
- cos(π2y1y2)=cos(π)=−1
D=∣∣
∣∣3216420−11∣∣
∣∣
Using the formula for the determinant of a 3x3 matrix:
D=a(ei−fh)−b(di−fg)+c(dh−eg)
where:
- a=3, b=2, c=1
- d=6, e=4, f=2
- g=0, h=−1, i=1
1. ei−fh=4⋅1−2⋅(−1)=4+2=6
2. di−fg=6⋅1−2⋅0=6−0=6
3. dh−eg=6⋅(−1)−4⋅0=−6−0=−6
D=3⋅6−2⋅6+1⋅(−6)
D=18−12−6=0
The value of the determinant is 0.
Topper's Solved these Questions
- BOOK - ARIHANT MATHS ENGLISHCHAPTER - DETERMINANTS EXERCISE - Exercise (More Than One Correct Option Type Questions)11videos
DETERMINANTS
- BOOK - ARIHANT MATHS ENGLISHCHAPTER - DETERMINANTS EXERCISE - Exercise (Passage Based Questions)21videos
DETERMINANTS
- BOOK - ARIHANT MATHS ENGLISHCHAPTER - DETERMINANTS EXERCISE - Exercise For Session 410videos
DETERMINANTS
- BOOK - ARIHANT MATHS ENGLISHCHAPTER - DEFINITE INTEGRALEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)38videos
DEFINITE INTEGRAL
- BOOK - ARIHANT MATHS ENGLISHCHAPTER - DIFFERENTIAL EQUATION EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)26videos
DIFFERENTIAL EQUATION
Similar Questions
If A(x1,y1),B(x2,y2) and C(x3,y3) are the vertices of a ΔABC and (x, y) be a point on the internal bisector of angle A, then prove that
b∣∣ ∣ ∣∣xy1x1y11x2y21∣∣ ∣ ∣∣+c∣∣ ∣ ∣∣xy1x1y11x3y31∣∣ ∣ ∣∣=0
where, AC = b and AB = c.View Solution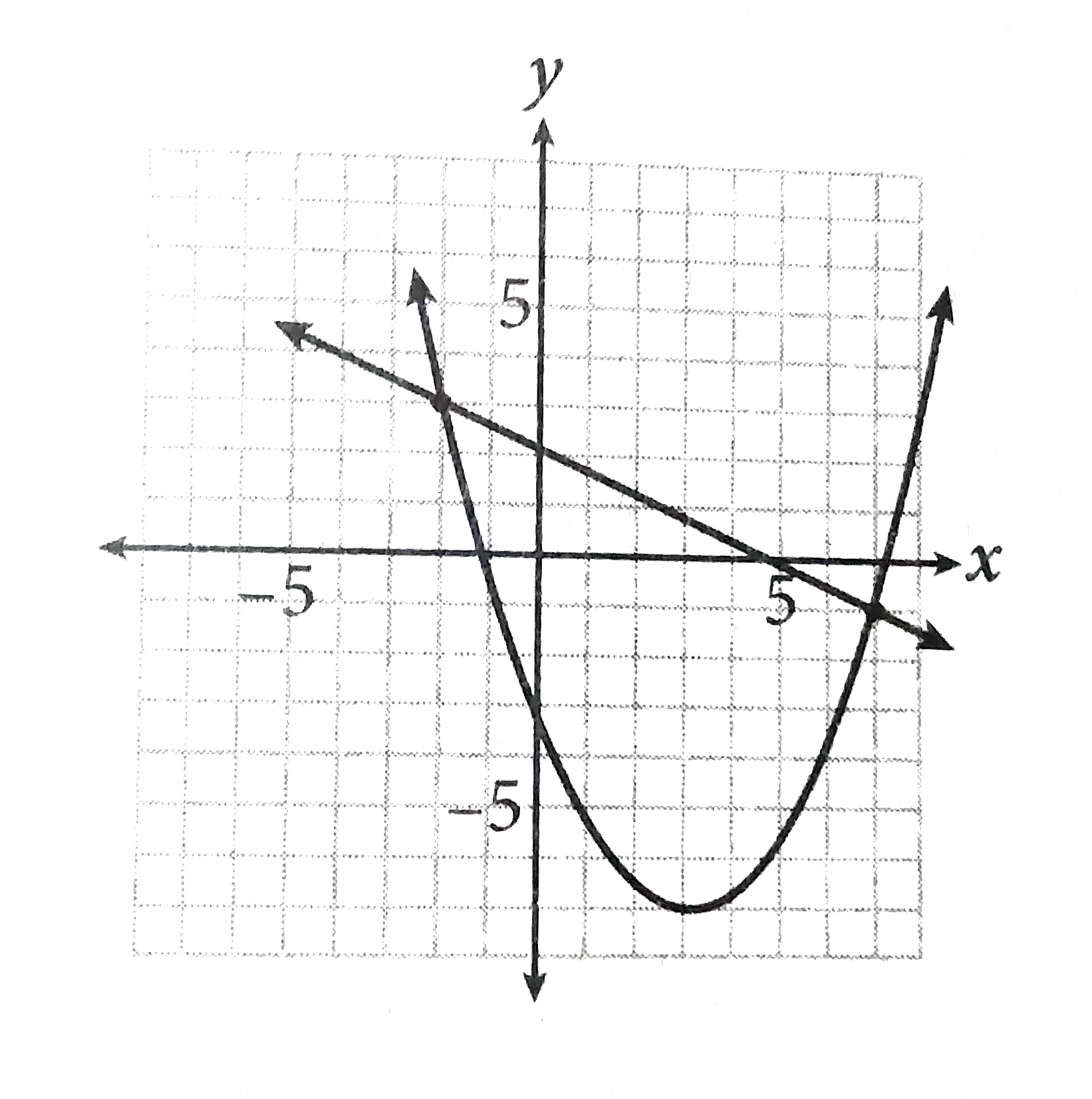
If (x1,y1)and(x2,y2) are solutions to the system of equations shown on the graph, what is the value of x1+x2?View SolutionIf the normals at (x1,y1) and (x2,y2) on y2=4ax meet again on parabola then x1x2+y1y2=
View SolutionIf (x1,y1) and (x2,y2) are ends of focal chord of y2=4ax then x1x2+y1y2 =
View SolutionIf (x1,y1) and (x2,y2) are the ends of a focal chord of the parabola y2 = 4ax then show that x1x2=a2,y1y2=−4a2
View SolutionIf the ordered pairs (x1,y1) and (x2,y2) satisfying the system of equations
log10y−log10|x|=log1004 and log100|x+y|=12, find the value of (x1+x2+y1+y2)View SolutionIf A(x1,y1),B(x2,y2)andC(x3,y3) are vertices of an equilateral triangle whose each side is equal to a, then prove that
∣∣ ∣ ∣∣x1y12x2y22x3y32∣∣ ∣ ∣∣ is equal toView SolutionAn equilateral triangle has each side to a. If the coordinates of its vertices are (x1,y1),(x2,y2) and (x3,y3) then the square of the determinat ∣∣ ∣ ∣∣x1y11x2y21x3y31∣∣ ∣ ∣∣ equals
View SolutionIf (x0,y0,z0)is any solution of the system of equations 2x−y−z=1,−x−y+2z=1andx−2y+z=2, then the value of x20−y20+1z0 (where, z0≠0) is
View SolutionIf the coordinates of the vertices of an equilateral triangle with sides of length a are (x1,y1),(x2,y2) and (x3,y3) then ∣∣ ∣ ∣∣x1y11x2y21x3y31∣∣ ∣ ∣∣2=3a44
View Solution
