img src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/CEN_ALG_DPP_4_2_E01_231_Q01.png" width="80%"gt
Let ABCD is a unit square and each side of the square is divided in the ratio α:(1−α)(0<α<1) . These points are connected to obtain another square. The sides of new square are divided in the ratio α:(1−α) and points are joined to obtain another square. The process is continued idefinitely. Let an denote the length of side and An the area of the nth square
Ifα=13, then the least value of n for which An>110 isSolution in Bengali
4
5
6
7
The correct Answer is:B
(b) 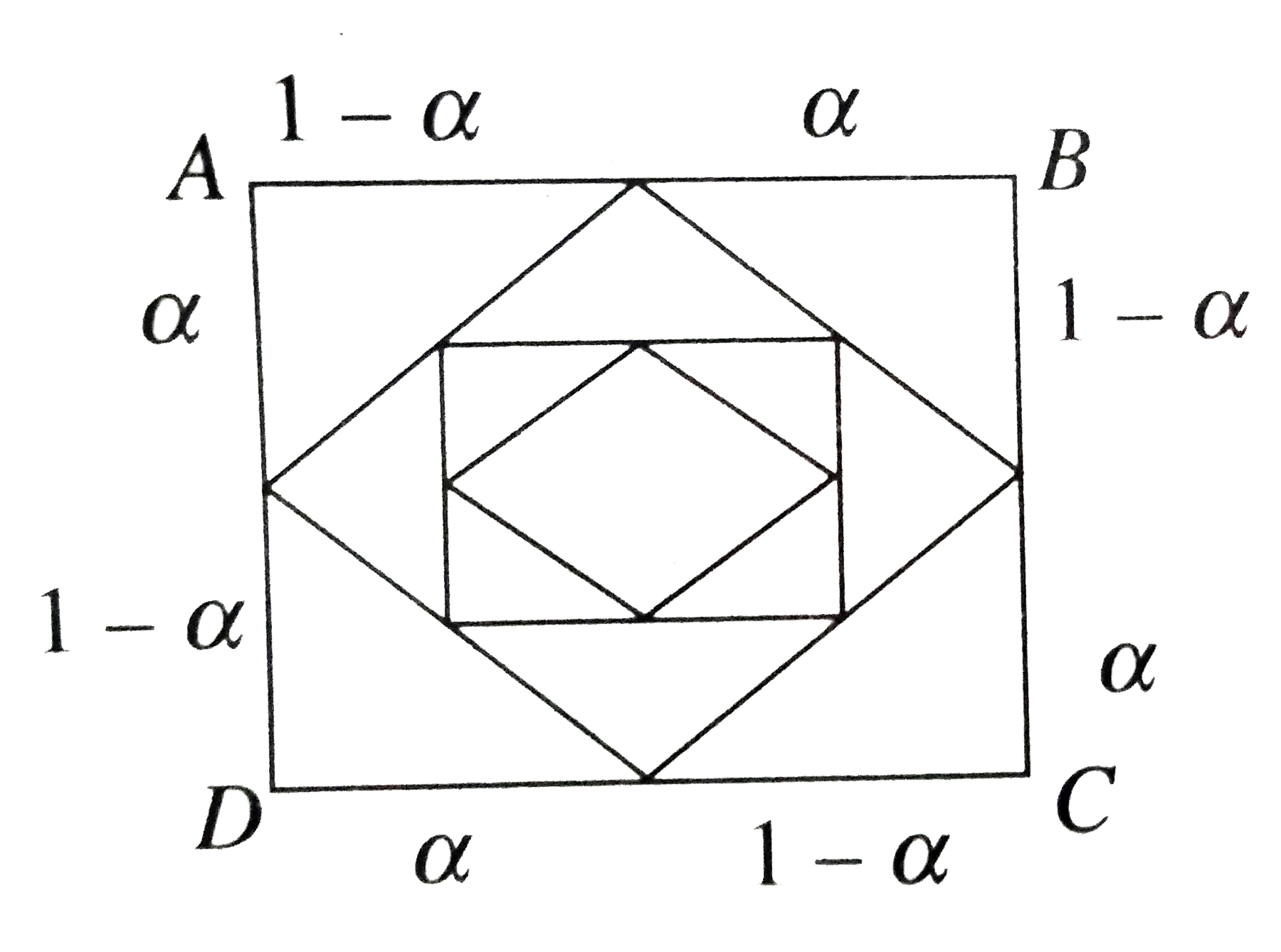
Clearly from the diagram,
a1=1
(an+1)2=[(1−α)2+α2](an)2⇒An+1=[2α2−2α+1]An
For α=13, (an+1)2=59(an)2
⇒An+1An=59
⇒A2=59, A3=(59)2,...
⇒An=(59)n−1
An<110⇒(59)n−1<110
⇒ Least value of n is 5.
∞∑n=1An=83
⇒11−(2α2−2α+1)=83
⇒16α2−16α+3=0
⇒16α2−12α−4α+3=0
⇒(4α−1)(4α−3)=0
⇒α=14, 34
The side of nth square equal to the diagonal of (n+1)th square. ⇒√2an=an+1
⇒(an+1)2(an)2=12
⇒4α2−4α+1=0
⇒α=1/2
Topper's Solved these Questions
- PROGRESSION AND SERIESBOOK - CENGAGE PUBLICATIONCHAPTER - PROGRESSION AND SERIESEXERCISE - Multiple Correct Answer
4 Videos
- PROGRESSION AND SERIESBOOK - CENGAGE PUBLICATIONCHAPTER - PROGRESSION AND SERIESEXERCISE - ILLUSTRATION 5.1
1 Videos
- PROGRESSION AND SERIESBOOK - CENGAGE PUBLICATIONCHAPTER - PROGRESSION AND SERIESEXERCISE - ARCHIVES (NUMERICAL VALUE TYPE )
8 Videos
- PROBABILITY IIBOOK - CENGAGE PUBLICATIONCHAPTER - PROBABILITY IIEXERCISE - MULTIPLE CORRECT ANSWER TYPE
6 Videos
- PROPERTIES AND SOLUTIONS OF TRIANGLEBOOK - CENGAGE PUBLICATIONCHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Archives (Numerical Value Type)
3 Videos
Similar Questions
If a,x,b are in A.P.,a,y,b are in G.P. and a,z,b are in H.P. such that x=9z and a>0, b>0, then (a) y=3z. (b) x=3y (c) 2y= x+z (d) none of these
View SolutionWrite down the sequence whose nth term is 2n/n and (ii) [3+(−1)n]/3n
View SolutionLet Sk,k=1,2,….100 denote the sum of the infinite geometric series whose first term is k−1K! and the common
ration is 1k then the value of (100)2100!+Σ100k=1|(k^2-3k+1)S_k| is ____________
View SolutionLet EandF be two independent events. The probability that exactly one of them occurs is 1125 and the probability if none of them occurring is 225. If P(T) denotes the probability of occurrence of the event T, then (a) P(E)=45,P(F)=35 (b) P(E)=15,P(F)=25 (c) P(E)=25,P(F)=15 (d) P(E)=35,P(F)=45
View SolutionLet ABCandABC' be two non-congruent triangles with sides AB=4,AC=AC′=2√2 and angle B=300 . The absolute value of the difference between the areas of these triangles is
View SolutionDetermine the point of symmetry of a regular hexagon.
View SolutionMatch the following Column A to Column B

View SolutionMatch the following Column A to Column B

View SolutionMatch the following Column A to Column B

View SolutionThe inequation represented by the graph given below is :
View SolutionIf the figure given below ( not to scale), ABCD, CBEF and EGHF are three congruent squares. Find ∠FAE+∠HAG.
View SolutionWhat is the distance OA for the square shown in figure :- ltimg src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/ALN_PHY_R05_E01_015_Q01.png" width="80%"gt
View Solutionimg src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/CEN_ALG_DPP_4_2_E01_231_Q01.png" width="80%"gt
Let ABCD is a unit square and each side of the square is divided in the ratio α:(1−α)(0<α<1) . These points are connected to obtain another square. The sides of new square are divided in the ratio α:(1−α) and points are joined to obtain another square. The process is continued idefinitely. Let an denote the length of side and An the area of the nth square
Ifα=13, then the least value of n for which An>110 is
View Solution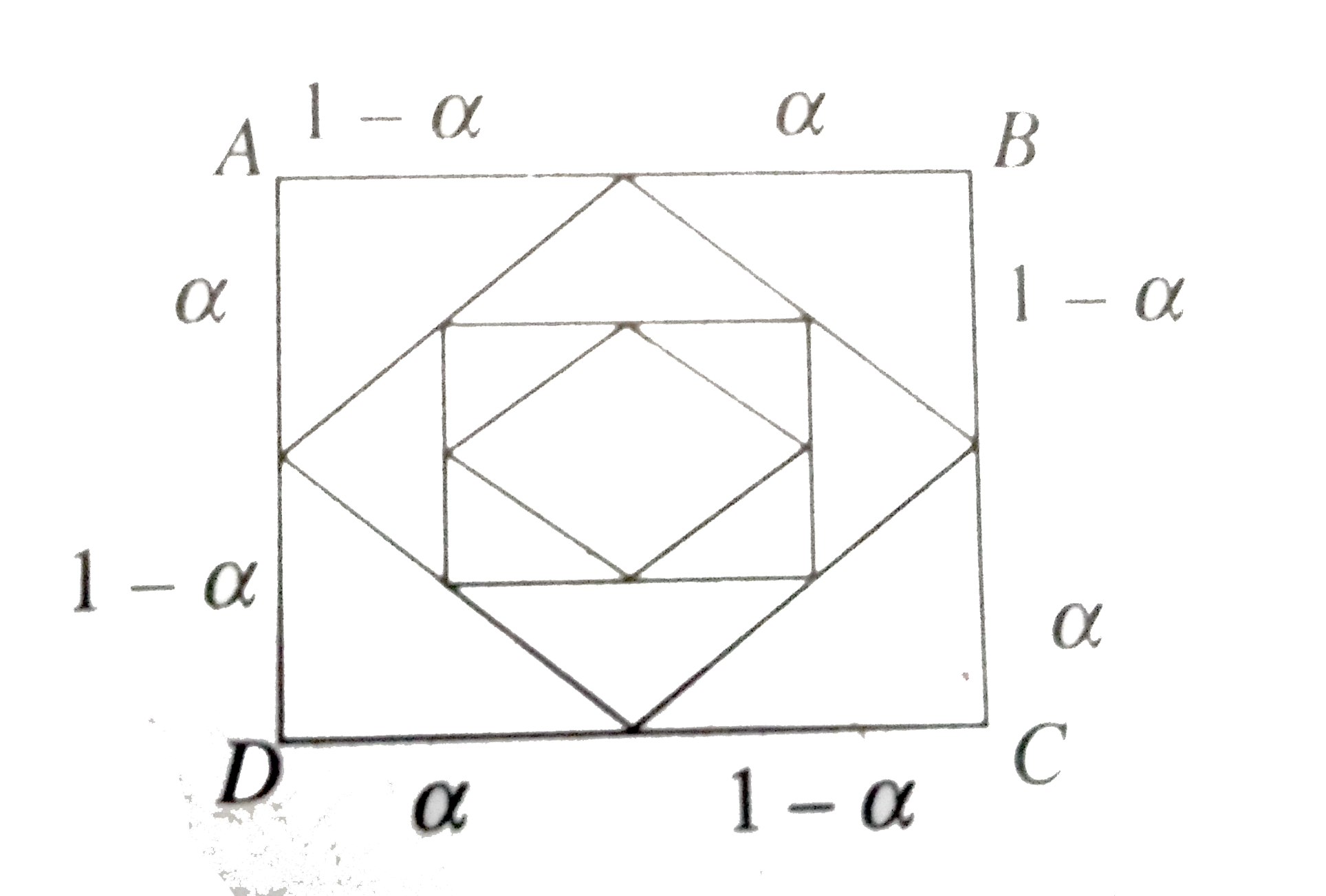
Let ABCD is a unit square and each side of the square is divided in the ratio α:(1−α)(0<α<1) . These points are connected to obtain another square. The sides of new square are divided in the ratio α:(1−α) and points are joined to obtain another square. The process is continued idefinitely. Let an denote the length of side and An the area of the nth square
The value of α for which ∞∑n=1An=83 is/are
View Solution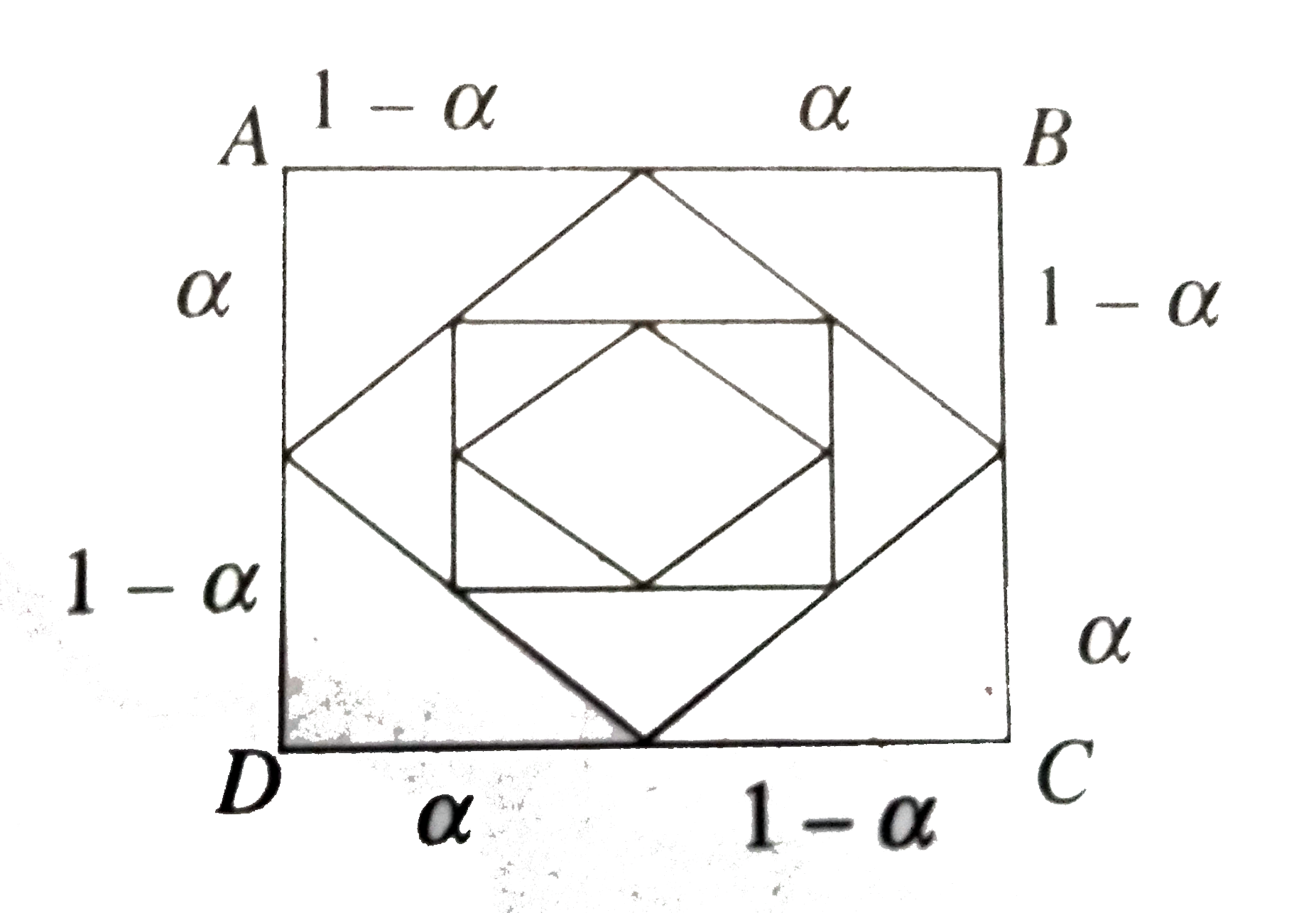 Let ABCD is a unit square and each side of the square is divided in the ratio α:(1−α)(0<α<1) . These points are connected to obtain another square. The sides of new square are divided in the ratio α:(1−α) and points are joined to obtain another square. The process is continued idefinitely. Let an denote the length of side and An the area of the nth square
Let ABCD is a unit square and each side of the square is divided in the ratio α:(1−α)(0<α<1) . These points are connected to obtain another square. The sides of new square are divided in the ratio α:(1−α) and points are joined to obtain another square. The process is continued idefinitely. Let an denote the length of side and An the area of the nth square
The value of α for which side of nth square equal to the diagonal of (n+1)th square is
View Solution
