In a class of 50 students, 35 opted for Mathematics and 37 opted for Biology . How have opted for only Mathematics ? (Assume that each student has to opt for at least one of the subjects )
15
17
13
19
The correct Answer is:C
To solve the problem step by step, we can follow these steps:
Step 1: Understand the problem
We have a total of 50 students in a class. Out of these, 35 students opted for Mathematics and 37 students opted for Biology. We need to find out how many students opted for only Mathematics.
Step 2: Use the formula for the union of sets
We know that the total number of students who opted for at least one subject (Mathematics or Biology) is given by the formula:
n(M∪B)=n(M)+n(B)−n(M∩B)
Where:
- n(M∪B) is the total number of students who opted for at least one subject (50).
- n(M) is the number of students who opted for Mathematics (35).
- n(B) is the number of students who opted for Biology (37).
- n(M∩B) is the number of students who opted for both subjects.
Step 3: Substitute the known values into the formula
Now we can substitute the values into the formula:
50=35+37−n(M∩B)
Step 4: Solve for n(M∩B)
Rearranging the equation gives us:
n(M∩B)=35+37−50
n(M∩B)=72−50
n(M∩B)=22
Step 5: Find the number of students who opted for only Mathematics
To find the number of students who opted for only Mathematics, we use the formula:
n(Only M)=n(M)−n(M∩B)
Substituting the known values:
n(Only M)=35−22
n(Only M)=13
Conclusion
Thus, the number of students who opted for only Mathematics is 13.
We have a total of 50 students in a class. Out of these, 35 students opted for Mathematics and 37 students opted for Biology. We need to find out how many students opted for only Mathematics.
We know that the total number of students who opted for at least one subject (Mathematics or Biology) is given by the formula:
n(M∪B)=n(M)+n(B)−n(M∩B)
Where:
- n(M∪B) is the total number of students who opted for at least one subject (50).
- n(M) is the number of students who opted for Mathematics (35).
- n(B) is the number of students who opted for Biology (37).
- n(M∩B) is the number of students who opted for both subjects.
Now we can substitute the values into the formula:
50=35+37−n(M∩B)
Rearranging the equation gives us:
n(M∩B)=35+37−50
n(M∩B)=72−50
n(M∩B)=22
To find the number of students who opted for only Mathematics, we use the formula:
n(Only M)=n(M)−n(M∩B)
Substituting the known values:
n(Only M)=35−22
n(Only M)=13
Thus, the number of students who opted for only Mathematics is 13.
Topper's Solved these Questions
- SETSBOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - SETSEXERCISE - Self Assessment Sheet
10 Videos
- SETSBOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - SETSEXERCISE - Self Assessment Sheet
10 Videos
- RATIO AND PROPORTION BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - RATIO AND PROPORTION EXERCISE - SELF ASSESSMENT SHEET - 15
10 Videos
- SIMPLE INTEREST BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - SIMPLE INTEREST EXERCISE - Self Assessment Sheet
10 Videos
Similar Questions
In a class of 80 students, 55 students passed in mathematics and 60 passed in chemistry. 10 stu-dents failed in both mathematics and chemistry.
(i) How many students passed in both the subjects ?
(ii) How many students passed in exactly one of the two subjects ?
View Solution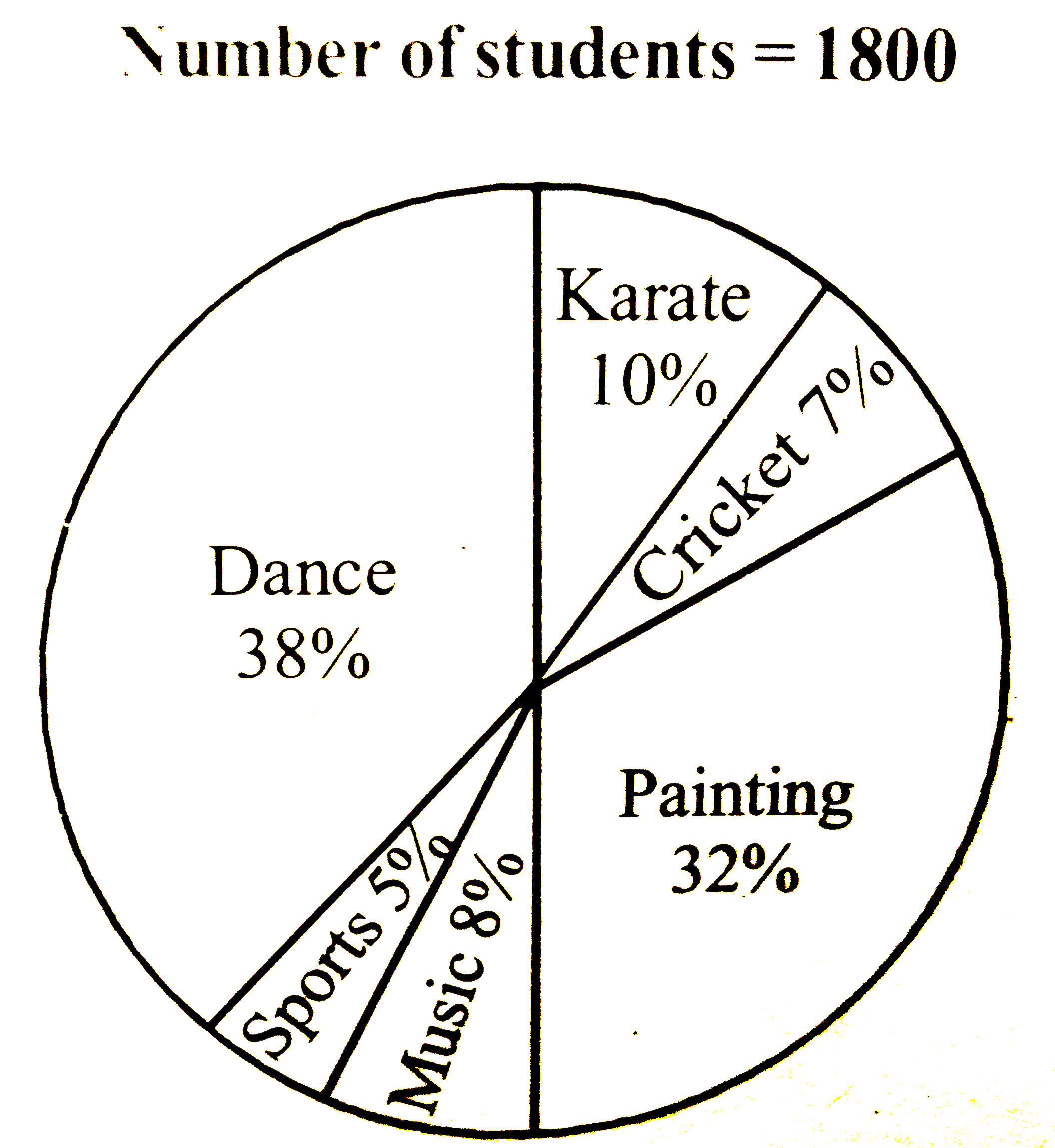
What is the number of students who opted for dancing ?
View Solution
Knowledge Check
Comprehension:
In a class of 25 students of Mathematics and Computer Science, 12 students have taken Mathematics. Out of this, 8 have taken Mathematics but not Computer Science.
What is the number of students who have taken Computer Science but not Mathematics?A13B4C8D12In the group of 240 students, 200 opts for history and 90 opts for Geography. If 20 students do not opt for History as well as Geography, then how many students opt for both the subjects i.e. History and Geography both
A70B40C170Dnone of theseStudy the following graph and answer the questions given below :
No. of students (in thousands) who opted for three different specializations during the given five years in a University

The total number of students who opted for Mathematics in the years 2005 and 2008 together are approximately what percent of the total number of students who opted for all three subjects in the same years ?A38B28C42D32
In a class of 80 students, 55 students passed in mathematics and 60 passed in chemistry. 10 stu-dents failed in both mathematics and chemistry.
(i) How many students passed in both the subjects ?
(ii) How many students passed in exactly one of the two subjects ?
View SolutionIn a class of 35 students , 17 have taken Mathematics , 10 have taken mathematics but not economics if each student has taken either mathematics OR Economics or both , then the number of students who have taken Economics but not mathematics is
View SolutionIn a class of 70 students, 50 students study HL Mathematics and 25 study SL Physics, if each student studies at least one of the two subjects, then number of students studying only SL Physics, is
View SolutionIn a class of 80 students who have appeared in a test in Mathematics and a test in Physics, 55 students have passed in Mathematics and 57 students have passed in Physics, then the number of students who have passed in Physics only is
View SolutionIn a class of 60 students, 40 opted for NCC, 30 opted for NSS and 20 opted for both NCC and NSS. If one of these students is selected at random, then the probability that the student selected has opted neither for NCC nor for NSS is
View Solution
