In a group of 65 people, 40 like cricket, 10 like both cricket and tennis. How many like tennis only and not cricket? How many like tennis?
Let C be the set of people who like cricket and T who like tennis
Here, n(C)=40
∴n(C∪T)=65andn(C∩T)=10
Number of people who like only tennis but not cricket
=n(C∪T)−n(C)
=65−40=25
Again n(C∪T)=n(C)+n(T)−n(C∩T)
⇒65=40+n(T)−10
⇒n(T)=65−40+10=35
and number of people who like tennis = 35.
Topper's Solved these Questions
- SETSBOOK - NAGEEN PRAKASHANCHAPTER - SETSEXERCISE - MISC Exercise
16 Videos
- SETSBOOK - NAGEEN PRAKASHANCHAPTER - SETSEXERCISE - Exercise 1.5
7 Videos
- SEQUENCE AND SERIESBOOK - NAGEEN PRAKASHANCHAPTER - SEQUENCE AND SERIESEXERCISE - Miscellaneous Exercise
32 Videos
- STATISTICSBOOK - NAGEEN PRAKASHANCHAPTER - STATISTICSEXERCISE - MISCELLANEOUS EXERCISE
7 Videos
Similar Questions
In a group of 65 people, 40 like cricket, 10 like both cricket and tennis. How many like tennis only and not cricket? How many like tennis?
View SolutionIn a group of 100 people, 60 people like to play cricket, 20 people like to play both cricket and Tennis. How many people like to play Tennis but not cricket?
View Solution
Knowledge Check
In a group of 60 persons, 40 persons like play cricket and 10 persons like play both Tennis and Cricket. Then, the number of persons who like to play Tennis, is
A20B25C30D40In a group of 60 persons, 40 persons like play cricket and 10 persons like play both Tennis and Cricket. Then, the number of persons who like to play Tennis, is
A20B25C30D40
In a group of 70 people, 37 like coffee, 52 like tea and each person likes at least one of the two drinks. How many people like both coffee and tea?
View SolutionIn a survey of 450 people, it was found that 110 play cricket, 160 play tennis and 70 play both cricket as well as tennis. How many play neither cricket nor tennis?
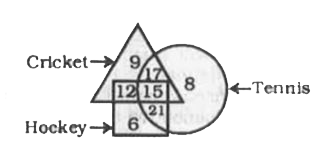
View SolutionIn the given figure, how many people like cricket and tennis both ?
View SolutionIn a group of 50 people , 30 like to play cricket , 25 like to play football and 32 like play hockey . Assume that each one like to play atleast one of the three games . If 15 people like to play both cricket as well as football, 11 people like to play both football well as hockey and 18 like to play both football as as hockey, then
(i) how many like to play all the three games?
(ii) how many like to play only football ?
(iii) how many like to play only hockey ?
View SolutionIn a group of 36 students, 18 like volleyball, 12 like hockey, and 14 like neither of the games. How many like both games?
View SolutionIn a group of 50 persons, 30 like tea, 25 like cofffee and 16 like both, how many like.
(i) either tea or coffee ?
(ii) neither tea nor coffee?
View Solution
