In a hurdle race, a runner has probability p of jumping over a specific hurdle. Given that in 5 trials, the runner succeeded 3 times, the conditional probabilit that the runner had succeeded in the first trial isSolution in Tamil
3/5
2/5
1/5
None of these
The correct Answer is:A
(a) Let A denote the event that the runner succeeds exactly 3 times out of five and B denote the events that the runner suceeds on the first trial.
P(B/A)=P(B∩A)P(A)
But P(B∩A)=P (clearing succeeding in the first trial and exactly once in two other trials)
=4C2p2(1−p)2)=6p3(1−p)2
and P(A)=5C3p3(1−p)2=10p3(1−p)2
Thus, P(B/A)=6p3(1−p)210p3(1−p)2=35
Topper's Solved these Questions
- PROBABILITYBOOK - CENGAGECHAPTER - PROBABILITYEXERCISE - Comprehension
2 Videos
- PROBABILITYBOOK - CENGAGECHAPTER - PROBABILITYEXERCISE - Multiple Correct Answer
13 Videos
- PRINCIPLE OF MATHEMATICAL INDUCTIONBOOK - CENGAGECHAPTER - PRINCIPLE OF MATHEMATICAL INDUCTIONEXERCISE - Exercise
9 Videos
- PROBABILITY AND STATISTICSBOOK - CENGAGECHAPTER - PROBABILITY AND STATISTICSEXERCISE - Question Bank
24 Videos
Similar Questions
A slip of paper is given to a person A who marks it either with a plus sign or a minus sign. The probability of his writing a plus sign is 1/3. A passes the slip to B, who may either leave it alone or change the sign before passing it to C. Next C passes the slip to D after perhaps changing the sign. Finally D passes it to a refere after perhaps changing the sign. B,C,Deachchan≥thesignwithprobability2//3.<br>TheprobabilitytˆtherefereeobservesaplussignontheslipifitisknowntˆA wrote a plus sign is
View SolutionThe probablities of events , A∩B, A, B and A∪B are respectively in A.P. with second term equal to the common difference. Therefore A and B are
View SolutionProve that by using the principle of mathematical induction for all n∈N:
1.3+3.5+5.7+....+(2n−1)(2n+1)=n(4n2+6n−1)3
View SolutionIf two events A and B are such that P(Al)=0.3, P(B)=0.4 and P(A∩Bl)=0.5, then P(BA∪Bl) equals
View Solution150 धावकों द्वारा 110 मीटर की बाधा दौड़ में लिए गये समय (सेकंड में ), नीचे सारणीबद्ध किए गए है :
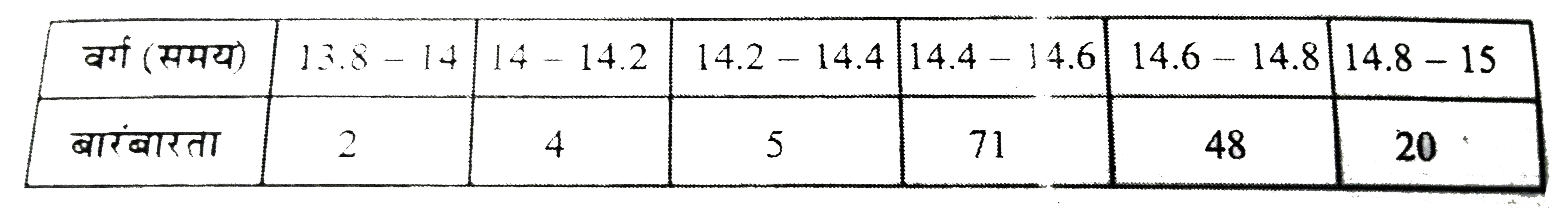
14.6 सेकंड से कम दौड़ समाप्त करने वाले धावकों की संख्या है :
View SolutionEight runners in a race are equally likely to win the race. What is the probability that the race will be won by the runner in lane 1 OR the runner in lane 8?
View Solutionধাবক ও বত্রুধাবক এর পার্থক্য লেখো।
View SolutionTwo runners are running in a race in the same direction at 7 m/s and 5 m/s respectively, the velocity of the first runner with respect to the second will be.
View Solutionएक छात्रा के धावक होने कि प्रायिकता 4/5 है | 5 छात्राओं में से 4 छात्राओं कि धावक होने कि प्रायिकता है :
View SolutionIn a race, the first runner-up finished the race with a faster velocity than the second runner-up and less time. If the acceleration of the first and second place competitors, respectively a_1 Oh a_2 But which relationship is correct?
View SolutionA runner wins a race in front of another runner. The uniform accelerations of them were a1 and a2 respectively . The time taken by the first runner is less by t, and the velocity at the finishing point is higher by v, relative to the second runner. Then
View SolutionIn a hurdle race a player has to cross 10 hurdles . The probability that he will clear each hurdle is 5/6. What is the probability that he will knock down less than 2 hurdles ?
View SolutionIn a hurdle race, a player has to cross 10 hurdles. The probability that he will clear each hurdle is 56. What is the probability that he will knock down fewer than 2 hurdles?
View Solutionধাবক ও বত্রুধাবক এর পার্থক্য লেখো।
View Solutionएक प्रयोग में दो परीक्षण सफल होते हैं फिर एक परीक्षण असफल होता है, तो 6 परीक्षणों में कम से कम 5 परीक्षणों के सफल होने की प्रायिकता है
View Solutionएक अवरोधक दौड़ में एक धावक की एक निश्चित अवरोध को कूदने की प्रायिकता p है। दिया है की 5 प्रयासों में धावक 3 बार सफल होता है धावक के पहले प्रयास में सफल होने की सहप्रतिबन्ध प्रायिकता है
View Solution
