In a parallelogram The bisector of meets in produced meet at Find the length C Foot
It is given that is a parallelogram,
in which and .
the bisector of mets in .
and produce meet at .
From the figure we have ,
therefore ,from and ,we obtain
[side opposite to equal angle are equal]
[,opposite sides of parallelogram]
.
therefore we get required result.
Topper's Solved these Questions
Similar Questions
In a parallelogram ABCD, AB=10cm, AD=6cm. The bisector of ∠A meets DC in E, AE and BC produced meet at F. Find the length C Foot
View SolutionIn a parallelogram ABCD, the bisector of ∠A also bisects BC at X Find AB:AD
View SolutionIn a parallelogram ABCD, the bisector of ∠A also bisects BC at X Prove that AD=2AB
View SolutionIn a parallelogram ABCD diagonals AC and BD intersect at O and AC=6.8 cm and BD=5.6cm . Find the measures of OC and OD
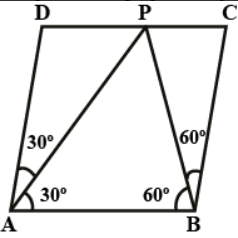
View SolutionIn Figure, ABCD is a parallelogram and ∠DAB=600. If the bisectors AP and BP of angles A and B respectively, meet at P on CD, prove that P is the mid-point of CD
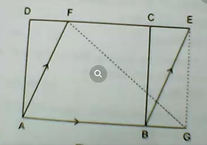
View SolutionIn Figure, ABCD is a rectangle with sides AB=10cmandAD=5cm Find the area of EFG
View SolutionIf ABCD is a rhombus with ∠ABC=56∘, find the measure of ∠ACD
View SolutionABCD is a parallelogram and E is the mid-point of BC, DE and AB when produced meet at F. Then AF= 32AB (b) 2 AB (c) 3 AB (c) 54 AB
View SolutionIn Fig. 21, ABCD is a parallelogram, DL⊥AB. If AB=20 cm , AD=13 cm and area of the parallelogram is 100 cm2 find AL.
View SolutionIn fig. 15 ABCD is a parallelogram CM⊥AB and BL⊥AD. If AB=16 cm, AD=12 cm, CM=10 cm, find BL If AD=10cm, CM=8cm, BL=12 cm, find AB .
View Solution
