In a rhombus of side 10 cm, one of the diagonals is 12 cm long. The length of the second diagonals is
Video Solution
Text Solution
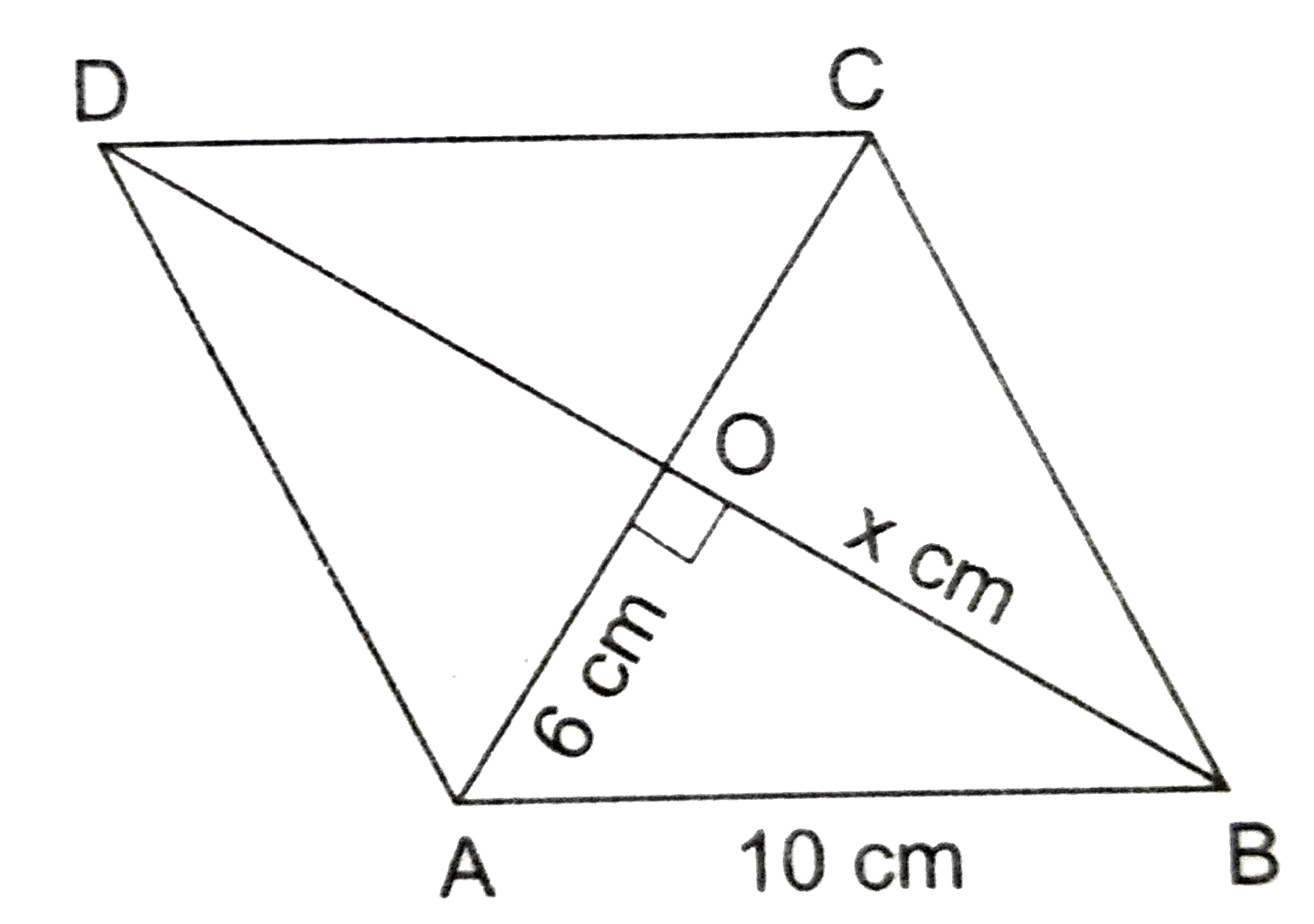
Let ABCD be the given rhomus whose diagonals intersect at O. then, AB=10cm
Let AC=12cmandBD=2xcm.
We know that the diagonals of a rhombus bisect each other at right angles.
∴OA=12AC=6cm,OB=12BD=xcm,and∠AOB=90∘
From right ΔAOB, we have
AB2=OA2+OB2
⇒OB2=AB2−OA2
={(10)2−(6)2}cm2=(100−36)cm2=64cm2
⇒x2=64⇒x=√64=8
∴OB=8cm
∴BD=2×OB=2×8cm=16cm
Hence, the length of the second diagonal is 16cm.
|
Topper's Solved these Questions
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - SOLVED EXAMPLE
4 Videos
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - EXERCISE 7 A
1 Videos
- T-RATIOS OF SOME PARTICULAR ANGLESBOOK - RS AGGARWALCHAPTER - T-RATIOS OF SOME PARTICULAR ANGLESEXERCISE - Exercise 11
27 Videos
- TRIGONOMETRIC IDENTITIESBOOK - RS AGGARWALCHAPTER - TRIGONOMETRIC IDENTITIESEXERCISE - Test Yourself
20 Videos
Similar Questions
Knowledge Check
The length of a side of a rhombus is 10 m and one of its diagonal is 12 m. The length of the other diagonal is :
A15 mB18 mC16 mDcan't be determinedThe perimeter of a rhombus is 40 cm. If one of the diagonals be 12 cm long, what ia the length of the other diagonal ?
A12 cmB√136cmC16 cmD√44cmThe length of a side of a rhombus is 10 m and one of its diagonal is 12 m. The length of the other diagonal is :
A15 mB18 mC16 mDcan't be determined
The side of a rhombus is 13 cm. if one if the diagonals is 24 cm, find the length of the other diagonal.
View SolutionThe length of one side of a rhombus is 13 cm and one of the diagonals is 10 cm. What is the length of the other diagonal?
View SolutionEach side of a rhombus is 10cm. If one of its diagonals is 16cm find the length of the other diagonal.
View SolutionThe area of a rhombus is 36 cm and the length of one of its diagonals is 6 cm. The length of the second diagonal is
View SolutionABCD is a rhombus with side 6 cm. If one of the diagonals is of 3√3 cm then length of other diagonal is
View Solution
