In an arithmetic Progression Tn=3n−1, then common difference is:Solution in Kannada
1
2
3
4
The correct Answer is:C
Step by step video, text & image solution for In an arithmetic Progression T_n=3n-1, then common difference is: by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ARITHMETIC PROGRESSIONSBOOK - OSWAAL PUBLICATIONCHAPTER - ARITHMETIC PROGRESSIONSEXERCISE - VERY SHORT ANSWER TYPE QUESTION
8 Videos
- ARITHMETIC PROGRESSIONSBOOK - OSWAAL PUBLICATIONCHAPTER - ARITHMETIC PROGRESSIONSEXERCISE - SHORT ANSWER TYPE QUESTION
15 Videos
- AREAS RELATED TO CIRCLES BOOK - OSWAAL PUBLICATIONCHAPTER - AREAS RELATED TO CIRCLES EXERCISE - LONG ANSWER TYPE QUESTIONS - I
20 Videos
- CIRCLES BOOK - OSWAAL PUBLICATIONCHAPTER - CIRCLES EXERCISE - TEXTBOOK CORNER (EXERCISE 4.2)
13 Videos
Similar Questions
What is the common difference between the consecutive terms of an A.P.
View SolutionHow many two digit numbers are divisible by 3?
View SolutionIn the given figure, AOB is a sector of angle 60∘ of a circle with centre O and radius 17 cm . If AP ⊥ OB and AP = 15 cm find the area of the shaded region.
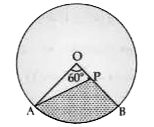
View SolutionFrom a point Q ,the length of the tangent to a circle is 24 cm and the distacne to Q from the centre is 24.5 cm.The radius of the circle is
(a) 4.92 cm
(b)12 cm
©15 cm
(d)24.5 cm
View SolutionIn an arithmetic progression, if S_n = n(5+3n) and t_n = 32, then the value of n is [Note : S_n and t_n denote the sum of first n terms and nth term of arithmetic progression respectively.] (A)4(B)5(C)6(D)7
View Solution(a) The nth term of a progression is (3n+5). Prove that this progression is an arithmetic progression. Also find its 6th term. (b) The nth term of a progression is (3−4n). Prove that this progression is an arithmetic progression. Also find its common difference. (c) The nth term of a progression is (n2−n+1). Prove that it is not an A.P.
View SolutionThe nth term of an arithmetic progression is 3n-1. Find the progression.
View SolutionIf the 10th,15th,25th term of an arithmetic progression are in geometric progression then the common ratio of geometric progression is (common difference of arithmetic progression ≠0) :
View SolutionMake a positive Arithmetic Progression in which the common difference is a small positive quantity.
View SolutionIn an arithmetic progression, if a = 2n +1, then the common difference of the given progression is
View SolutionIn an Arithmetic progression, Tn=10−3n. Find S40
View SolutionFind out which of the following sequences are arithmetic progressions. For those which are arithmetic progressions, find out the common difference. :- 3,6,12,24,….
View SolutionWrite the common difference of Arithmetic Progression (A.P.) : 3, 1, -1,-3,………. .
View SolutionWrite the common difference of Arithmetic Progression (A.P.) : 4, 2, 0,-2,…….. .
View SolutionWrite the common difference of Arithmetic Progression (A.P.) : 2, 0,- 2, - 4, …. .
View Solution
