In Figure, O is the centre of the circle. Find ∠BAC
To solve the problem of finding the angle ∠BAC in the circle where O is the center, we can follow these steps:
1. Identify the Given Angles:
- From the problem, we know:
- ∠AOB=80∘
- ∠AOC=110∘
2. Use the Property of Angles Around a Point:
- The angles around point O (the center of the circle) add up to 360∘. Therefore, we can write:
∠AOB+∠BOC+∠AOC=360∘
3. Substitute the Known Angles:
- Substitute the values of ∠AOB and ∠AOC into the equation:
80∘+∠BOC+110∘=360∘
4. Combine Like Terms:
- Combine 80∘ and 110∘:
190∘+∠BOC=360∘
5. Solve for ∠BOC:
- Rearranging gives:
∠BOC=360∘−190∘=170∘
6. Apply the Inscribed Angle Theorem:
- According to the theorem, the angle subtended by an arc at the center of the circle is twice the angle subtended at any point on the circumference. Therefore:
∠BOC=2×∠BAC
7. Substitute the Value of ∠BOC:
- We know ∠BOC=170∘, so:
170∘=2×∠BAC
8. Solve for ∠BAC:
- Dividing both sides by 2 gives:
∠BAC=170∘2=85∘
9. Conclusion:
- Thus, the measure of angle ∠BAC is:
∠BAC=85∘
- From the problem, we know:
- ∠AOB=80∘
- ∠AOC=110∘
- The angles around point O (the center of the circle) add up to 360∘. Therefore, we can write:
∠AOB+∠BOC+∠AOC=360∘
- Substitute the values of ∠AOB and ∠AOC into the equation:
80∘+∠BOC+110∘=360∘
- Combine 80∘ and 110∘:
190∘+∠BOC=360∘
- Rearranging gives:
∠BOC=360∘−190∘=170∘
- According to the theorem, the angle subtended by an arc at the center of the circle is twice the angle subtended at any point on the circumference. Therefore:
∠BOC=2×∠BAC
- We know ∠BOC=170∘, so:
170∘=2×∠BAC
- Dividing both sides by 2 gives:
∠BAC=170∘2=85∘
- Thus, the measure of angle ∠BAC is:
∠BAC=85∘
Topper's Solved these Questions
Similar Questions
In Figure, O is the centre of the circle. Find ∠CBD
View SolutionIn Figure, O is the centre of the circle and ∠BDC=420. The measure of ∠ACB is (a)420 (b) 480 (c) 580 (d) 520
View SolutionIn Figure, O is the centre of the circle. If ∠BOD=160∘, find the values of x and y
View SolutionIn Figure, O is the centre of the circle. If ∠APB=500, find ∠AOB and ∠OAB
View SolutionIn the adjoining figure, O is the centre of the circle. Find the value of ∠BEC.
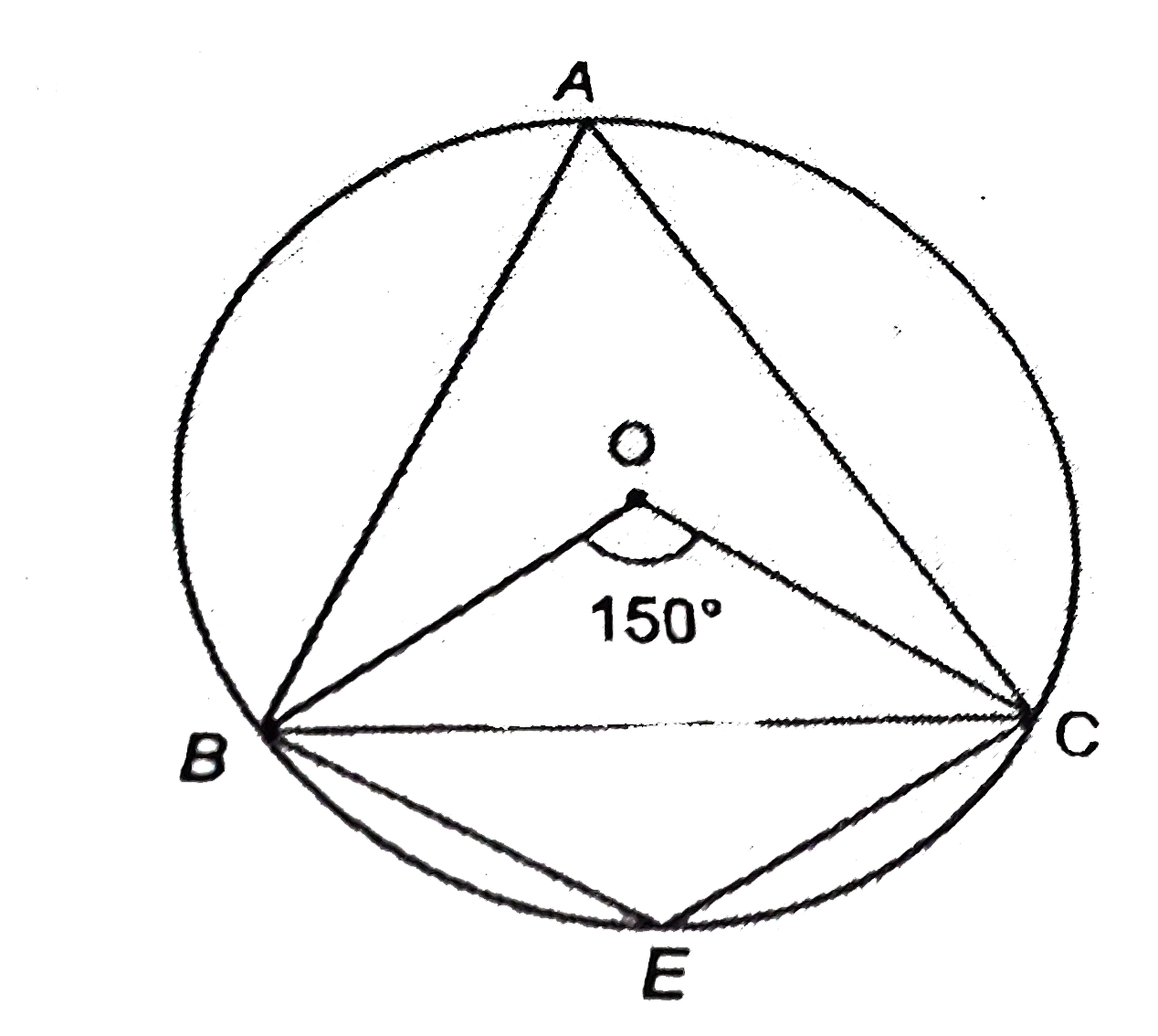
View SolutionIn Figure, O is the centre of the circle. If ∠CEA=300, find the values of x, y and z
View SolutionIn Figure, O is the centre of the circle and BCD is tangent to it at C. Prove that ∠BAC+∠ACD=900 .
View SolutionIn Figure, O is the centre of the circle such that ∠AOC =1300, then ∠ABC= (a)1300 (b) 1150 (c) 650 (d) 1650
View SolutionIn Figure, O is the centre of the circle and the measure of arc ABC is 1000. Determine ∠ADC and ∠ABC
View SolutionIn each of the following figure. O is the centre of the circle . Find the values of a,b c. and d
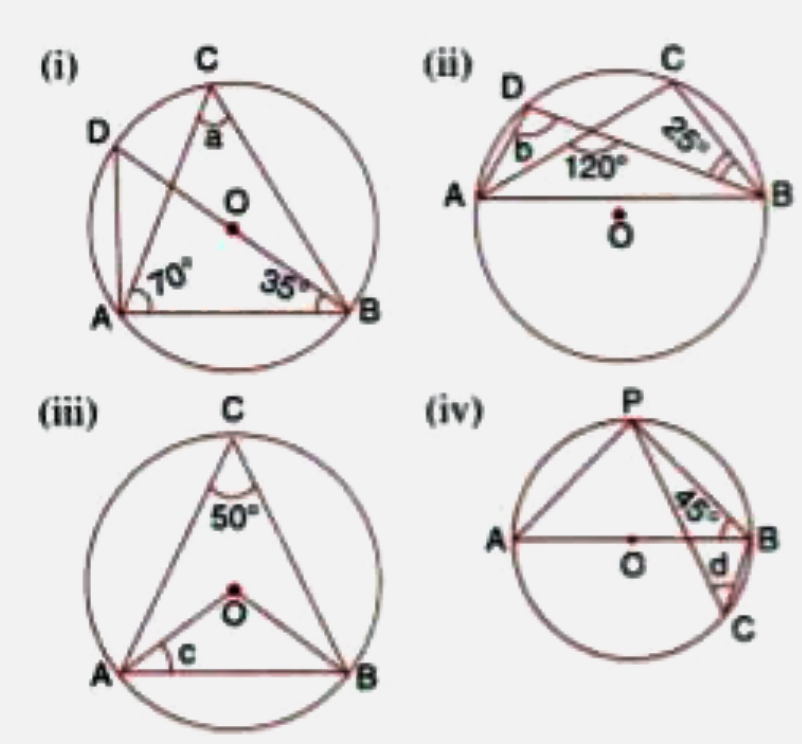
View Solution
