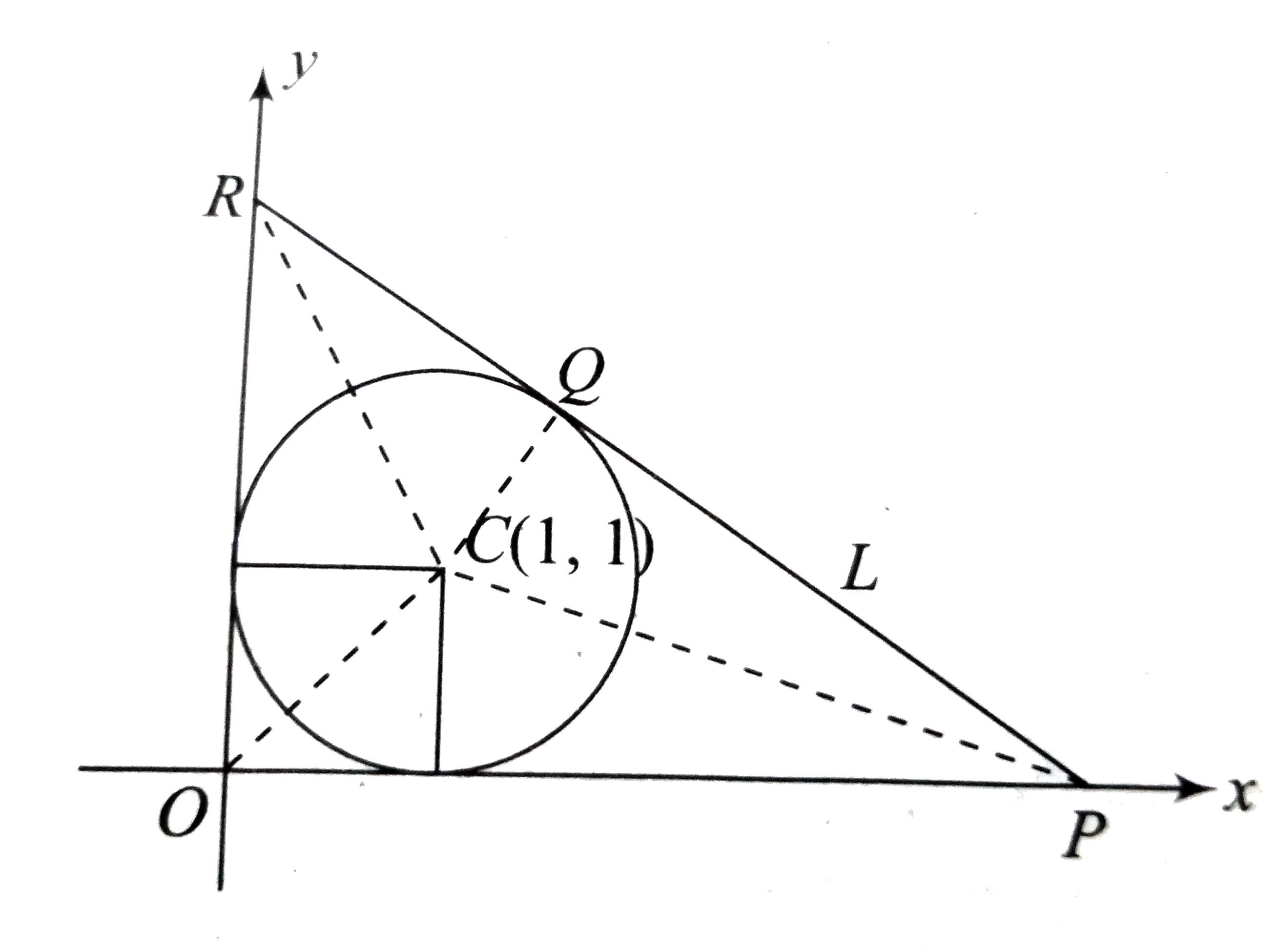
In the diagram as shown, a circle is drawn with centre and radius 1 and a line L. The line L is tangent to the circle at Q. Further L meets the y-axis at R and the x-axis at P in such a way that the angle OPQ equals where .
Equation of the line PR is
The correct Answer is:C
Equation of PR is
or
or
or
Topper's Solved these Questions
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Single Correct Answer Type
58 Videos
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank
32 Videos
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank
32 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - JEE Advanced (Single Correct Answer Type)
14 Videos
- COMPLEX NUMBERSBOOK - CENGAGECHAPTER - COMPLEX NUMBERSEXERCISE - Question Bank
30 Videos
Similar Questions
In the diagram as shown, a circle is drawn with centre C(1, 1) and radius I and a line L. The line Lis tangential to the circle at Q. Further L meet the y-axis at R and the x-axis at Pis such a way that the angle OPQ equals θ where 0<θ<π2 The coordinates of Q are
View SolutionLine 2x+3y+1=0 is a tangent to the circle at (1,-1). This circle is orthogonal to a circle which is drawn having diameter as a line segment with end points (0, -1) and (-2, 3). Then the equation of the circle is
View Solution
Knowledge Check

In the diagram as shown, a circle is drawn with centre C(1,1) and radius 1 and a line L. The line L is tangent to the circle at Q. Further L meets the y-axis at R and the x-axis at P in such a way that the angle OPQ equals θ where 0<θ<π2.
Area of triangle OPR when θ=π/4 isA(3−2√2)B(3+2√2)C(6+4√2)Dnone of theseA tangent to the hyperbola meets x-axis at P and y-axis at Q. Lines PR and QR are drawn such that OPRQ is a rectangle (where O is the origin) then R lies on
A4x2+2y2=1B2x2−4y2=1C2x2+4y2=1D4x2−2y2=1a tangent to the hyperbole X24−y22=1 meets x-axis at P and y-axis at Q. Lines PR and QR are deawn such that OPRQ is a rectangle (where O is the origin). Then R lies on :
A4x2+2y2=1B2x2−4y2=1C2x2+4y2=1D4x2−2y2=1
The line 2x+3y=6,2x+3y−8 cut the X-axis at A,B respectively. A line L=0 drawn through the point (2,2) meets the X-axis at C in such a way that abscissa of A,B,C are in arithmetic Progression. then the equation of the line L is
View SolutionThe area of triangle formed by the lines l1andl2 and the x-axis is:
View SolutionA tangent to the hyperbola x2−2y2=4 meets x-axis at P and y-aixs at Q. Lines PR and QR are drawn such that OPRQ is a rectangle (where O is origin).Find the locus of R.
View SolutionEquation of tangent to the circle x2+y2=50 at the point where the line x+7=0 meets the circle
View SolutionThe lines 2x+3y=6,2x+3y=8 cut the x-axis at A and B A line 'l' is drawn through the point (2,2) meets the x-axis at C in such a way that the abscissa of A, B and C are in geometric progression. The equation of the line 'l' is
View Solution
